Determine the end behavior of the given polynomial function. f ( x ) = x 2 + 4 x + x + 7 x 3 f\left(x\right)=x^2+4x+x+7x^3 f ( x ) = x 2 + 4 x + x + 7 x 3

In this practice problem we want to determine that the end behavior of the given polynomial function, and here I have the function f of x is equal to x squared plus 4x x plus x plus 7x cubed. Now remember, to determine end behavior, we want to look at the very first term when our function is written in standard form. But looking at the polynomial function that I have here, it's actually not written in standard form. So that is the very first thing I wanna do is rewrite this in standard form. Now, remember, we want to have all like terms combined. So here I have this 4 x and this x that can get combined, and then I need it to be in descending order of power, which I can do by moving this 7x cube to the front. So let's go ahead and rewrite this in standard form. I end up with f of x is equal to 7x cubed plus x squared. And then lastly, combining that 4x and that x to give me positive 5x. Now double checking here, I am in standard form now. I'm in descending order of power from 3 to 2 to a power of 1, and everything that can be combined is combined, so we're good. Now we can go ahead and determine our end behavior by looking at that very first term in standard form, which here is 7x3. Now, first, to determine that the behavior of the right side of our function, I know that I need to look at my leading coefficient, which here is 7. Now, this is a positive 7. If I have a positive leading coefficient, I know that that right side is going to rise up, so my right side rises. Now to determine the behavior of the other end, so the ends, remember, I'm looking at the degree here, which which in this case is 3. Now 3 is an odd number, and remember odd opposite. So my n's are going to have the opposite behavior. That's all for this one. Thanks for watching.

Table of contents

Skip topic navigation

0. Fundamental Concepts of Algebra3h 29m
1. Equations and Inequalities3h 27m
2. Graphs1h 43m
3. Functions & Graphs2h 17m
4. Polynomial Functions1h 54m
5. Rational Functions1h 23m
6. Exponential and Logarithmic Functions2h 28m
7. Measuring Angles40m
8. Trigonometric Functions on Right Triangles2h 5m
9. Unit Circle1h 19m
10. Graphing Trigonometric Functions1h 19m
11. Inverse Trigonometric Functions and Basic Trig Equations1h 41m
12. Trigonometric Identities 2h 34m
13. Non-Right Triangles1h 38m
14. Vectors2h 25m
15. Polar Equations2h 5m
16. Parametric Equations1h 6m
17. Graphing Complex Numbers1h 7m
18. Systems of Equations and Matrices3h 6m
19. Conic Sections2h 36m
20. Sequences, Series & Induction1h 15m
21. Combinatorics and Probability1h 45m
22. Limits & Continuity1h 49m
23. Intro to Derivatives & Area Under the Curve2h 9m

4. Polynomial Functions

Understanding Polynomial Functions

Precalculus

4. Polynomial Functions

Understanding Polynomial Functions

Previous problemNext problem

Struggling with Precalculus?

Join thousands of students who trust us to help them ace their exams!Watch the first video

Multiple Choice

Determine the end behavior of the given polynomial function. $f\left(x\right)=x^2+4x+x+7x^3$

Right side rises; Ends are same

Right side rises; Ends are opposite

Right side falls; Ends are same

Right side falls; Ends are opposite

Previous problemNext problem

Verified step by step guidance

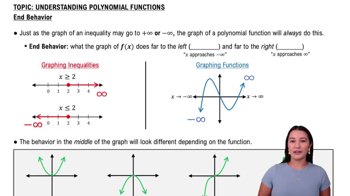
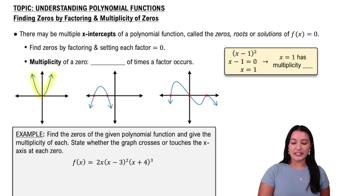
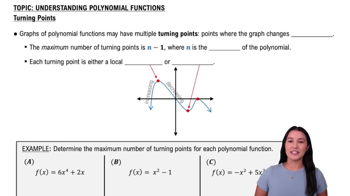
First, simplify the given polynomial function by combining like terms. The function is f(x) = x^2 + 4x + x + 7x^3. Combine the x terms to get f(x) = 7x^3 + x^2 + 5x.

Identify the leading term of the polynomial. The leading term is the term with the highest power of x, which in this case is 7x^3.

Determine the degree of the polynomial. The degree is the highest power of x in the polynomial, which is 3 in this case.

Analyze the leading coefficient and the degree to determine the end behavior. Since the degree is odd (3) and the leading coefficient (7) is positive, the end behavior is such that as x approaches positive infinity, f(x) approaches positive infinity, and as x approaches negative infinity, f(x) approaches negative infinity.

Conclude the end behavior: The right side of the graph rises, and the ends are opposite, meaning one end rises and the other falls.

6:04m

Watch next

Master Introduction to Polynomial Functions with a bite sized video explanation from Patrick

Start learning

Struggling with Precalculus?

Determine the end behavior of the given polynomial function. f(x)=x2+4x+x+7x3f\left(x\right)=x^2+4x+x+7x^3f(x)=x2+4x+x+7x3

Watch next

Determine the end behavior of the given polynomial function. $f\left(x\right)=x^2+4x+x+7x^3$