A uniform, spherical, 1000.0-kg shell has a radius of 5.00 m. (b) Sketch a qualitative graph of the magnitude of the gravitational force this sphere exerts on a point mass m as a function of the distance r of m from the center of the sphere. Include the region from r = 0 to r -> ∞.

Verified step by step guidance

1. Identify the key regions to consider in the graph: inside the sphere (r < 5.00 m) and outside the sphere (r > 5.00 m).
2. Apply the shell theorem for the region inside the sphere (r < 5.00 m). According to the shell theorem, the gravitational force inside a uniform spherical shell of mass is zero. Thus, the graph should start at zero and remain at zero for all values of r less than the radius of the sphere (5.00 m).
3. For the region outside the sphere (r > 5.00 m), use Newton's law of universal gravitation. The force exerted by the sphere can be considered as if all the mass were concentrated at the center. The formula for gravitational force is F = G \frac{Mm}{r^2}, where G is the gravitational constant, M is the mass of the sphere, m is the mass of the point, and r is the distance from the center of the sphere.
4. Plot the gravitational force as inversely proportional to the square of the distance (r^2) for values of r greater than the radius of the sphere (5.00 m). This part of the graph should start at a certain value at r = 5.00 m and decrease asymptotically towards zero as r approaches infinity.
5. Combine these two regions into a single graph: the force is zero from r = 0 to r = 5.00 m, and decreases as 1/r^2 from r = 5.00 m to infinity. This provides a complete sketch of the gravitational force as a function of distance from the center of the sphere.

Key Concepts

Gravitational Force

Gravitational force is the attractive force between two masses, described by Newton's law of universal gravitation. It states that the force is directly proportional to the product of the two masses and inversely proportional to the square of the distance between their centers. This fundamental concept is crucial for understanding how objects interact under gravity.

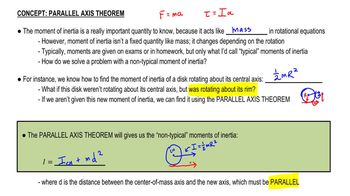
Shell Theorem

The Shell Theorem states that a uniform spherical shell of mass exerts no net gravitational force on any mass located inside it, while outside the shell, it behaves as if all its mass were concentrated at its center. This theorem simplifies the analysis of gravitational forces for spherical objects and is essential for sketching the gravitational force graph in the given scenario.

Graphing Gravitational Force

When graphing the gravitational force exerted by a spherical shell on a point mass, the x-axis typically represents the distance from the center of the shell, while the y-axis represents the magnitude of the gravitational force. The graph will show that the force is zero for distances less than the shell's radius, increases with distance outside the shell, and follows an inverse square law as distance increases, illustrating the behavior of gravitational attraction.