Textbook Question
A 2.00-kg rock has a horizontal velocity of magnitude 12.0 m>s when it is at point P in Fig. E10.35. (a) At this instant, what are the magnitude and direction of its angular momentum relative to point O?
507
views
Ch 10: Dynamics of Rotational Motion
Chapter 10, Problem 10.21c
A solid ball is released from rest and slides down a hillside that slopes downward at 65.0° from the horizontal. (c) In part (a), why did we use the coefficient of static friction and not the coefficient of kinetic friction?
Verified step by step guidance1
Understand the difference between static and kinetic friction: Static friction occurs between two surfaces that are not moving relative to each other, while kinetic friction acts between surfaces in relative motion.
Identify the state of motion of the ball at the beginning: The ball starts from rest, meaning initially there is no relative motion between the ball and the surface of the hill.
Recognize the role of static friction in initiating motion: Static friction must be overcome for the ball to start moving. It acts to prevent the motion as long as the force exerted (due to gravity, in this case) does not exceed the maximum force of static friction.
Determine when kinetic friction would come into play: Once the ball starts moving, the frictional force transitions from static to kinetic. Kinetic friction would then act to oppose the motion of the ball as it slides down.
Conclude why static friction is used in the initial analysis: Since the problem specifically deals with the moment the ball is released and begins to move, static friction is the relevant force resisting the onset of motion, not kinetic friction.
Verified Solution
Video duration:
1m
This video solution was recommended by our tutors as helpful for the problem above.Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Static Friction vs. Kinetic Friction
Static friction is the force that prevents an object from starting to move when a force is applied. It acts on objects at rest and is generally higher than kinetic friction, which applies to objects in motion. In this scenario, the ball is initially at rest, so static friction is relevant until it begins to slide.
Recommended video:
Guided course
04:05
Static & Kinetic Friction
Coefficient of Friction
The coefficient of friction is a dimensionless scalar value that represents the ratio of the force of friction between two bodies and the force pressing them together. The coefficient of static friction is used when an object is not moving, while the coefficient of kinetic friction is used when it is sliding. This distinction is crucial for accurately analyzing the forces acting on the ball.
Recommended video:
Guided course
08:11Static Friction & Equilibrium
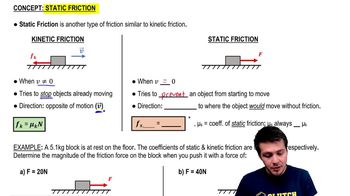
Forces on an Inclined Plane
When an object is on an inclined plane, several forces act on it, including gravitational force, normal force, and frictional force. The angle of the incline affects the components of these forces. Understanding how these forces interact is essential for determining whether the object will remain at rest or begin to slide down the slope.
Recommended video:
Guided course
06:59Intro to Inclined Planes
Related Practice
Textbook Question
The rotor (flywheel) of a toy gyroscope has mass 0.140 kg. Its moment of inertia about its axis is 1.20 * 10^-4 kg•m^2. The mass of the frame is 0.0250 kg. The gyroscope is supported on a single pivot (Fig. E10.51) with its center of mass a horizontal distance of 4.00 cm from the pivot. The gyroscope is precessing in a horizontal plane at the rate of one revolution in 2.20 s. (a) Find the upward force exerted by the pivot.
858
views
Textbook Question
A machine part has the shape of a solid uniform sphere of mass 225 g and diameter 3.00 cm. It is spinning about a frictionless axle through its center, but at one point on its equator it is scraping against metal, resulting in a friction force of 0.0200 N at that point. (b) How long will it take to decrease its rotational speed by 22.5 rad/s?
141
views
Textbook Question
A playground merry-go-round has radius 2.40 m and moment of inertia 2100 kg•m^2 about a vertical axle through its center, and it turns with negligible friction. (a) A child applies an 18.0-N force tangentially to the edge of the merry-go-round for 15.0 s. If the merry-go-round is initially at rest, what is its angular speed after this 15.0-s interval?
181
views
Textbook Question
CP A small block on a frictionless, horizontal surface has a mass of 0.0250 kg. It is attached to a massless cord passing through a hole in the surface (Fig. E10.40). The block is originally revolving at a distance of 0.300 m from the hole with an angular speed of 2.85 rad/s. The cord is then pulled from below, shortening the radius of the circle in which the block revolves to 0.150 m. Model the block as a particle. (a) Is the angular momentum of the block conserved? Why or why not?
140
views
Textbook Question
CP A small block on a frictionless, horizontal surface has a mass of 0.0250 kg. It is attached to a massless cord passing through a hole in the surface (Fig. E10.40). The block is originally revolving at a distance of 0.300 m from the hole with an angular speed of 2.85 rad/s. The cord is then pulled from below, shortening the radius of the circle in which the block revolves to 0.150 m. Model the block as a particle. (b) What is the new angular speed?
159
views