A factory worker pushes a 30.0-kg crate a distance of 4.5 m along a level floor at constant velocity by pushing horizontally on it. The coefficient of kinetic friction between the crate and the floor is 0.25. (e) What is the total work done on the crate?

Verified step by step guidance

1. Calculate the force of friction acting on the crate. Use the formula for frictional force, \( F_{friction} = \mu_k \times F_{normal} \), where \( \mu_k \) is the coefficient of kinetic friction and \( F_{normal} \) is the normal force. Since the crate is moving on a level floor, the normal force is equal to the gravitational force on the crate, which can be calculated using \( F_{normal} = m \times g \), where \( m \) is the mass of the crate and \( g \) is the acceleration due to gravity (approximately 9.8 m/s^2).
2. Determine the force exerted by the worker. Since the crate moves at a constant velocity, the net force on the crate is zero. Therefore, the force exerted by the worker must be equal and opposite to the frictional force.
3. Calculate the work done by the worker using the formula \( W = F \times d \times \cos(\theta) \), where \( W \) is the work done, \( F \) is the force exerted by the worker, \( d \) is the distance over which the force is exerted, and \( \theta \) is the angle between the force and the direction of motion. In this case, the force is exerted horizontally and the crate moves horizontally, so \( \theta = 0 \) degrees.
4. Calculate the work done by the frictional force. It is also given by \( W = F \times d \times \cos(\theta) \). However, the frictional force acts in the opposite direction to the motion, so \( \theta = 180 \) degrees, and \( \cos(180^\circ) = -1 \).
5. Add the work done by the worker and the work done by the friction to find the total work done on the crate. Since the work done by the friction is negative, it will subtract from the work done by the worker.

Key Concepts

Work

Work is defined as the product of the force applied to an object and the distance over which that force is applied, in the direction of the force. Mathematically, it is expressed as W = F × d × cos(θ), where θ is the angle between the force and the direction of motion. In this scenario, since the crate is pushed horizontally and moves in the same direction, the angle θ is 0 degrees, simplifying the calculation to W = F × d.

Friction

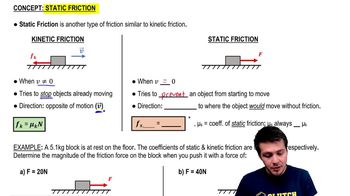
Friction is a force that opposes the relative motion of two surfaces in contact. The coefficient of kinetic friction (μ_k) quantifies this force and is used to calculate the frictional force (F_friction = μ_k × N), where N is the normal force. In this case, the frictional force acts against the worker's push, and understanding this force is crucial for determining the net work done on the crate.

Constant Velocity

When an object moves at constant velocity, it means that its speed and direction remain unchanged over time. According to Newton's first law, if the net force acting on the object is zero, it will maintain its state of motion. In this problem, the worker's applied force must equal the frictional force to keep the crate moving at constant velocity, indicating that the total work done on the crate is zero since there is no net force acting on it.