Textbook Question
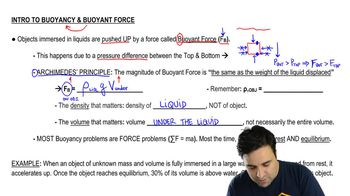
A water tank of height h has a small hole at height y. The water is replenished to keep h from changing. The water squirting from the hole has range 𝓍. The range approaches zero as y → 0 because the water squirts right onto the ground. The range also approaches zero as y → h because the horizontal velocity becomes zero. Thus there must be some height y between 0 and h for which the range is a maximum. (a) Find an algebraic expression for the flow speed v with which the water exits the hole at height y.
771
views