Here are the essential concepts you must grasp in order to answer the question correctly.
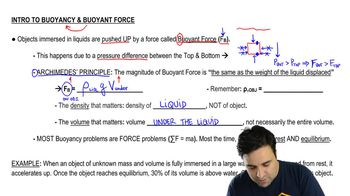
Buoyancy
Buoyancy is the upward force exerted by a fluid on an object submerged in it. This force counteracts the weight of the object, allowing it to float or remain suspended. An object is neutrally buoyant when the buoyant force equals its weight, resulting in no net force acting on it.
Recommended video:
Intro to Buoyancy & Buoyant Force
Density
Density is defined as mass per unit volume and is a key factor in determining whether an object will float or sink in a fluid. For an object to be neutrally buoyant, its density must equal the density of the fluid it is in. The formula for density is ρ = m/V, where ρ is density, m is mass, and V is volume.
Recommended video:
Volume of a Sphere
The volume of a sphere is calculated using the formula V = (4/3)πr³, where r is the radius. This volume is essential for determining the density of the sphere when combined with its mass. In this problem, knowing the sphere's diameter allows us to find its radius and subsequently its volume, which is necessary for calculating the density.
Recommended video: