Here are the essential concepts you must grasp in order to answer the question correctly.
Moment of Inertia
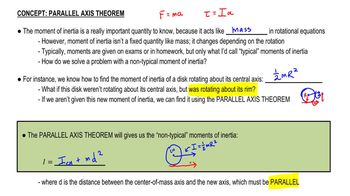
The moment of inertia is a measure of an object's resistance to rotational motion about a specific axis. It depends on the mass distribution relative to that axis, calculated as the sum of the products of each mass and the square of its distance from the axis. For point masses, the formula is I = Σ(m_i * r_i^2), where m_i is the mass and r_i is the distance from the axis.
Recommended video:
Intro to Moment of Inertia
Axis of Rotation
The axis of rotation is an imaginary line around which an object rotates. In this problem, the axis passes through mass A and is perpendicular to the page. The choice of axis significantly affects the moment of inertia, as it determines the distances of the masses from the axis, influencing the overall rotational inertia of the system.
Recommended video:
Mass Distribution
Mass distribution refers to how mass is spread out in a system. In this scenario, the three masses are connected by rigid rods, forming a triangular configuration. Understanding the distances between the masses and their respective weights is crucial for calculating the moment of inertia, as it directly impacts how the system will behave under rotational forces.
Recommended video:
Moment of Inertia & Mass Distribution

 Verified step by step guidance
Verified step by step guidance