Here are the essential concepts you must grasp in order to answer the question correctly.
Newton's Second Law of Motion
Newton's Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. In this scenario, since the safe is sliding at a constant speed, the net force acting on it is zero, indicating that the forces applied by Clyde and Bonnie are balanced by the frictional force.
Recommended video:
Intro to Forces & Newton's Second Law
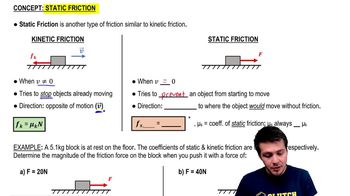
Friction
Friction is the force that opposes the relative motion of two surfaces in contact. The coefficient of kinetic friction is a dimensionless value that represents the ratio of the frictional force resisting the motion of an object to the normal force acting on it. In this case, it helps determine how much force is needed to keep the safe moving at a constant speed.
Recommended video:
Static Friction & Equilibrium
Coefficient of Kinetic Friction
The coefficient of kinetic friction (μk) quantifies the frictional force between two moving surfaces. It is calculated using the formula μk = F_friction / F_normal, where F_friction is the force of friction and F_normal is the normal force. In this problem, the total horizontal force applied by Clyde and Bonnie must equal the frictional force to maintain constant speed, allowing us to solve for μk.
Recommended video:
Kinetic Friction Problems