Here are the essential concepts you must grasp in order to answer the question correctly.
Kinematics
Kinematics is the branch of mechanics that deals with the motion of objects without considering the forces that cause the motion. It involves concepts such as displacement, velocity, and acceleration. In this problem, understanding the initial velocity of the bullet and how it decelerates as it penetrates the wood is crucial for calculating the time taken to come to rest.
Recommended video:
Deceleration
Deceleration refers to the rate at which an object slows down, which is a form of acceleration but in the opposite direction. It is essential to determine how quickly the bullet loses its speed as it penetrates the wood. By applying the equations of motion, we can relate the initial speed, final speed (zero when the bullet stops), and the distance penetrated to find the time taken to come to rest.
Recommended video:
Acceleration with Multiple Parts
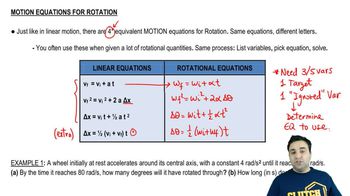
Equations of Motion
The equations of motion describe the relationship between an object's displacement, initial velocity, final velocity, acceleration, and time. These equations are fundamental in solving problems involving linear motion. In this scenario, we can use the second equation of motion, which relates distance, initial velocity, final velocity, and time, to find the time it takes for the bullet to stop after striking the block of wood.
Recommended video:
Equations of Rotational Motion