What is the magnitude of the acceleration of a skydiver at the instant she is falling at one-half her terminal speed?

Verified step by step guidance

1. Understand the problem: The skydiver is falling at half of her terminal speed. At terminal velocity, the net force acting on the skydiver is zero because the gravitational force is balanced by the drag force. At half the terminal speed, the forces are not balanced, so we need to calculate the net force and use Newton's second law to find the acceleration.
2. Express the drag force: The drag force is proportional to the square of the velocity. Mathematically, it can be written as \( F_{\text{drag}} = k v^2 \), where \( k \) is a proportionality constant and \( v \) is the velocity.
3. Relate the drag force at half terminal speed: At terminal velocity \( v_t \), the drag force equals the gravitational force \( F_g = mg \). Therefore, \( k v_t^2 = mg \). At half the terminal speed, the drag force becomes \( F_{\text{drag}} = k (v_t / 2)^2 = \frac{1}{4} k v_t^2 \). Substituting \( k v_t^2 = mg \), we get \( F_{\text{drag}} = \frac{1}{4} mg \).
4. Determine the net force: The net force acting on the skydiver is the difference between the gravitational force and the drag force. Thus, \( F_{\text{net}} = F_g - F_{\text{drag}} = mg - \frac{1}{4} mg = \frac{3}{4} mg \).
5. Apply Newton's second law: Use \( F_{\text{net}} = ma \) to find the acceleration. Substituting \( F_{\text{net}} = \frac{3}{4} mg \), we get \( a = \frac{F_{\text{net}}}{m} = \frac{\frac{3}{4} mg}{m} = \frac{3}{4} g \). The magnitude of the acceleration is \( \frac{3}{4} g \).

Key Concepts

Terminal Velocity

Terminal velocity is the constant speed that a freely falling object eventually reaches when the resistance of the medium through which it is falling prevents further acceleration. For a skydiver, this occurs when the force of gravity is balanced by the drag force due to air resistance, resulting in zero net acceleration.

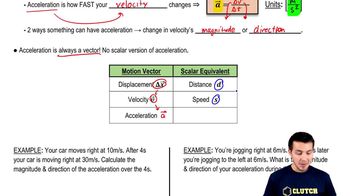
Acceleration

Acceleration is the rate of change of velocity of an object with respect to time. In the context of a skydiver, it is influenced by gravitational force and air resistance. At one-half terminal speed, the skydiver experiences a net force that results in a specific acceleration, which can be calculated using Newton's second law.

Newton's Second Law of Motion

Newton's Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass, expressed as F = ma. This principle is essential for determining the acceleration of the skydiver, as it allows us to calculate the forces acting on her at different speeds, including the effects of gravity and drag.