Pressure Gauge: U-shaped Tube: Videos & Practice Problems

U-shaped tubes, or U-tubes, are essential for measuring pressure differences using height variations of liquids. The fundamental equation is $p=p+\rho gh$. When two liquids of different densities are involved, the heights adjust to maintain equilibrium, leading to the equation $\rho ₁h$_₁=ρ₂h_₂. Understanding these principles is crucial for solving related problems in fluid mechanics.

Pressure Gauges: U-Shaped Tube

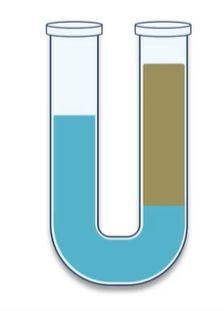
In this discussion, we explore the U-shaped tube, a common type of pressure gauge used to measure pressure differences by utilizing the height of liquid columns. The fundamental principle behind this device is that pressure is determined by the height difference of the liquid columns, which can be expressed using the equation:

\( P_{\text{bottom}} = P_{\text{top}} + \rho g h \)

In a U-shaped tube, both ends can be open to the atmosphere or one end can be closed, creating a vacuum. When both ends are open, the liquid levels will equalize due to atmospheric pressure acting equally on both sides. However, if one side is closed and a vacuum is created, the liquid will rise higher on the open side due to the atmospheric pressure pushing down on it.

When dealing with two different liquids in the U-tube, the situation becomes more complex. The heights of the two liquid columns will not equalize directly because they have different densities. The pressure at the interface of the two liquids must be equal, leading to the relationship:

\( \rho_1 h_1 = \rho_2 h_2 \)

Here, \( \rho_1 \) and \( \rho_2 \) are the densities of the first and second liquids, while \( h_1 \) and \( h_2 \) are their respective heights. This equation is crucial for solving problems involving U-shaped tubes with two different liquids.

To find the difference in height between the two liquid columns, we can use the equation:

\( \Delta h = |h_2 - h_1| \)

In practical applications, such as calculating gauge pressure at the interface of two liquids, the gauge pressure can be determined using the formula:

\( P_{\text{gauge}} = \rho g h \)

Where \( \rho \) is the density of the liquid above the point of interest, \( g \) is the acceleration due to gravity, and \( h \) is the height of the liquid column. For example, if we have a column of oil above water, we would use the density of the oil and its height to calculate the gauge pressure at the water-oil interface.

In summary, understanding the principles of pressure in U-shaped tubes involves recognizing how different liquid densities affect height differences and pressure calculations. The key equations allow for the determination of gauge pressure and height differences, which are essential for solving related physics problems.

Water and oil are poured into a u-shape tube, as shown below. The column of oil, on the right side, is 25 cm tall, and the distance between the top of the two columns is 9 cm. Calculate the density of the oil.

• A. 360 kg/m³
• B. 560 kg/m³
• C. 640 kg/m³
• D. 1562 kg/m³

Answer: (C)

Here’s what students ask on this topic:

What is a U-shaped tube and how is it used to measure pressure?

A U-shaped tube, or U-tube, is a device used to measure pressure differences by observing the height variations of liquids within the tube. It consists of a U-shaped glass tube that can be open on both ends or closed on one end. When a liquid is added, the height of the liquid columns adjusts to balance the pressure. The fundamental equation used is $p=p+\rho gh$, where $\rho$ is the liquid density, $g$ is the acceleration due to gravity, and $h$ is the height difference. This setup allows for the calculation of pressure differences between two points.

How do you calculate the pressure difference in a U-shaped tube with two different liquids?

To calculate the pressure difference in a U-shaped tube with two different liquids, you use the equation $\rho 1_{}h{1}_{}=\rho 2_{}h{2}_{}$. Here, $\rho 1_{}$ and $\rho 2_{}$ are the densities of the two liquids, and $h{1}_{}$ and $h{2}_{}$ are the heights of the liquid columns. This equation ensures that the pressure at the interface of the two liquids is balanced. By knowing the densities and measuring the heights, you can determine the pressure difference between the two sides of the tube.

What is the significance of the height difference in a U-shaped tube?

The height difference in a U-shaped tube is crucial for determining the pressure difference between two points. This difference, denoted as $\mathrm{\Delta h}$, is calculated using the equation $\mathrm{\Delta h}=|h{2}_{}-h{1}_{}|$. The height difference directly relates to the pressure difference via the equation $p=p+\rho gh$. In cases with two different liquids, the height difference helps in balancing the pressures at the interface, ensuring equilibrium. This principle is fundamental in fluid mechanics and is widely used in various applications, including manometers and barometers.

How do you derive the equation ρ₁h₁ = ρ₂h₂ for a U-shaped tube?

To derive the equation $\rho 1_{}h{1}_{}=\rho 2_{}h{2}_{}$ for a U-shaped tube, start with the pressure balance at the interface of the two liquids. The pressure at the bottom of the left column is $p{B}_{}=p{A}_{}+\rho 1_{}gh{1}_{}$, and the pressure at the bottom of the right column is $p{D}_{}=p{C}_{}+\rho 2_{}gh{2}_{}$. Since the pressures at the same height in the same liquid are equal, $p{B}_{}=p{D}_{}$. Setting the right sides of these equations equal and canceling out common terms, you get $\rho 1_{}h{1}_{}=\rho 2_{}h{2}_{}$.

What are the common applications of U-shaped tubes in real life?

U-shaped tubes are commonly used in various applications to measure pressure differences. One of the primary uses is in manometers, which measure the pressure of gases or liquids. They are also used in barometers to measure atmospheric pressure. In laboratory settings, U-tubes help in experiments involving fluid dynamics and pressure measurements. Additionally, they are used in HVAC systems to measure the pressure drop across filters and other components. The simplicity and accuracy of U-shaped tubes make them valuable tools in both educational and industrial contexts.