LR Circuits: Videos & Practice Problems

An LR circuit consists of an inductor (L) and a resistor (R). When a switch closes, current grows, reaching a maximum value of $V/R$ due to the battery's influence. This growth is resisted by the inductor, described by an exponential function. Conversely, when the battery is disconnected, current decays through the resistor, also following an exponential decay pattern. The time constant $\tau =L/R$ determines the rate of these changes, influencing how quickly current stabilizes or dissipates.

LR Circuits

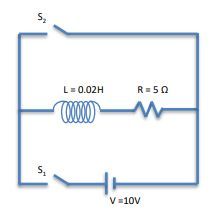
In an LR circuit, which consists of an inductor (L) and a resistor (R), the behavior of current can be categorized into two main processes: current growth and current decay. When a switch (S1) is closed while another switch (S2) remains open, a continuous loop is formed, allowing current to flow from the battery through the inductor and resistor. This phase is characterized by current growth, where the current starts at zero and gradually increases towards its maximum value, determined by Ohm's Law as I_{max} = \frac{V}{R}, where V is the voltage of the battery.

The inductor opposes rapid changes in current due to its property of self-induction, which generates an electromotive force (EMF) that resists the increase in current. The relationship governing this behavior is described by the exponential function I(t) = I_{max} \left(1 - e^{-\frac{t}{\tau}}\right), where \tau = \frac{L}{R} is the time constant of the circuit. As time progresses, the current approaches its maximum value, and after a long time, the exponential term diminishes, indicating that the current stabilizes.

Once the battery is disconnected by opening switch S1 and closing switch S2, the circuit enters the current decay phase. In this scenario, the inductor still resists changes in current, but now the current begins to decrease as it is dissipated through the resistor. The governing equation for this decay is I(t) = I_{max} e^{-\frac{t}{\tau}}. As time increases, the current asymptotically approaches zero, indicating that the energy stored in the inductor is being released through the resistor.

The time constant \tau plays a crucial role in determining the rate of both growth and decay. A smaller time constant, resulting from a lower inductance or higher resistance, leads to faster changes in current, while a larger time constant indicates slower changes. Understanding these principles is essential for analyzing the behavior of LR circuits in various applications.

Unknown Resistance in an LR Circuit

In an LR circuit, when the circuit is initially connected to a battery and then disconnected, the current begins to decay. This decay can be analyzed using the current decay equation, which is essential for understanding the behavior of the circuit after disconnection. The equation is given by:

$$I(t) = \frac{V}{R} e^{-\frac{t}{\tau}}$$

Here, I(t) represents the current at time t, V is the voltage of the battery, R is the resistance, and τ (tau) is the time constant of the circuit. In this scenario, we have:

• Voltage (V): 10 volts
• Time constant (τ): 0.025 seconds
• Time (t): 0.005 seconds
• Current (I) at time t: 0.5 A

To find the resistance R, we can rearrange the current decay equation. By isolating R, we have:

$$R = \frac{V}{I(t) e^{-\frac{t}{\tau}}}$$

Substituting the known values into the equation, we get:

$$R = \frac{10}{0.5 e^{-\frac{0.005}{0.025}}}$$

First, simplify the fraction:

$$R = \frac{10}{0.5} = 20$$

Next, calculate the exponential term:

$$e^{-\frac{0.005}{0.025}} = e^{-0.2}$$

Now, substituting this back into the equation for resistance:

$$R = 20 e^{0.2}$$

Using a calculator, we find that:

$$R \approx 20 \times 0.8187 \approx 16.4 \text{ ohms}$$

Thus, the resistance of the circuit is approximately 16.4 ohms. Understanding this process is crucial for analyzing the behavior of LR circuits during current decay.

Consider the LR circuit shown below. Initially, both switches are open. Switch 1 is closed. a) What is the maximum current in the circuit after a long time? Then, S₁ is opened and S₂ is closed. b) What is the current in the circuit after 0.05s?

• A. (a) i_max=0.5 A; (b) i (0.05s)=1.86×10⁻⁶ A
• B. (a) i_max=2 A; (b) i (0.05s)=7.45×10⁻⁶ A
• C. (a) i_max=0.5 A; (b) i (0.05s)=0.14 A
• D. (a) i_max=2 A; (b) i (0.05s)=0.57 A
• E. (a) i_max=0.5 A; (b) i (0.05s)=0.46 A A
• F. (a) i_max=2 A; (b) i (0.05s)=1.8 A

Answer: (B)

An LR circuit with L = 0.1 H and R = 10 Ω are connected to a battery with the circuit initially broken. When the circuit is closed, how much time passes until the current reaches half of its maximum value?

• A. 6.9×10⁻⁵ s
• B. 6.9×10⁻³ s
• C. 0.69 s
• D. Not enough information to tell

Answer: (B)

Here’s what students ask on this topic:

What is an LR circuit and how does it work?

An LR circuit, also known as an RL circuit, consists of an inductor (L) and a resistor (R) connected in series with a power source. When a switch in the circuit is closed, current begins to flow, and the inductor initially resists changes in current due to its property of self-inductance. This results in an exponential growth of current, described by the equation:

$I=\frac{V}{R}(1-e^{\frac{-}{t}})$

where $\tau =\frac{L}{R}$ is the time constant. When the battery is disconnected, the current decays exponentially through the resistor, following:

$I=I{\max }_e{-}^{\frac{t}{\tau }}$

where $I{\max }_{}$ is the initial current. The time constant $\tau$ determines the rate of current growth and decay.

What is the time constant in an LR circuit and how is it calculated?

The time constant in an LR circuit, denoted as $\tau$, determines the rate at which current grows or decays in the circuit. It is calculated using the formula:

$\tau =\frac{L}{R}$

where $L$ is the inductance of the inductor in henries (H) and $R$ is the resistance of the resistor in ohms (Ω). The time constant represents the time it takes for the current to reach approximately 63% of its maximum value during growth or to decay to about 37% of its initial value during decay. A smaller time constant means faster changes, while a larger time constant indicates slower changes.

How does current growth occur in an LR circuit?

Current growth in an LR circuit occurs when a switch is closed, connecting the circuit to a battery. Initially, the current is zero, but it begins to increase as the battery supplies electrons. The inductor resists this change due to its property of self-inductance, causing the current to grow exponentially rather than instantaneously. The growth of current is described by the equation:

$I=\frac{V}{R}(1-e^{\frac{-}{t}})$

where $V$ is the voltage of the battery, $R$ is the resistance, $t$ is time, and $\tau$ is the time constant $\tau =\frac{L}{R}$. The current eventually reaches a maximum value of $\frac{V}{R}$.

What happens during current decay in an LR circuit?

Current decay in an LR circuit occurs when the battery is disconnected, and the switch is positioned to allow current to flow only through the inductor and resistor. Without the battery, the current begins to decrease as the resistor dissipates the energy stored in the inductor. The inductor resists this decrease, causing the current to decay exponentially. The decay of current is described by the equation:

$I=I{\max }_{}{e}^{\frac{-}{t}}$

where $I{\max }_{}$ is the initial current, $t$ is time, and $\tau$ is the time constant $\tau =\frac{L}{R}$. The current eventually approaches zero as the energy is fully dissipated.

How is the behavior of an LR circuit similar to an RC circuit?

The behavior of an LR circuit is similar to an RC circuit in that both involve exponential growth and decay processes. In an LR circuit, the inductor resists changes in current, leading to exponential current growth and decay, described by:

$I=\frac{V}{R}(1-e^{\frac{-}{t}})$ (growth) and $I=I{\max }_{}{e}^{\frac{-}{t}}$ (decay).

In an RC circuit, a capacitor resists changes in voltage, leading to exponential voltage growth and decay, described by:

$V=\frac{V}{0_{}}(1-e^{\frac{-}{t}})$ (growth) and $V=V{0}_{}{e}^{\frac{-}{t}}$ (decay).

In both circuits, the time constant $\tau$ determines the rate of these changes, with $\tau =\frac{L}{R}$ for LR circuits and $\tau =\mathrm{RC}$ for RC circuits.