Hey, guys. So we saw how when you connect a battery to a capacitor, it becomes a simple circuit. Well, a lot of times, things won't be so simple. You'll have to know how to deal with situations where you have multiple capacitors. All right? So go ahead and check it out in this video.
So basically, the idea is that in circuit problems, you can take and collapse and combine multiple capacitors into what's called a single equivalent capacitor. This is the word that you're going to see a lot. What is the equivalent capacitance? And basically, the idea is that if you have a circuit that's like this, where you have a bunch of capacitors in some random combinations, you can take all of these capacitors with their own individual capacitance. So let's say this is hooked up to, like, a battery like this. Let's make that a little bit better. So if you have a battery that's hooked up to a combination of capacitors, let's say each one of these has their own individual capacitance, so you're going to start labeling these, C_1, C_2, C_3, C_4. You can use some rules to basically simplify this circuit down, so that it turns into and behaves the same way as one, equivalent capacitor hooked up to 1 battery. So that these two things are basically the same, and you have the C_eq here, which is the equivalent capacitance. So all 4 of these capacitors in this arrangement will behave exactly the same way as if you had 1 capacitor hooked up to this one battery.
Okay? So there are a couple of rules that we're going to follow, a couple of situations. The first is when you have a series connection of capacitors, like we have over here with C_1 and C_2. So a series is when you have one capacitor, and then it just follows straight into the next one. So you have all these capacitors like this. And so the way I like to remember it is, like, kind of like how when you're watching Netflix, you're watching a series, you're just binging 1 after the other after the other. So the idea is that these 3 capacitors, each with their own individual capacitance, C_1, C_2, C_3, all behave as if you had a single capacitor like this, which has an equivalent capacitance, C_eq. And I'll write this s here, because we're going to be talking about series connections. So these are direct connections from one to the next, and the equivalent capacitance to find that, we're going to use a rule called inverse sums, which is that the reciprocal of the equivalent capacitance is going to be the sum of the individual reciprocals of capacitors. So one over C_1, C_2, and C_3. And then, of course, if you had more, then you would just keep adding them.
Now what happens, what trips most students up is you'll do all of the stuff in your calculator. You'll do one over C_1, one over C_2, one over C_3, and then you'll forget to take the inverse of that when you're finally done. So you'll get the wrong answer. So you have to remember that this is one over the equivalent capacitance. A lot of the times, like 9 times out of 10, if you screw this up or if a lot of times you'll get answers wrong, it's because you screwed up this step. So add all of the inverse capacitances and then inverse that. So you have to do one over that to get your equivalent capacitance. Okay? So just remember that step right there.
Now, the other kind of connection we have is a parallel connection. So this is where you have a wire, and basically it splits off into a little junction. And you have capacitors that are not following directly one after the other, but you have these capacitors that sort of break off into their own individual loops right here. So you have all these parallel connections right here, because all these capacitors basically are in parallel with each other. So you can see all these parallel lines here. So they're not following directly after one another, they sort of break off into their own loops. So the idea is that this connection right here is the same, and we can collapse this down into the equivalent capacitor C_eq parallel. And so these things split off and form their own individual loops, and to figure out what this equivalent capacitance is, this is actually the easier rule. All you have to do is if you have C_1, C_2, and C_3, you just add them up together. So C_1, C_2, C_3, and then so on and so forth, depending on how many capacitors that you have. Okay? So this is the simpler rule. If they're in parallel, you just add them straight up. If they're in series, then you have to take the inverse sums and then inverse that when you're done with that. Okay? So these are the two rules.
Now sometimes, like I have in this situation right here, we have a circuit with combinations of these things. Some of them are in series, some are in parallel. And to work with, so to figure out how you get to these equivalent capacitances, so we get down to one single equivalent capacitor, you have to work basically from the inside out. So what I mean by that is we have all these capacitors right here. So if I could figure out what the equivalent capacitance of this is, then this would be in series with these other 2. But if you look a little bit more closely, first, I have to do the parallel connection first and then I can do what the series connection is. Okay? So to get a little bit more practice with that, why don't we go ahead and just take a look at some examples.
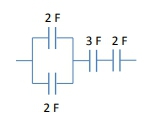
So we've got this first example right here. What's the equivalent capacitance of the following capacitors? So I've got a parallel connection right over here. Right? Because I've got these wires will split off into their own little junctions. So I've got these are in parallel. But if I look a little bit more closely, what I've got is I've got each of the 2 capacitors pairs that are in parallel. Both of these are in series. So I have to work from the inside out. What's the smallest arrangement that I have? I have 2 capacitors in series on the top and the bottom, and then those pairs are in parallel with each other. So the way this is going to work is I want to go from series I want to do all the series ones first, and then I want to move over to the parallel. Okay? So for the series, basically, I have these top and bottom capacitors, and they're going to form an equivalent top and bottom capacitance.
So I've got the rule for summing in series. I have 1ctop=11+13farads. I have to add the inverses of those individual capacitors. Okay? So we've got to remember our rules for adding fractions with unlike denominators. So this one over 1 is the same thing as if I had 3 over 3 plus 1 over 3. So this actually turns out to be 4 over 3, but remember, I'm not done yet, because I have to do the inverse of my, of this fraction right here. So that means that C_top is equal to the inverse of 4 thirds, which is equal to 3 fourths.
Now the other, sort of shortcut that I can give you guys is that if you're ever adding 2 capacitors in series with each other, this only works in series and only works when you're working with 2? Then one shortcut to this equation is you're going to multiply the 2 capacitors, and then you're going to divide by the sum of the 2 capacitors. And we can see how this, this shortcut will give us the exact same 3 fourths. So we have 1 times 3 is 3, and then 1 plus 3 is 4. So this would equal 3 fourths for the top one. You know? So you could use either one of those paths or those equations in order to get what the equivalent capacitance is. Okay? So now, for the bottom one, now we're just going to go ahead and we can use the same process here, or we can use our shortcut equation. So we've got C_1 times C_2. So in other words, 4 times 2 is equal to 8. And now we've got divided by, 4 plus 2, which is 6. So in other words, we've got 3 4 over 3. So now what happens is we have this 4 over 3 and or sorry, this 3 over 4 and this 4 over 3, and those equivalent capacitors are in parallel with each other. So that means that the total equivalent capacitance, that one equivalent capacitor of the entire circuit is just going to be C_top plus C_bottom, because these things are in parallel. So in other words, C_eq is equal to I've got, 3 fourths plus 4 thirds. Now, again, these things are not the same denominator, so I have to make them the same denominator. This is going to be 9 twelfths, and this is going to be, this is going to be 16 twelfths. So if you work this out, you should get 25 over 12. But and yeah. That's it. So actually, this is our answer. So this is actually, our equivalent capacitance in Farads. Okay? So that would be how you deal with these kinds of situations.
Let's do another example. We've got now the equivalent capacitance of this circuit. But if you take a look, what happens is we have these equivalent capacitors are in series with each other. But if you look a little bit more closely, we have each one of those ones in series has a parallel connection. So instead of doing series to parallel, now we actually have to do parallel, and then go into the series. So we have to handle those the up the opposite way. So it all depends on what the innermost arrangement of capacitors that you see is. Now for parallel ones, what I'm a what I have is on the left, so C_left. I have just I would just go ahead and add those 2 things together. So that's just going to be 2 farads plus 2 farads. Because, again, I can just add them straight up because they're just in parallel with each other. So in other words, C_left, which I'll just call C_L, is equal to 4 farads. And just similarly, C_right, I have 1 farad plus 4 farads, is equal to 5 farads. Right? So I've got that C_R.
Now what happens is these 2 capacitors so this basically boils down to I've got a capacitor that is 4 farads, and then plus a capacitor that's 5 farads. And both of these things are in series with each other. So now what I have to do is that the equivalent capacitance is going to be the inverse, so I have to take the inverse of this, and this is going to be 1 over 4 plus 1 over 5. And, again, these things are not the same denominator, so you have to make them. One way to do that is just by multiplying the denominators, if you remember that. So that's just going to be, let's see. 1 over 4 is the same thing as 5 over 20, and then 1 over 5 is the same thing as 4 over 20. So if you add this up together, you're just going to get 9 over 20, but we're not done. The last step is to take the reciprocal of that. So that means that C_eq is just equal to 20 over 9, and that's in farads. So that's how you work with these kinds of problems.
Alright? So let's go ahead and get some more by, like, taking a look at more examples. Let me know in the comments if you have any questions about this.