Hey, guys. In this video, we're going to talk about these really incredible tools that we use when solving AC circuits called phasors. Alright. Let's get to it. Now a phasor is just a rotating vector. Okay. Phasor means phase vector. All the information contained by a phasor is contained in its x component. You can completely ignore the vertical components because it doesn't mean anything. Alright. Phasors are perfect for capturing all the information and representing it very easily for oscillating values like voltage and current, which we know oscillate. For instance, we know that the voltage is a function of time, looks like some maximum voltage times cosine of omega t. This is exactly what I've drawn here. I've given one cycle of voltage that undergoes a sinusoidal oscillation. Okay? And we want to look at how a phasor can easily represent this exact information. Now there are 4 times that I'm going to be interested in. What I'll call time 1, when the voltage is at a maximum and positive, time 2, when the voltage is okay? So these 4 diagrams here, okay? So these 4 diagrams here, sorry, t2, t3, t 4, are going to contain the phasor that represents the information about the voltage at each of those 4 times. These diagrams, by the way, are called phasor diagrams for obvious reasons. Okay? Now, initially, the voltage is at a maximum. In order for a phasor to be at its maximum, its entire length has to be along the x-axis. Okay. This is just because a vector that points along an axis, for instance, the x-axis, that's when that vector's component is largest. Okay? The x component of a vector is largest when that vector points along the x axis. Now the question is which side, left or right, do we want to put it on? By convention, to the right is considered positive and to the left is considered negative. So I'm going to draw the phasor like, negative. So I'm going to draw the phasor like this. It's entirely along the x axis which means that the voltage is at the largest value it could possibly be. Because the phasor, as it rotates, remember a phasor is a rotating vector, is not going to change length. Okay? So this is our voltage phasor. That's at time 1. Now at time 2, the voltage is 0. That means that it has to have no x component. So the phasor has to lie entirely along the vertical axis. The question is, is it up or is it down? These four diagrams that I have marked here, incidentally, are referred to as phasor diagrams for obvious reasons. Okay? Now, initially the voltage is at a maximum. To be at its maximum, a phasor must be fully extended along the x-axis. Okay. This merely indicates that when a vector is aligned with an axis, such as the x-axis, its respective component reaches its maximum value. Now the issue is which side, left or right, should we mount it? By convention, right is deemed positive and left negative. Thus, I will align the phasor like this—it lies entirely on the x-axis, signifying that the voltage has reached the highest possible value. Because the phasor rotates as a rotating vector, its length will not change. Okay? Hence, this represents our voltage phasor at time one. Now at time two, the voltage zeroes out. This necessitates a phasor devoid of any x-component, fully enclosed by the vertical axis. Does it point upwards or downwards? When devoid of any x-components at time two, we conventionally have the phasor pointing upwards. This standard assumes counterclockwise phasor rotation. Okay? At time three, the voltage returns to its maximum but inversely, making it negative. Thus, since it's at its fullest, the phasor should stretch entirely along the x-axis, but pointing leftwards because of its negativity. Okay. And finally, at t4, the voltage zeroes out again. Lacking any x-components, it must reside purely on the vertical axis. Initially directed leftward and rotating counterclockwise, it now faces downwards. And this constitutes the phasor, rotating counterclockwise with the same angular oscillation frequency omega. Simply put, if omega equals 2 per second, it completes two full rotations each second. Okay? Now phasors might seem odd initially as you encounter them. They require practice to be understood fully. So let's engage in an example to aid our familiarity with what a phasor entails. For this upcoming voltage phasor, is the voltage positive or negative? Recall, all relevant data lies along the x-axis. So that's all we focus on: the x-component. This. About as lengthy as this phasor. It's somewhat longer. Regardless. Since it points rightward, we identify this as positive. Okay? Its projection is termed such. It's projection onto the x-axis is positive. Okay? Now the reason phasors prove so invaluable, and why they are so heavily utilized, is that at any particular moment, if you pause time and capture a snapshot, phasors can be manipulated just as vectors are: they can be added, subtracted, and their magnitudes calculated through the Pythagorean theorem, exactly as you would determine a vector's magnitude. Let's demonstrate this with an example. In the subsequent phasor diagram, determine the net phasor's direction for the three displayed phasors. Is the resultant phasor quantity positive or negative? Assume, hypothetically, that these three phasors all describe voltage. Okay? Merely as an illustration—they could just as well delineate current, for example. What I must do is depict this as an entire net voltage phasor. Okay? Here we have two phasors facing identical directions. My apologies, I forgot to label these. V1, v2, and v3. V1 and v3 align along the same axis. Okay? Consequently, the resultant of these two will orient in v3's direction since v3 stretches longer. It resembles two forces aligned contrarily: the stronger force prevails. Thus, we maintain a phasor directed as v3 but slightly diminished in size. Now v2 stands alone because it's orthogonal. Here’s v2, here’s v3 minus v1. I'm uncertain which of these proves longer, v2 or v3 minus v1, but our net phasor will point somewhere in between here. Perhaps in this direction, maybe precisely along the axis, possibly below. In actuality, its precise alignment is irrelevant because it points right regardless. Our value remains invariably positive. Sorry, I shouldn't assert invariably. Our value will be positive. For the net phasor to yield a negative outcome, it would need to point leftward, which it clearly doesn’t. Okay? This only commences our exploration with phasors, gentlemen. Phasors can confuse just as vectors initially bewildered you, but with continual use, you’ll grow increasingly adept with phasors. In upcoming videos, we'll delve more into phasors within the specific contexts of voltage and current in circuits, crystallizing their functionality and application methods. Alright, guys. Thanks for watching.
- 0. Math Review31m
- 1. Intro to Physics Units1h 23m
- 2. 1D Motion / Kinematics3h 56m
- Vectors, Scalars, & Displacement13m
- Average Velocity32m
- Intro to Acceleration7m
- Position-Time Graphs & Velocity26m
- Conceptual Problems with Position-Time Graphs22m
- Velocity-Time Graphs & Acceleration5m
- Calculating Displacement from Velocity-Time Graphs15m
- Conceptual Problems with Velocity-Time Graphs10m
- Calculating Change in Velocity from Acceleration-Time Graphs10m
- Graphing Position, Velocity, and Acceleration Graphs11m
- Kinematics Equations37m
- Vertical Motion and Free Fall19m
- Catch/Overtake Problems23m
- 3. Vectors2h 43m
- Review of Vectors vs. Scalars1m
- Introduction to Vectors7m
- Adding Vectors Graphically22m
- Vector Composition & Decomposition11m
- Adding Vectors by Components13m
- Trig Review24m
- Unit Vectors15m
- Introduction to Dot Product (Scalar Product)12m
- Calculating Dot Product Using Components12m
- Intro to Cross Product (Vector Product)23m
- Calculating Cross Product Using Components17m
- 4. 2D Kinematics1h 42m
- 5. Projectile Motion3h 6m
- 6. Intro to Forces (Dynamics)3h 22m
- 7. Friction, Inclines, Systems2h 44m
- 8. Centripetal Forces & Gravitation7h 26m
- Uniform Circular Motion7m
- Period and Frequency in Uniform Circular Motion20m
- Centripetal Forces15m
- Vertical Centripetal Forces10m
- Flat Curves9m
- Banked Curves10m
- Newton's Law of Gravity30m
- Gravitational Forces in 2D25m
- Acceleration Due to Gravity13m
- Satellite Motion: Intro5m
- Satellite Motion: Speed & Period35m
- Geosynchronous Orbits15m
- Overview of Kepler's Laws5m
- Kepler's First Law11m
- Kepler's Third Law16m
- Kepler's Third Law for Elliptical Orbits15m
- Gravitational Potential Energy21m
- Gravitational Potential Energy for Systems of Masses17m
- Escape Velocity21m
- Energy of Circular Orbits23m
- Energy of Elliptical Orbits36m
- Black Holes16m
- Gravitational Force Inside the Earth13m
- Mass Distribution with Calculus45m
- 9. Work & Energy1h 59m
- 10. Conservation of Energy2h 51m
- Intro to Energy Types3m
- Gravitational Potential Energy10m
- Intro to Conservation of Energy29m
- Energy with Non-Conservative Forces20m
- Springs & Elastic Potential Energy19m
- Solving Projectile Motion Using Energy13m
- Motion Along Curved Paths4m
- Rollercoaster Problems13m
- Pendulum Problems13m
- Energy in Connected Objects (Systems)24m
- Force & Potential Energy18m
- 11. Momentum & Impulse3h 40m
- Intro to Momentum11m
- Intro to Impulse14m
- Impulse with Variable Forces12m
- Intro to Conservation of Momentum17m
- Push-Away Problems19m
- Types of Collisions4m
- Completely Inelastic Collisions28m
- Adding Mass to a Moving System8m
- Collisions & Motion (Momentum & Energy)26m
- Ballistic Pendulum14m
- Collisions with Springs13m
- Elastic Collisions24m
- How to Identify the Type of Collision9m
- Intro to Center of Mass15m
- 12. Rotational Kinematics2h 59m
- 13. Rotational Inertia & Energy7h 4m
- More Conservation of Energy Problems54m
- Conservation of Energy in Rolling Motion45m
- Parallel Axis Theorem13m
- Intro to Moment of Inertia28m
- Moment of Inertia via Integration18m
- Moment of Inertia of Systems23m
- Moment of Inertia & Mass Distribution10m
- Intro to Rotational Kinetic Energy16m
- Energy of Rolling Motion18m
- Types of Motion & Energy24m
- Conservation of Energy with Rotation35m
- Torque with Kinematic Equations56m
- Rotational Dynamics with Two Motions50m
- Rotational Dynamics of Rolling Motion27m
- 14. Torque & Rotational Dynamics2h 5m
- 15. Rotational Equilibrium3h 39m
- 16. Angular Momentum3h 6m
- Opening/Closing Arms on Rotating Stool18m
- Conservation of Angular Momentum46m
- Angular Momentum & Newton's Second Law10m
- Intro to Angular Collisions15m
- Jumping Into/Out of Moving Disc23m
- Spinning on String of Variable Length20m
- Angular Collisions with Linear Motion8m
- Intro to Angular Momentum15m
- Angular Momentum of a Point Mass21m
- Angular Momentum of Objects in Linear Motion7m
- 17. Periodic Motion2h 9m
- 18. Waves & Sound3h 40m
- Intro to Waves11m
- Velocity of Transverse Waves21m
- Velocity of Longitudinal Waves11m
- Wave Functions31m
- Phase Constant14m
- Average Power of Waves on Strings10m
- Wave Intensity19m
- Sound Intensity13m
- Wave Interference8m
- Superposition of Wave Functions3m
- Standing Waves30m
- Standing Wave Functions14m
- Standing Sound Waves12m
- Beats8m
- The Doppler Effect7m
- 19. Fluid Mechanics2h 27m
- 20. Heat and Temperature3h 7m
- Temperature16m
- Linear Thermal Expansion14m
- Volume Thermal Expansion14m
- Moles and Avogadro's Number14m
- Specific Heat & Temperature Changes12m
- Latent Heat & Phase Changes16m
- Intro to Calorimetry21m
- Calorimetry with Temperature and Phase Changes15m
- Advanced Calorimetry: Equilibrium Temperature with Phase Changes9m
- Phase Diagrams, Triple Points and Critical Points6m
- Heat Transfer44m
- 21. Kinetic Theory of Ideal Gases1h 50m
- 22. The First Law of Thermodynamics1h 26m
- 23. The Second Law of Thermodynamics3h 11m
- 24. Electric Force & Field; Gauss' Law3h 42m
- 25. Electric Potential1h 51m
- 26. Capacitors & Dielectrics2h 2m
- 27. Resistors & DC Circuits2h 7m
- 28. Magnetic Fields and Forces2h 23m
- 29. Sources of Magnetic Field2h 30m
- Magnetic Field Produced by Moving Charges10m
- Magnetic Field Produced by Straight Currents27m
- Magnetic Force Between Parallel Currents12m
- Magnetic Force Between Two Moving Charges9m
- Magnetic Field Produced by Loops and Solenoids42m
- Toroidal Solenoids aka Toroids12m
- Biot-Savart Law (Calculus)18m
- Ampere's Law (Calculus)17m
- 30. Induction and Inductance3h 37m
- 31. Alternating Current2h 37m
- Alternating Voltages and Currents18m
- RMS Current and Voltage9m
- Phasors20m
- Resistors in AC Circuits9m
- Phasors for Resistors7m
- Capacitors in AC Circuits16m
- Phasors for Capacitors8m
- Inductors in AC Circuits13m
- Phasors for Inductors7m
- Impedance in AC Circuits18m
- Series LRC Circuits11m
- Resonance in Series LRC Circuits10m
- Power in AC Circuits5m
- 32. Electromagnetic Waves2h 14m
- 33. Geometric Optics2h 57m
- 34. Wave Optics1h 15m
- 35. Special Relativity2h 10m
Phasors - Online Tutor, Practice Problems & Exam Prep
 Created using AI
Created using AIPhasors are rotating vectors that effectively represent oscillating values like voltage and current in AC circuits. The x component of a phasor indicates the voltage's value, while its direction shows whether it's positive or negative. As phasors rotate counterclockwise, they can be analyzed like vectors, allowing for addition and subtraction. Understanding phasors is crucial for solving AC circuit problems, as they simplify the representation of sinusoidal functions, making calculations more manageable. The angular frequency, denoted as ω, determines the rotation speed of the phasor.
Phasors
Video transcript
The following phasor diagram shows an arbitrary phasor during its first rotation. Assuming that it begins with an angle of 0° , if the phasor took 0.027 s to get to its current position, what is the angular frequency of the phasor?
Converting Between a Function and a Phasor
Video transcript
Hey guys, let's do a phasor example. In this case, an example that deals with relating the phasor's equation to the phasor diagram. Okay? The current in an AC circuit is given by this equation. Draw the phasor that corresponds to this current at 15 milliseconds, assuming the phasor begins at 0 degrees. Okay? So in the beginning, the phasor is going to start here at 0 degrees, and it's going to rotate through some amount of angle to arrive at its final position. We want to figure out what that angle is so we know where to draw the final position of this phasor. Remember that the angle is just ω, the angular frequency of the phasor times t. Okay? Now, we know that t is just 15 milliseconds. So what's the angular frequency of the phasor? Well, we're told that the angular frequency of the current is 377 rad/s, and that's going to be the angular frequency of the phasor. However, quickly it's oscillating on a function, right, if I were to draw this as an oscillating graph is going to be the same rate as how quickly it's oscillating on a phasor diagram. Those angular frequencies are the same. So this is just going to be 377 times 15 milliseconds, millis 10 to the negative 3, and this equals 5.66 radians. We want this to be in degrees because it's easiest to graph degrees for us or sorry to draw degrees on a diagram. Remember, you can convert by dividing this by π and multiplying it by 180 degrees. This is going to be 324 degrees. Okay, I'm going to minimize myself and draw this phasor diagram. It started from 0 degrees. Don't forget the phasor began at 0. So starting from 0 and rotating counterclockwise, this phasor ends up in the 4th quadrant because 324 is greater than 270 but less than 360. Okay? There are 2 other ways that you can represent this number if you want. This one is 324 as the full rotation. You can represent it from the negative y-axis if you want, and this would be 54 degrees, or you can represent it from the positive x-axis if you'd like, and this would be 36 degrees. Either way, this is correct, but the important thing to remember is that this value, 324, is how far it traveled from its initial position. It started at 0 degrees and phasors always rotate counterclockwise. So this is 324 degrees. Alright, guys. Thanks for watching.
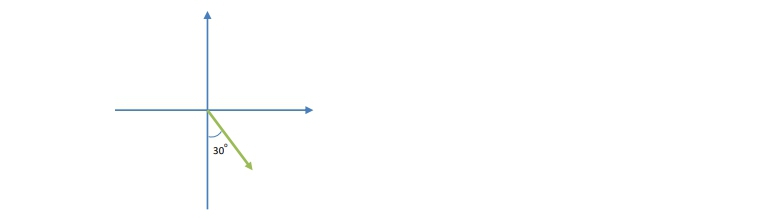
An AC source oscillates with an angular frequency of 120 s-1 . If the initial voltage phasor is shown in the following phasor diagram, draw the voltage phasor after 0.01 s. (Select the correct absolute angle below of the phasor's location below after you have drawn it.)
A phasor of length 4 begins at 0° . If it is rotating at ω = 250 s−1 , what is the value of the phasor after 0.007 s?