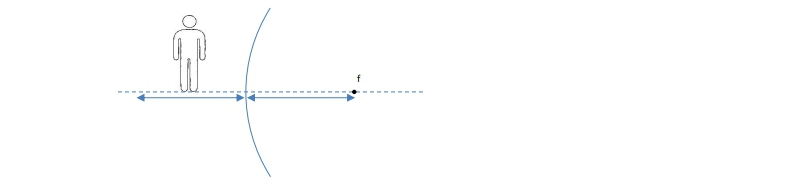
Hey guys, in this video we're going to talk about ray diagrams for concave mirrors. Okay, let's get to it. You can see what happens to light when it reflects off of a mirror by drawing what we refer to as ray diagrams. Ray diagrams are diagrams that simply follow the law of reflection, which we've talked about before, to show the path of these light rays as they reflect off the surface of these mirrors. Okay? Before you can draw any ray diagrams, there's one important point on a ray diagram that you always need to find which is known as the focus. It's the point where initially collimated light converges after reflecting off the mirror. So these blue light rays, this is light coming in from the left that is collimated. Remember, collimated means all of the light rays are parallel. Okay? This collimated light then encounters the surface of this concave mirror, and that causes all of the light to bounce closer towards what we would call the central axis, which goes through the apex of our mirror, sometimes called the vertex. Okay? Now, this light gets reflected towards the central axis and it converges on a point right here which we call the focus, the point where the light is focused. The distance is the focal length and is given by the letter f. Okay? Now that we know what a focus is, when you are given the position of the focus, it's very easy to draw ray diagrams. To draw ray diagrams for concave mirrors, you need to draw 2 of the following lines. Okay? One is a line parallel to the central axis that when reflected off of the mirror passes through the focus. Okay? And that's exactly what we just saw. A line parallel to the central axis by definition is reflected through the focus. If you draw a line through the focus then it's when it's reflected off the mirror it's parallel to the central axis. Okay? This is also just geometry. If I scroll back up really quickly, just like we can follow a line this way and then end up going through the focus, if we follow a line through the focus, we end up reflecting off parallel to the central axis. Okay? It works both ways. Lastly, if you draw a line to the apex of the mirror, it reflects at the same incident angle. And this is just the law of reflection. Right? That if no matter where you are in relation to the mirror you draw the line straight to the apex it bounces off at the same incident angle. That's just the law of reflection. Okay? Let's see this in action. When light comes off an object, in this case I drew a person, a mirror can form an image. This is something that we all know. Right? You can look in a mirror, you can look in the spoon, any reflective surface, and you will see a reflection of yourself. That reflection is an image formed by the mirror. What we want to talk about is how this image is formed by the mirror. Okay? An image by definition is a convergence of light. In order to find where light converges we need to draw 2 lines, 2 of those 3 possible ray diagram lines, and find the point where they intersect. That will be the point where all of the light converges. Okay? So I'm gonna use a protractor because I don't have a ruler and I need to draw these lines straight. So just bear with me because this is gonna take a moment. Alright, so I have a ray coming off the head of the person and is going to be reflected off of the surface of this mirror. This protractor is not working great for the position that it's in so I'm going to scroll up just a little bit. Okay? And it hits the surface. Once it hits the surface it is then reflected through the focus. Okay. That's the first of our three types of rays. Next, I'm just gonna draw the second one which is through the focus to the edge of the mirror. Okay. Straight through the focus to the edge and then parallel to the central axis coming off. Wow. That was weird that it changed at the end. Okay. You can see right here there is a convergence of light. Okay? That convergence of light is going to be an image. What's the image of? The top of the head of this guy that I drew. I want to draw the image of a second part of this guy. I want to do it for his hand. Right? So I'm gonna draw out the first line, which is going to be parallel to the central axis and then through the focus. Alright? And then I'm gonna draw the second ray which is going to be through the focus. And then parallel. Something like that. Okay. This is not exact because I'm literally just using actually used to find angles, rulers, etc. But basically what's happening is right here is the image formed of his hand, and right here is the image formed of his head so we can clearly see that the full image of the person is going to be upside down. In this case, the image is inverted. Okay? The central axis for these ray diagrams, right, this right here, provides that information really easily. If the convergence of the light is below the central axis your image is gonna be inverted. If the convergence of light is above the central axis your image is gonna be upright. That way you don't have to look every time at 2 individual points on an object. You can look at one point and see does the light converge above the central axis or below the central axis. Okay? Let's do an example. Where would an image be formed for an object at the focal point of a concave mirror? So this guy is sitting right on the focal point. We can still draw our same lines parallel to the central axis, then through the focus. Okay. The second line that I'm going to draw is going to be to the apex. Okay? The reason is is because I can't draw through the focus that would be straight down. So I'm gonna do the 3rd line now and I'm gonna draw through, sorry, to the apex. And then when it comes off the apex it's going to come off at the same angle that it entered. Something like this. Okay? Now look, those 2 rays don't converge anywhere in this image. So clearly if they converge it's going to be way way way behind the guy. I want to see if they actually do ever converge. Order to test that I have to compare these two angles. Right? If theta is larger than phi, that means the blue line will be moving towards the red line and they'll converge eventually. But if phi is the bigger angle, that means the red line is always moving away from the blue line and they never converge. So no image will be formed. In order to test this or in order to find the relationship between those angles, I'm gonna draw this as one triangle, And I'm gonna draw this as another triangle. Okay, let me minimize myself for this. First of all, this angle right here is phi, right? That's the whole point of that third line. Whatever the incident angle is that's the same as the reflected angle so this is phi. But notice what's this angle? This is theta. How tall is this triangle? H. What about for the blue triangle? How tall is it? H, however tall the guy is. What's this edge length? Also the focal length. Look at this. These two triangles are identical. So theta equals phi. This means no convergence, no intersection of the light anywhere, which means no image is formed. Okay? So if you have an object on the focal point for a concave mirror, no image will ever be formed because the lines coming off the mirror, the rays coming off the mirror, will always be parallel. These two angles are going to be equal and those rays will always be parallel, so no image. Alright guys. That wraps this up. Thanks for watching.
- 0. Math Review31m
- 1. Intro to Physics Units1h 23m
- 2. 1D Motion / Kinematics3h 56m
- Vectors, Scalars, & Displacement13m
- Average Velocity32m
- Intro to Acceleration7m
- Position-Time Graphs & Velocity26m
- Conceptual Problems with Position-Time Graphs22m
- Velocity-Time Graphs & Acceleration5m
- Calculating Displacement from Velocity-Time Graphs15m
- Conceptual Problems with Velocity-Time Graphs10m
- Calculating Change in Velocity from Acceleration-Time Graphs10m
- Graphing Position, Velocity, and Acceleration Graphs11m
- Kinematics Equations37m
- Vertical Motion and Free Fall19m
- Catch/Overtake Problems23m
- 3. Vectors2h 43m
- Review of Vectors vs. Scalars1m
- Introduction to Vectors7m
- Adding Vectors Graphically22m
- Vector Composition & Decomposition11m
- Adding Vectors by Components13m
- Trig Review24m
- Unit Vectors15m
- Introduction to Dot Product (Scalar Product)12m
- Calculating Dot Product Using Components12m
- Intro to Cross Product (Vector Product)23m
- Calculating Cross Product Using Components17m
- 4. 2D Kinematics1h 42m
- 5. Projectile Motion3h 6m
- 6. Intro to Forces (Dynamics)3h 22m
- 7. Friction, Inclines, Systems2h 44m
- 8. Centripetal Forces & Gravitation7h 26m
- Uniform Circular Motion7m
- Period and Frequency in Uniform Circular Motion20m
- Centripetal Forces15m
- Vertical Centripetal Forces10m
- Flat Curves9m
- Banked Curves10m
- Newton's Law of Gravity30m
- Gravitational Forces in 2D25m
- Acceleration Due to Gravity13m
- Satellite Motion: Intro5m
- Satellite Motion: Speed & Period35m
- Geosynchronous Orbits15m
- Overview of Kepler's Laws5m
- Kepler's First Law11m
- Kepler's Third Law16m
- Kepler's Third Law for Elliptical Orbits15m
- Gravitational Potential Energy21m
- Gravitational Potential Energy for Systems of Masses17m
- Escape Velocity21m
- Energy of Circular Orbits23m
- Energy of Elliptical Orbits36m
- Black Holes16m
- Gravitational Force Inside the Earth13m
- Mass Distribution with Calculus45m
- 9. Work & Energy1h 59m
- 10. Conservation of Energy2h 54m
- Intro to Energy Types3m
- Gravitational Potential Energy10m
- Intro to Conservation of Energy32m
- Energy with Non-Conservative Forces20m
- Springs & Elastic Potential Energy19m
- Solving Projectile Motion Using Energy13m
- Motion Along Curved Paths4m
- Rollercoaster Problems13m
- Pendulum Problems13m
- Energy in Connected Objects (Systems)24m
- Force & Potential Energy18m
- 11. Momentum & Impulse3h 40m
- Intro to Momentum11m
- Intro to Impulse14m
- Impulse with Variable Forces12m
- Intro to Conservation of Momentum17m
- Push-Away Problems19m
- Types of Collisions4m
- Completely Inelastic Collisions28m
- Adding Mass to a Moving System8m
- Collisions & Motion (Momentum & Energy)26m
- Ballistic Pendulum14m
- Collisions with Springs13m
- Elastic Collisions24m
- How to Identify the Type of Collision9m
- Intro to Center of Mass15m
- 12. Rotational Kinematics2h 59m
- 13. Rotational Inertia & Energy7h 4m
- More Conservation of Energy Problems54m
- Conservation of Energy in Rolling Motion45m
- Parallel Axis Theorem13m
- Intro to Moment of Inertia28m
- Moment of Inertia via Integration18m
- Moment of Inertia of Systems23m
- Moment of Inertia & Mass Distribution10m
- Intro to Rotational Kinetic Energy16m
- Energy of Rolling Motion18m
- Types of Motion & Energy24m
- Conservation of Energy with Rotation35m
- Torque with Kinematic Equations56m
- Rotational Dynamics with Two Motions50m
- Rotational Dynamics of Rolling Motion27m
- 14. Torque & Rotational Dynamics2h 5m
- 15. Rotational Equilibrium3h 39m
- 16. Angular Momentum3h 6m
- Opening/Closing Arms on Rotating Stool18m
- Conservation of Angular Momentum46m
- Angular Momentum & Newton's Second Law10m
- Intro to Angular Collisions15m
- Jumping Into/Out of Moving Disc23m
- Spinning on String of Variable Length20m
- Angular Collisions with Linear Motion8m
- Intro to Angular Momentum15m
- Angular Momentum of a Point Mass21m
- Angular Momentum of Objects in Linear Motion7m
- 17. Periodic Motion2h 9m
- 18. Waves & Sound3h 40m
- Intro to Waves11m
- Velocity of Transverse Waves21m
- Velocity of Longitudinal Waves11m
- Wave Functions31m
- Phase Constant14m
- Average Power of Waves on Strings10m
- Wave Intensity19m
- Sound Intensity13m
- Wave Interference8m
- Superposition of Wave Functions3m
- Standing Waves30m
- Standing Wave Functions14m
- Standing Sound Waves12m
- Beats8m
- The Doppler Effect7m
- 19. Fluid Mechanics2h 27m
- 20. Heat and Temperature3h 7m
- Temperature16m
- Linear Thermal Expansion14m
- Volume Thermal Expansion14m
- Moles and Avogadro's Number14m
- Specific Heat & Temperature Changes12m
- Latent Heat & Phase Changes16m
- Intro to Calorimetry21m
- Calorimetry with Temperature and Phase Changes15m
- Advanced Calorimetry: Equilibrium Temperature with Phase Changes9m
- Phase Diagrams, Triple Points and Critical Points6m
- Heat Transfer44m
- 21. Kinetic Theory of Ideal Gases1h 50m
- 22. The First Law of Thermodynamics1h 26m
- 23. The Second Law of Thermodynamics3h 11m
- 24. Electric Force & Field; Gauss' Law3h 42m
- 25. Electric Potential1h 51m
- 26. Capacitors & Dielectrics2h 2m
- 27. Resistors & DC Circuits3h 8m
- 28. Magnetic Fields and Forces2h 23m
- 29. Sources of Magnetic Field2h 30m
- Magnetic Field Produced by Moving Charges10m
- Magnetic Field Produced by Straight Currents27m
- Magnetic Force Between Parallel Currents12m
- Magnetic Force Between Two Moving Charges9m
- Magnetic Field Produced by Loops and Solenoids42m
- Toroidal Solenoids aka Toroids12m
- Biot-Savart Law (Calculus)18m
- Ampere's Law (Calculus)17m
- 30. Induction and Inductance3h 37m
- 31. Alternating Current2h 37m
- Alternating Voltages and Currents18m
- RMS Current and Voltage9m
- Phasors20m
- Resistors in AC Circuits9m
- Phasors for Resistors7m
- Capacitors in AC Circuits16m
- Phasors for Capacitors8m
- Inductors in AC Circuits13m
- Phasors for Inductors7m
- Impedance in AC Circuits18m
- Series LRC Circuits11m
- Resonance in Series LRC Circuits10m
- Power in AC Circuits5m
- 32. Electromagnetic Waves2h 14m
- 33. Geometric Optics2h 57m
- 34. Wave Optics1h 15m
- 35. Special Relativity2h 10m
Ray Diagrams For Mirrors: Study with Video Lessons, Practice Problems & Examples
 Created using AI
Created using AIRay diagrams illustrate how light interacts with different types of mirrors. For concave mirrors, light converges at a focal point, forming real images that can be inverted. In contrast, convex mirrors cause light to diverge, creating virtual images that appear upright and are located behind the mirror. Plane mirrors reflect light without convergence or divergence, producing virtual images of the same height as the object, positioned at an equal distance behind the mirror. Understanding these principles is essential for grasping concepts like focal length and the law of reflection.
Ray Diagrams for Concave Mirrors
Video transcript
Will an image be formed for an object placed inside the focus of a concave mirror? If so, where will it be formed?

The same distance behind the concave mirror.
No image
Yes, at focus
Yes, at the center of curvature.
Ray Diagrams for Convex Mirrors
Video transcript
Guys, in this video, we're going to talk about ray diagrams for convex mirrors. We already saw them for concave mirrors. Now we want to see what they look like for the opposite shaped mirrors. Alright? Let's get to it. While a concave mirror converges light, as we saw when initially collimated light enters a concave mirror, when light reflects off of a convex mirror, it spreads apart. It doesn't come closer together. That means that the light will never focus. Okay? I cannot emphasize this enough. Off of a convex mirror, you will never, ever get convergence of light. It will always be divergent. However, if you were looking at this light, you only see lines; you only see light as traveling in a straight line. So to your brains, this line, this ray, and this ray, all appear to have come from a point where they focused. This is known as an apparent focus. There is an apparent focusing of light or an apparent convergence, and your brain cannot tell the difference. Okay? So when you look at light coming off of this convex mirror, it appears to have focused at this point. Okay? This focus, while it's not real, is often simply referred to as the focus anyway, even though it's technically an apparent focus. And we can define a focal length just like we did before, which is the distance from the apex to the apparent focus. Okay?
Now, to draw ray diagrams for convex mirrors, once again you need to draw two of the three lines. These rules are basically the same but slightly different because the focus is on the other side of the mirror. So a line drawn parallel to the axis is then reflected off of the mirror away from the focus. Okay? A line towards the focus is reflected off of the mirror parallel to the central axis. And, finally, a line to the apex of the mirror is reflected at the same incident angle. So the third line is the same. The first two are very similar but slightly different in how you apply them because the focus is on the opposite side of the mirror. Let's see what I mean. I'm going to minimize myself so we have an unobstructed view of this image. When light comes off of an object, a convex mirror can also form an image, which is what we're about to see. Okay? I cannot stress this enough though. This image is not actually a real image. It's not real because light never converges. Let's see what I mean by this. I'm going to draw the first type of ray which is going to be parallel to the central axis, and then reflected off of the mirror in the direction away from the focus. So you see that green line? The reflected ray follows that green line as if it was leaving the focus. Okay? Now the second line goes towards the focus. Okay? If I were to continue this, it would look like it was going towards the focus. I don't want to have that right now. Okay? But that's what I mean by towards the focus. And then it's reflected off the mirror parallel to the central axis. But notice something. When you see this ray, it appears to have come straight across from the other side of the mirror. Right? So there appears to be a focus. There is an apparent image. This image is not real. This image is not there because the light never actually converges there, but it sure does appear to our brains like it's there. Now I'm going to draw how we always draw images as just being an arrow that points from the central axis to the focus. So this would be upright because it's above the horizontal axis. Okay?
Since this light appears to converge, to our brains this looks identical to any other image. This is known as a virtual image or an apparent image. It's not real. It's just virtual. And in this case, the image is upright. Right? The apparent focus point, the apparent convergence of light occurs above the central axis, so the image is upright. Okay? But these images are not real. They are virtual images. But to our eyes, they appear to be real. Okay? Let's do a quick example. Where would the image be formed for an object a focal length away from the surface of a convex mirror? Okay? So I'm just going to use the first ray diagram. The first ray which is parallel to the central axis. Okay? And then directed away from the focus. Okay? Whoops. Whenever you are drawing these lines, you need to continue them on the other side like this, so you know where the light appears to have intersected. The second one is towards the focus, and then off of the mirror parallel to the central axis. So that's towards the focus, and then off of the mirror parallel to the central axis. Whoops. I need to complete this. So you see, right here, we have an apparent convergence of light which is known as a virtual image. And it will appear inside of the focus of the convex mirror. Okay? That wraps up this discussion on ray diagrams for convex mirrors. Thanks for watching, guys.
Find the location of the virtual image produced by a convex mirror when the object is placed a distance less than the focal length from the surface of the mirror.
Distance less than f behind the mirror
Distance less than f in front of the mirror
A distance greater than f behind the mirror
A distance greater than f in front of the mirror
No image is formed
Ray Diagrams for Plane Mirrors
Video transcript
Hey guys. So far we've discussed convex mirrors and concave mirrors, but now the final type of mirror, plane mirrors. Not plain as in simple, but plane as in flat. These are the types of mirrors that you would hang on your wall. These are your bathroom mirrors, etc. So by far the most popular kind of mirror. Let's get to it. Collimated light coming off of a plane mirror doesn't converge or diverge. The law of reflection states that if you're hitting a flat surface perpendicular to that surface, you bounce off at the same angle. So all the blue rays, those initially collimated rays of light, all bounce off collimated. The light doesn't converge or diverge. That means that there's no focus for a plane mirror, not on the front side of it and not an apparent focus on the back side of it. Sometimes just for equations which we'll cover in the future, just to make those equations work, the focal length of a plane mirror is said to be infinity. It's said that infinitely far away, hypothetically, those lines could converge. It's just a mathematical tool to make equations that we'll see in a little bit work better.
To draw ray diagrams for plane mirrors, we need to draw 2 of the following lines. There are 2 types of axis, then reflected off of the mirror parallel to the axis then reflected off of the mirror parallel to the central axis. And that's exactly what I showed in the image above. If you come in parallel to the central axis, you leave parallel to the central axis. And then any line from anywhere to any points on the mirror is reflected at the same incident angle. For convex and concave mirrors, the second point was only true for lines that went to the apex. But for plane mirrors, because they're flat everywhere, not just at the apex, this applies to any line drawn at any point on the surface.
Let's do an example. A 1.6 meter tall person stands 0.7 meters away from a plane mirror. How tall does the person appear in the mirror? How far from the mirror does the image appear? Is this image real or virtual? So, just a whole bunch of information about the image that they want to know. So let's draw our lines: first, parallel. And this is going to return parallel. So this one is a round trip. It goes both ways. What this also means though is when it's coming back to you, it appears as if it came off the other side of the mirror parallel. Now what I'm going to do next is draw a line from the head to halfway down the body because then it's going to reflect at a 45-degree angle and reach the feet. From the head, I can choose any point on the mirror and it will reflect at the same angle that it hits, but I'm strategically choosing to have it reflect at a point halfway down the person’s body. And so I need to draw where this line appears to come from. And you can see right away there is an apparent convergence of light. So, there is an image here. What type of image? Is it a real image or is it a virtual image? This is absolutely a virtual image. This is not real because the light is not actually converging on that point. It only appears to converge on that point. Furthermore, the only types of mirrors that can produce real images are mirrors that can actually converge light which are concave mirrors. Convex mirrors diverge light so they can never form a real image and plane mirrors don't converge or diverge but since they don't converge, they cannot form a real image either. What's the height of this image? Look at this particular green line. It's at the same height as the person. So the height of the image is just 1.6 meters. Now the question is, how far away is this? Well, this angle is actually going to be the same as this angle. These two triangles are identical triangles. That means that this distance has to be the same. So you're going to find that whenever an object is in front of a plane mirror, that mirror produces a virtual image of the same height as the object, upright, and the same distance behind the mirror that the object exists in front of the mirror. This wraps up our discussion on ray diagrams for plane mirrors. Thanks for watching guys.
You want to hang a plane mirror on your wall. If you want your entire body to fit into the mirror, what's the maximum height off the ground that the mirror must be? What is the smallest mirror you can buy? Consider yourself to be 1.55 m tall.
Height of mirror = 0m; Size of mirror = 1.55m
Height of mirror = 0.775m; Size of mirror = 0.775m
Height of mirror = 0.775m; Size of mirror = 1.55m
Height of mirror = 0.5m; Size of mirror = 0.775m
Do you want more practice?
More setsHere’s what students ask on this topic:
What is the difference between real and virtual images in ray diagrams for mirrors?
Real images are formed when light rays actually converge at a point after reflecting off a mirror. These images can be projected onto a screen and are typically inverted. Concave mirrors can form real images when the object is placed beyond the focal point. Virtual images, on the other hand, are formed when light rays appear to diverge from a point behind the mirror. These images cannot be projected onto a screen and are always upright. Convex mirrors and plane mirrors always form virtual images. Understanding the nature of these images is crucial for applications in optics and imaging technologies.
 Created using AI
Created using AIHow do you draw ray diagrams for concave mirrors?
To draw ray diagrams for concave mirrors, follow these steps: First, draw a line parallel to the central axis from the object to the mirror. This ray will reflect through the focal point. Second, draw a line from the object through the focal point to the mirror. This ray will reflect parallel to the central axis. Lastly, you can draw a line from the object to the apex of the mirror, which will reflect at the same incident angle. The point where these reflected rays converge is where the image is formed. This method helps visualize how concave mirrors focus light to form images.
 Created using AI
Created using AIWhy do convex mirrors only form virtual images?
Convex mirrors only form virtual images because they cause light rays to diverge after reflection. When parallel light rays hit a convex mirror, they spread apart as if they are emanating from a focal point behind the mirror. Since the reflected rays never actually converge, the image formed is virtual. This virtual image appears upright and smaller than the actual object, making convex mirrors useful for applications like vehicle side mirrors, where a wider field of view is beneficial.
 Created using AI
Created using AIHow do plane mirrors form images?
Plane mirrors form images by reflecting light according to the law of reflection, where the angle of incidence equals the angle of reflection. The reflected rays appear to come from a point behind the mirror, creating a virtual image. This image is the same size as the object and is located at the same distance behind the mirror as the object is in front of it. Plane mirrors do not converge or diverge light, so the image is always virtual and upright. This principle is why plane mirrors are commonly used in everyday applications like bathroom mirrors.
 Created using AI
Created using AIWhat is the focal length of a concave mirror, and how is it determined?
The focal length of a concave mirror is the distance from the mirror's surface to its focal point, where parallel light rays converge after reflection. It is denoted by the letter f. The focal length can be determined using the mirror equation: , where do is the object distance and di is the image distance. For a concave mirror, the focal length is positive, and it helps in determining the position and nature of the image formed.
 Created using AI
Created using AI