Here are the essential concepts you must grasp in order to answer the question correctly.
Definition of a Vector
A vector is a mathematical object that has both magnitude and direction. It is often represented graphically as an arrow, where the length indicates the magnitude and the arrowhead shows the direction. Vectors are fundamental in physics and engineering, as they can represent quantities such as force, velocity, and displacement.
Recommended video:
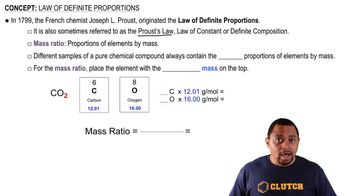
Law of Definite Proportions
Vector Operations
Vectors can be added, subtracted, and multiplied by scalars, which allows for various operations that are essential in solving problems. The addition of vectors involves combining their magnitudes and directions, while scalar multiplication changes the magnitude without altering the direction. Understanding these operations is crucial for applying vectors in real-world scenarios.
Recommended video:
Addition and Subtraction Operations
Applications of Vectors
Vectors are widely used in various fields, including physics, engineering, and computer graphics. They help in modeling physical phenomena, such as motion and forces, and are essential in navigation and robotics. By understanding the function of vectors, one can analyze and predict the behavior of dynamic systems effectively.
Recommended video:
The Ideal Gas Law Applications
 Verified step by step guidance
Verified step by step guidance