Write the nuclear equation for the fusion of H-3 with H-1 to form He-4.
A typical home uses approximately 1.0⨉103 kWh of energy per month. If the energy came from a nuclear reaction, what mass would have to be converted to energy per year to meet the energy needs of the home?
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Energy-Mass Equivalence

Nuclear Energy
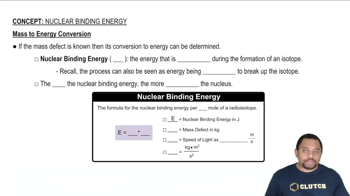
Energy Consumption Calculations

Rutherfordium-257 was synthesized by bombarding Cf-249 with C-12. Write the nuclear equation for this reaction.
If 1.0 g of matter is converted to energy, how much energy is formed?
Calculate the mass defect and nuclear binding energy per nucleon of each nuclide. a. Li-7 (atomic mass = 7.016003 amu)
Calculate the quantity of energy produced per gram of U-235 (atomic mass = 235.043922 amu) for the neutron-induced fission of U-235 to form Xe-144 (atomic mass = 143.9385 amu) and Sr-90 (atomic mass = 89.907738 amu) (discussed in Problem 57).
Calculate the quantity of energy produced per mole of U-235 (atomic mass = 235.043922 amu) for the neutron-induced fission of U-235 to produce Te-137 (atomic mass = 136.9253 amu) and Zr-97 (atomic mass = 96.910950 amu) (discussed in Problem 58).
