For each reaction, calculate ΔH°rxn, ΔS°rxn, and ΔG°rxn at 25 °C and state whether or not the reaction is spontaneous. If the reaction is not spontaneous, would a change in temperature make it spontaneous? If so, should the temperature be raised or lowered from 25 °C? d. 2 KClO3(s) → 2 KCl(s) + 3 O2(g)
Determine ΔG° for the reaction: Fe2O3(s) + 3 CO(g) → 2 Fe(s) + 3 CO2(g) Use the following reactions with known ΔG°rxn values:
2 Fe(s) + 3/2 O2(g) → Fe2O3(s) ΔG°rxn = -742.2 kJ
CO(g) + 12 O2( g) → CO2(g) ΔG°rxn = -257.2 kJ
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Gibbs Free Energy (ΔG°)

Standard Reaction Gibbs Free Energy (ΔG°rxn)

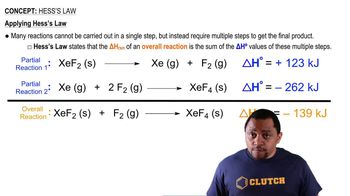
Hess's Law
Use standard free energies of formation to calculate ΔG° at 25 °C for each reaction in Problem 61. How do the values of ΔG° calculated this way compare to those calculated from ΔH° and ΔS°? Which of the two methods could be used to determine how ΔG° changes with temperature?
Consider the reaction: 2 NO(g) + O2(g) → 2 NO2(g) Estimate ΔG° for this reaction at each temperature and predict whether or not the reaction is spontaneous. (Assume that ΔH° and ΔS° do not change too much within the given temperature range.) b. 715 K
Consider the sublimation of iodine at 25.0 °C : I2(s) → I2(g) b. Find ΔG°rxn at 25.0 °C under the following nonstandard conditions: i. PI2 = 1.00 mmHg ii. PI2 = 0.100 mmHg
Consider the sublimation of iodine at 25.0 °C : I2(s) → I2(g) c. Explain why iodine spontaneously sublimes in open air at 25.0 °C
Consider the evaporation of methanol at 25.0 °C : CH3OH(l) → CH3OH(g) a. Find ΔG°r at 25.0 °C.
