A gaseous hydrogen- and carbon-containing compound is decomposed and found to contain 82.66% carbon and 17.34% hydrogen by mass. The mass of 158 mL of the gas, measured at 556 mmHg and 25 °C, was 0.275 g. What is the molecular formula of the compound?

Verified step by step guidance

1. Convert the given percentages of carbon and hydrogen to grams assuming a 100 g sample, which gives 82.66 g of carbon and 17.34 g of hydrogen.
2. Calculate the number of moles of carbon and hydrogen using their respective molar masses: \( \text{Molar mass of C} = 12.01 \, \text{g/mol} \) and \( \text{Molar mass of H} = 1.008 \, \text{g/mol} \).
3. Determine the simplest whole number ratio of moles of carbon to moles of hydrogen to find the empirical formula.
4. Use the ideal gas law \( PV = nRT \) to calculate the number of moles of the gas, where \( P = 556 \, \text{mmHg} \), \( V = 158 \, \text{mL} \), \( R = 0.0821 \, \text{L} \, \text{atm/mol} \, \text{K} \), and \( T = 25 \, ^\circ\text{C} \).
5. Calculate the molar mass of the compound using the mass of the gas and the moles calculated, then compare it with the empirical formula mass to determine the molecular formula.

Key Concepts

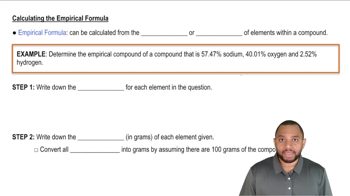
Empirical Formula Calculation

The empirical formula represents the simplest whole-number ratio of elements in a compound. To determine it, the mass percentages of each element are converted to moles by dividing by their atomic masses. The resulting mole ratios are then simplified to the smallest whole numbers, providing a foundational understanding of the compound's composition.

Ideal Gas Law

The Ideal Gas Law (PV=nRT) relates the pressure, volume, temperature, and number of moles of a gas. It allows for the calculation of the number of moles from the given conditions of pressure and temperature. Understanding this law is crucial for converting the mass of the gas into moles, which is necessary for determining the molecular formula.

Molecular Formula Determination

The molecular formula of a compound indicates the actual number of atoms of each element present in a molecule. It can be derived from the empirical formula by comparing the molar mass of the compound to the molar mass of the empirical formula. This step is essential for identifying the true composition of the compound after calculating the empirical formula.