A gas mixture contains 75.2% nitrogen and 24.8% krypton by mass. What is the partial pressure of krypton in the mixture if the total pressure is 745 mmHg?

Verified step by step guidance

1. Convert the percentage by mass of krypton to a mole fraction. Assume a sample size of 100 g for simplicity, which means you have 24.8 g of krypton.
2. Calculate the number of moles of krypton using its molar mass (approximately 83.8 g/mol).
3. Calculate the number of moles of nitrogen using its molar mass (approximately 28.0 g/mol) from the remaining mass (75.2 g of nitrogen).
4. Determine the total number of moles in the mixture by adding the moles of krypton and nitrogen.
5. Calculate the mole fraction of krypton by dividing the moles of krypton by the total moles in the mixture, then use the mole fraction to find the partial pressure of krypton by multiplying it by the total pressure (745 mmHg).

Key Concepts

Dalton's Law of Partial Pressures

Dalton's Law states that in a mixture of non-reacting gases, the total pressure exerted is equal to the sum of the partial pressures of each individual gas. Each gas's partial pressure is proportional to its mole fraction in the mixture. This principle is essential for calculating the contribution of each gas to the total pressure.

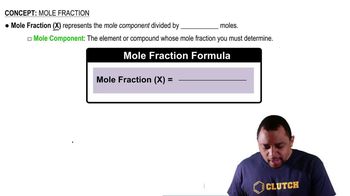
Mole Fraction

The mole fraction is a way of expressing the concentration of a component in a mixture. It is calculated by dividing the number of moles of a specific gas by the total number of moles of all gases in the mixture. In this context, the mole fraction of krypton can be derived from its mass percentage and the molar masses of nitrogen and krypton.

Ideal Gas Law

The Ideal Gas Law relates the pressure, volume, temperature, and number of moles of a gas through the equation PV = nRT. While this question primarily focuses on partial pressures, understanding the Ideal Gas Law provides a foundational context for how gases behave under various conditions, which can be useful for more complex calculations involving gas mixtures.