Copper iodide crystallizes in the zinc blende structure. The sep- aration between nearest neighbor cations and anions is approximately 311 pm, and the melting point is 606 °C. Potassium chloride, by contrast, crystallizes in the rock salt structure. Even though the separation between nearest-neighbor cations and anions is greater (319 pm), the melting point of potassium chlo- ride is higher (776 °C). Explain.
Ch.13 - Solids & Modern Materials
Chapter 13, Problem 86
Calculate the fraction of empty space in cubic closest packing to five significant figures.
 Verified step by step guidance
Verified step by step guidance1
Understand that cubic closest packing (CCP) is also known as face-centered cubic (FCC) packing.
In FCC, each unit cell contains 4 atoms. The atoms are located at each corner and the centers of each face of the cube.
Calculate the volume of the unit cell. If 'a' is the edge length of the cube, the volume is given by \( V_{cell} = a^3 \).
Determine the volume occupied by the atoms in the unit cell. The effective radius of each atom is \( r \), and the relationship between 'a' and 'r' in FCC is \( a = 2\sqrt{2}r \). The volume occupied by the atoms is \( 4 \times \frac{4}{3}\pi r^3 \).
Calculate the fraction of empty space by subtracting the volume occupied by the atoms from the total volume of the unit cell, and then divide by the total volume of the unit cell: \( \text{Fraction of empty space} = 1 - \frac{\text{Volume occupied by atoms}}{V_{cell}} \).

Verified Solution
Video duration:
5mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Cubic Closest Packing (CCP)
Cubic Closest Packing (CCP) is a method of packing spheres in three-dimensional space to maximize density. In this arrangement, spheres are stacked in layers, with each layer fitting into the gaps of the layer below. This structure achieves a packing efficiency of approximately 74%, meaning that 74% of the volume is occupied by spheres, while the remaining 26% is empty space.
Recommended video:
Guided course
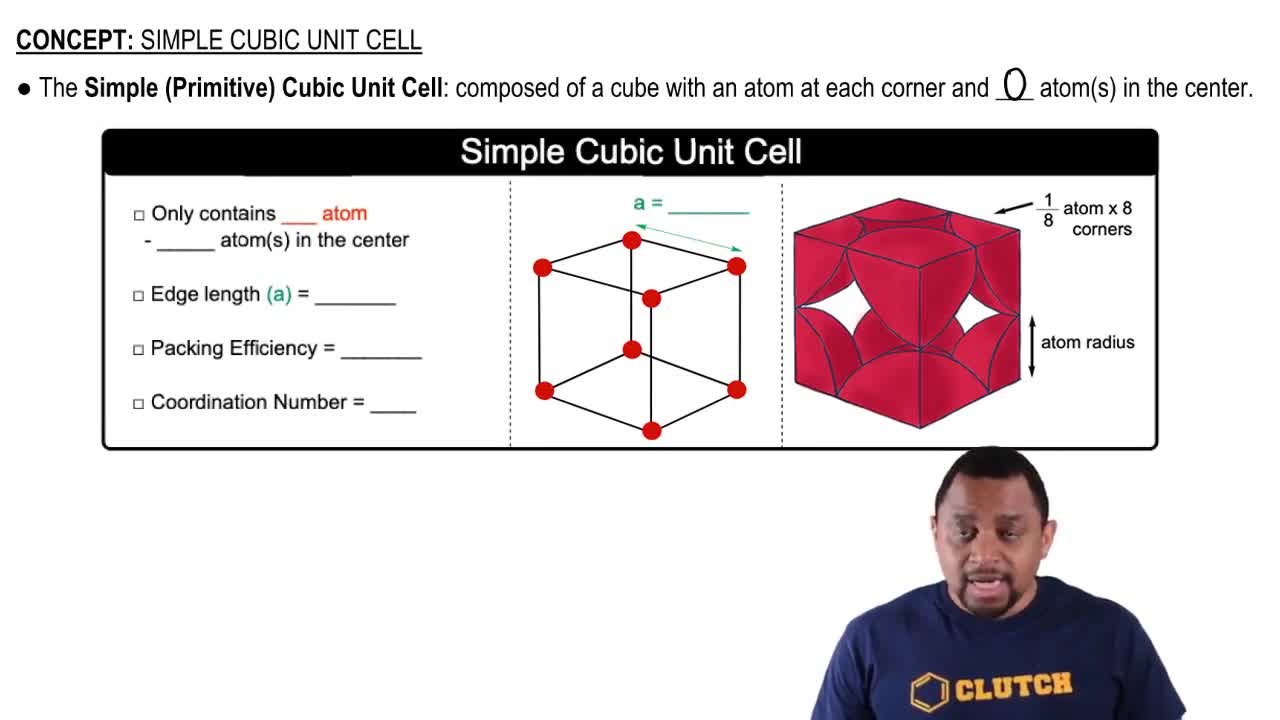
Simple Cubic Unit Cell
Packing Efficiency
Packing efficiency is a measure of how effectively a given volume is filled with particles, expressed as a percentage. In the case of cubic closest packing, the packing efficiency is calculated by dividing the volume occupied by the spheres by the total volume of the packing arrangement. Understanding packing efficiency is crucial for determining the fraction of empty space in a given packing structure.
Recommended video:
Guided course

Cubic Unit Cells
Fraction of Empty Space
The fraction of empty space in a packing arrangement is calculated by subtracting the packing efficiency from 1 (or 100% if expressed as a percentage). For cubic closest packing, with a packing efficiency of 74%, the fraction of empty space is 1 - 0.74 = 0.26. This concept is essential for understanding how much of the volume in a packed structure is not occupied by the particles.
Recommended video:
Guided course
Mole Fraction Formula
Related Practice
Textbook Question
470
views
Textbook Question
The density of an unknown metal is 12.3 g/cm3, and its atomic radius is 0.134 nm. It has a face-centered cubic lattice. Find the atomic mass of this metal
2319
views
Textbook Question
An unknown metal is found to have a density of 7.8748 g/cm3 and to crystallize in a body-centered cubic lattice. The edge of the unit cell is 0.28664 nm. Calculate the atomic mass of the metal.
2543
views
2
rank
Textbook Question
X-ray diffractometers often use metals that have had their core electrons excited as a source of X-rays. Consider the 2p → 1s transition for copper, which is called the K⍺ transition. Calculate the wavelength of X-rays (in Å) given off by the K⍺ transition if the energy given off by a mole of copper atoms is 7.77⨉105 kJ.(1Å = 10-10 m)
303
views
