Instant cold packs used to ice athletic injuries on the field contain ammonium nitrate and water separated by a thin plastic divider. When the divider is broken, the ammonium nitrate dissolves according to the endothermic reaction: NH4NO3(s) → NH4+(aq) + NO3– (aq) In order to measure the enthalpy change for this reaction, 1.25 g of NH4NO3 is dissolved in enough water to make 25.0 mL of solution. The initial temperature is 25.8 °C and the final temperature (after the solid dissolves) is 21.9 °C. Calculate the change in enthalpy for the reaction in kJ. (Use 1.0 g/mL as the density of the solution and 4.18 J/g•°C as the specific heat capacity.)
For each generic reaction, determine the value of ΔH2 in terms of ΔH1.
c. A → B + 2 C ΔH1
1/2 B + C → 1/2 A ΔH2 = ?

Verified Solution
Key Concepts
Hess's Law
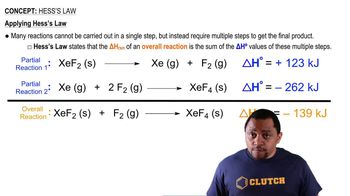
Enthalpy Change (ΔH)

Stoichiometry in Reactions

For each generic reaction, determine the value of ΔH2 in terms of ΔH1.
a. A + B → 2 C ΔH1
2 C→ A + B ΔH2 = ?
For each generic reaction, determine the value of ΔH2 in terms of ΔH1.
b. A + 1/2 B → C ΔH1
2 A + B → 2 C ΔH2 = ?
Consider the generic reaction:
A + 2 B → C + 3 D ΔH = 155 kJ
Determine the value of ΔH for each related reaction.
a. 3 A + 6 B → 3 C + 9 D
b. C + 3 D → A + 2 B
c. 1/2 C + 3/2 D → 1/2 A + B
Calculate ΔHrxn for the reaction:
Fe2O3(s) + 3 CO(g) → 2 Fe(s) + 3 CO2(g)
Use the following reactions and given ΔH's:
2 Fe(s) + 3/2 O2(g) → Fe2O3(s) ΔH = –824.2 kJ
CO(g) + 1/2 O2(g) → CO2(g) ΔH = –282.7 kJ
Calculate ΔHrxn for the reaction:
CaO(s) + CO2(g) → CaCO3(s)
Use the following reactions and given ΔH's:
Ca(s) + CO2(g) + 1/2 O2(g) → CaCO3(s) ΔH = –812.8 kJ
2 Ca(s) + O2(g) → 2 CaO(s) ΔH = –1269.8 kJ
