Complete each nuclear equation and calculate the energy change (in J/mol of reactant) associated with each (Al-27 = 26.981538 amu, Am-241 = 241.056822 amu, He-4 = 4.002603 amu, Np-237 = 237.048166 amu, P-30 = 29.981801 amu, S-32 = 31.972071 amu, and Si-29 = 28.976495 amu).
a. 2713Al + 42He → 3015P + ____

Verified Solution
Key Concepts
Nuclear Reactions
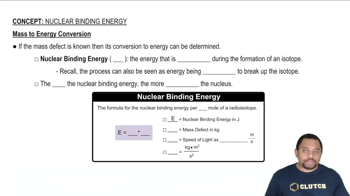
Mass-Energy Equivalence

Atomic Mass Units (amu)

Suppose a patient is given 1.55 mg of I-131, a beta emitter with a half-life of 8.0 days. Assuming that none of the I-131 is eliminated from the person's body in the first 4.0 hours of treatment, what is the exposure (in Ci) during those first four hours?
Complete each nuclear equation and calculate the energy change (in J/mol of reactant) associated with each (Be-9 = 9.012182 amu, Bi-209 = 208.980384 amu, He-4 = 4.002603 amu, Li-6 = 6.015122 amu, Ni-64 = 63.927969 amu, Rg-272 = 272.1535 amu, Ta-179 = 178.94593 amu, and W-179 = 178.94707 amu). a. _____ + 94Be → 63Li + 42He
Write the nuclear equation for the most likely mode of decay for each unstable nuclide. b. Ra-216
Write the nuclear equation for the most likely mode of decay for each unstable nuclide. a. Kr-74 b. Th-221 c. Ar-44 d. Nb-85
