Calculate the mass defect and nuclear binding energy per nucleon of each nuclide. a. O-16 (atomic mass = 15.994915 amu) b. Ni-58 (atomic mass = 57.935346 amu) c. Xe-129 (atomic mass = 128.904780 amu)
Ch.20 - Radioactivity and Nuclear Chemistry
Chapter 20, Problem 70
Calculate the quantity of energy produced per mole of U-235 (atomic mass = 235.043922 amu) for the neutron-induced fission of U-235 to produce Te-137 (atomic mass = 136.9253 amu) and Zr-97 (atomic mass = 96.910950 amu) (discussed in Problem 58).
 Verified step by step guidance
Verified step by step guidance1
Identify the nuclear reaction: U-235 undergoes fission when it absorbs a neutron, resulting in the formation of Te-137, Zr-97, and additional neutrons.
Calculate the mass defect: Determine the difference in mass between the reactants (U-235 and a neutron) and the products (Te-137, Zr-97, and additional neutrons).
Convert the mass defect to energy: Use Einstein's equation, \( E = \Delta m c^2 \), where \( \Delta m \) is the mass defect and \( c \) is the speed of light, to find the energy released per fission event.
Determine the energy per mole: Multiply the energy per fission event by Avogadro's number (\( 6.022 \times 10^{23} \) mol\(^{-1}\)) to find the energy produced per mole of U-235.
Summarize the process: The energy produced per mole of U-235 is the result of converting the mass defect into energy, as described by Einstein's equation, and scaling it to a molar quantity using Avogadro's number.

Verified Solution
Video duration:
6mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Nuclear Fission
Nuclear fission is a process in which a heavy nucleus, such as uranium-235, splits into two smaller nuclei, along with the release of energy and neutrons. This reaction can be initiated by the absorption of a neutron, leading to a chain reaction that can produce significant amounts of energy, which is the principle behind nuclear reactors and atomic bombs.
Recommended video:
Guided course

Band of Stability: Nuclear Fission
Mass-Energy Equivalence
Mass-energy equivalence, expressed by Einstein's equation E=mc², states that mass can be converted into energy and vice versa. In nuclear reactions, the mass of the products is often less than the mass of the reactants, and this 'missing' mass is converted into energy, which can be calculated to determine the energy released during fission.
Recommended video:
Guided course

Energy to Mass Conversion
Binding Energy
Binding energy is the energy required to disassemble a nucleus into its constituent protons and neutrons. It is a measure of the stability of a nucleus; higher binding energy indicates a more stable nucleus. In fission, the binding energy of the products is greater than that of the reactants, resulting in the release of energy during the reaction.
Recommended video:
Guided course
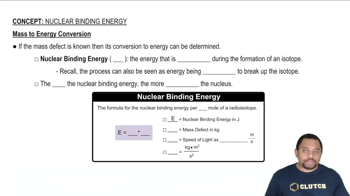
Nuclear Binding Energy
Related Practice
Textbook Question
Textbook Question
Calculate the mass defect and nuclear binding energy per nucleon of each nuclide. a. Li-7 (atomic mass = 7.016003 amu)
1438
views
Textbook Question
Calculate the quantity of energy produced per gram of U-235 (atomic mass = 235.043922 amu) for the neutron-induced fission of U-235 to form Xe-144 (atomic mass = 143.9385 amu) and Sr-90 (atomic mass = 89.907738 amu) (discussed in Problem 57).
1942
views
1
rank
Open Question
Calculate the quantity of energy produced per gram of reactant for the fusion of two H-2 (atomic mass = 2.014102 amu) atoms to form He-3 (atomic mass = 3.016029 amu) and one neutron.
Open Question
Calculate the quantity of energy produced per gram of reactant for the fusion of H-3 (atomic mass = 3.016049 amu) with H-1 (atomic mass = 1.007825 amu) to form He-4 (atomic mass = 4.002603 amu).
Textbook Question
A 75-kg human has a dose of 32.8 rad of radiation. How much energy is absorbed by the person's body? Compare this energy to the amount of energy absorbed by the person's body if he or she jumped from a chair to the floor (assume that the chair is 0.50 m from the ground and that all of the energy from the fall is absorbed by the person).
805
views
