Here are the essential concepts you must grasp in order to answer the question correctly.
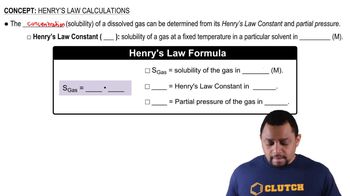
Henry's Law
Henry's Law states that the amount of gas that dissolves in a liquid at a given temperature is directly proportional to the partial pressure of that gas above the liquid. This principle is crucial for understanding how the concentration of oxygen in water relates to its partial pressure in the atmosphere.
Recommended video:
Gas Solubility
Gas solubility refers to the maximum amount of gas that can dissolve in a solvent at a specific temperature and pressure. In this case, the solubility of O2 in water at 0 °C and 1 atm is given as 2.21 * 10^-3 mol/L, which helps determine how much oxygen can be dissolved to meet the survival needs of fish.
Recommended video:
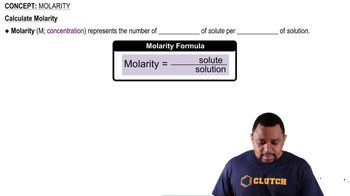
Molar Concentration
Molar concentration, or molarity, is a measure of the concentration of a solute in a solution, expressed in moles per liter (mol/L). To find the required partial pressure of oxygen to achieve a concentration of 4 mg/L, it is essential to convert this mass concentration into molarity using the molar mass of oxygen.
Recommended video: