What is the change in entropy (∆S) when 1.32 g of propane (C3H8) at 0.100 atm pressure is compressed by a factor of five at a constant temperature at 20°C? Assume that propane behaves as an ideal gas. (a) ∆S = +13 J/K (b) ∆S = -13 J/K (c) ∆S = - 0.40 J/K (d) ∆S = + 0.40 J/K

Verified step by step guidance

1. Identify the molar mass of propane (C3H8). Calculate the number of moles of propane using the formula: number of moles = mass / molar mass.
2. Use the ideal gas law to determine the initial volume of the gas. The ideal gas law is PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the gas constant, and T is the temperature in Kelvin.
3. Calculate the final volume of the gas after compression. Since the gas is compressed by a factor of five, the final volume is one fifth of the initial volume.
4. Use the formula for the change in entropy for an ideal gas undergoing isothermal compression or expansion: \(\Delta S = nR \ln(\frac{V_f}{V_i})\), where \(V_f\) is the final volume and \(V_i\) is the initial volume.
5. Substitute the values of n, R, \(V_f\), and \(V_i\) into the entropy change formula to find the change in entropy (\(\Delta S\)).

Key Concepts

Entropy (S)

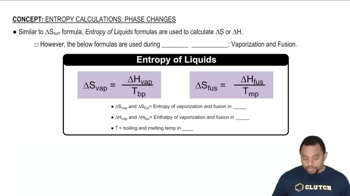
Entropy is a measure of the disorder or randomness in a system. In thermodynamics, it quantifies the amount of energy in a physical system that is not available to do work. Higher entropy indicates greater disorder, while lower entropy suggests more order. Understanding how entropy changes during processes, such as compression or expansion, is crucial for predicting the spontaneity of reactions and the direction of energy flow.

Ideal Gas Law

The Ideal Gas Law relates the pressure, volume, temperature, and number of moles of an ideal gas through the equation PV = nRT. This law assumes that gas particles do not interact and occupy no volume, which simplifies calculations. In this question, the behavior of propane as an ideal gas allows us to apply this law to determine changes in state variables, such as pressure and volume, when the gas is compressed.

Change in Entropy for Compression

The change in entropy (∆S) during a process can be calculated using the formula ∆S = nR ln(Vf/Vi) for an ideal gas, where Vf and Vi are the final and initial volumes, respectively. In this case, compressing the gas by a factor of five reduces its volume, leading to a negative change in entropy, as the system becomes more ordered. Understanding this relationship is essential for solving the problem and determining the correct answer.