What is the pH at the equivalence point for the titration of 0.10 M solutions of the following acids and bases, and which of the indicators in Figure 17.5 would be suitable for each titration? (a) HNO2 and NaOH
Ch.17 - Applications of Aqueous Equilibria
Chapter 17, Problem 95a
Consider the titration of 50.0 mL of a 0.100 M solution of the protonated form of the amino acid alanine (H2A+: Ka1 = 4.6 × 10–3, Ka2 = 2.0 × 10–10) with 0.100 M NaOH. Calculate the pH after the addition of each of the following volumes of base. (a) 10.0 mL
 Verified step by step guidance
Verified step by step guidance1
Step 1: Determine the initial moles of the protonated form of alanine (H2A+). Use the formula: moles = concentration × volume. Here, the initial concentration is 0.100 M and the volume is 50.0 mL.
Step 2: Calculate the moles of NaOH added. Use the formula: moles = concentration × volume. The concentration of NaOH is 0.100 M and the volume added is 10.0 mL.
Step 3: Determine the reaction between H2A+ and NaOH. The reaction is: H2A+ + OH- → HA + H2O. Calculate the moles of H2A+ and OH- after the reaction.
Step 4: Identify the species present in the solution after the reaction. Since NaOH is a strong base, it will react completely with H2A+. Determine the remaining moles of H2A+ and the moles of HA formed.
Step 5: Calculate the pH of the solution. Since HA is a weak acid, use the Henderson-Hasselbalch equation: pH = pKa1 + log([A-]/[HA]) to find the pH, where pKa1 corresponds to the first dissociation constant of H2A+.

Verified Solution
Video duration:
7mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Titration
Titration is a quantitative analytical technique used to determine the concentration of a solute in a solution. It involves the gradual addition of a titrant (in this case, NaOH) to a solution containing the analyte (the protonated form of alanine) until the reaction reaches its equivalence point. The pH changes during this process can be monitored to understand the acid-base behavior of the solution.
Recommended video:
Guided course
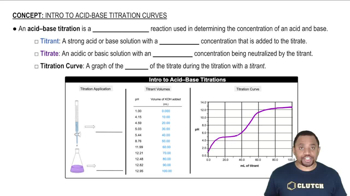
Acid-Base Titration
Acid-Base Equilibria
Acid-base equilibria describe the balance between protonated and deprotonated forms of a substance in solution. For alanine, the two dissociation constants (Ka1 and Ka2) indicate how readily it donates protons. Understanding these constants is crucial for calculating the pH at various points during the titration, particularly before and after the equivalence point.
Recommended video:
Guided course

Arrhenius Acids and Bases
Henderson-Hasselbalch Equation
The Henderson-Hasselbalch equation provides a way to calculate the pH of a buffer solution based on the concentrations of the acid and its conjugate base. It is expressed as pH = pKa + log([A-]/[HA]). This equation is particularly useful in titration scenarios, as it allows for the determination of pH at points where both the acid and its conjugate base are present, such as after the addition of a specific volume of NaOH.
Recommended video:
Guided course

Henderson-Hasselbalch Equation
Related Practice
Textbook Question
408
views
Textbook Question
What is the pH at the equivalence point for the titration of 0.10 M solutions of the following acids and bases, and which of the indicators in Figure 17.5 would be suitable for each titration? (c) CH3NH2 (methylamine) and HCl
539
views
Textbook Question
What is the pH at the equivalence point for the titration of 0.20 M solutions of the following acids and bases? Which of the indicators in Figure 17.5 would be suitable for each titration?
(c) Ba(OH)2 and HBr
379
views
Textbook Question
Consider the titration of 50.0 mL of a 0.100 M solution of the protonated form of the amino acid alanine (H2A+: Ka1 = 4.6 × 10–3, Ka2 = 2.0 × 10–10) with 0.100 M NaOH. Calculate the pH after the addition of each of the following volumes of base. (d) 75.0 mL
911
views
Textbook Question
Consider the titration of 50.0 mL of a 0.100 M solution of the protonated form of the amino acid alanine (H2A+: Ka1 = 4.6 × 10–3, Ka2 = 2.0 × 10–10) with 0.100 M NaOH. Calculate the pH after the addition of each of the following volumes of base. (e) 100.0 mL
485
views
Open Question
Consider the titration of 25.0 mL of 0.0200 M H2CO3 with 0.0250 M KOH. Calculate the pH after the addition of each of the following volumes of base: (a) 10.0 mL, (b) 20.0 mL, (c) 30.0 mL, (d) 40.0 mL.
