Consider the first-order decomposition of A molecules (red spheres) in three vessels of equal volume. (1)-(3)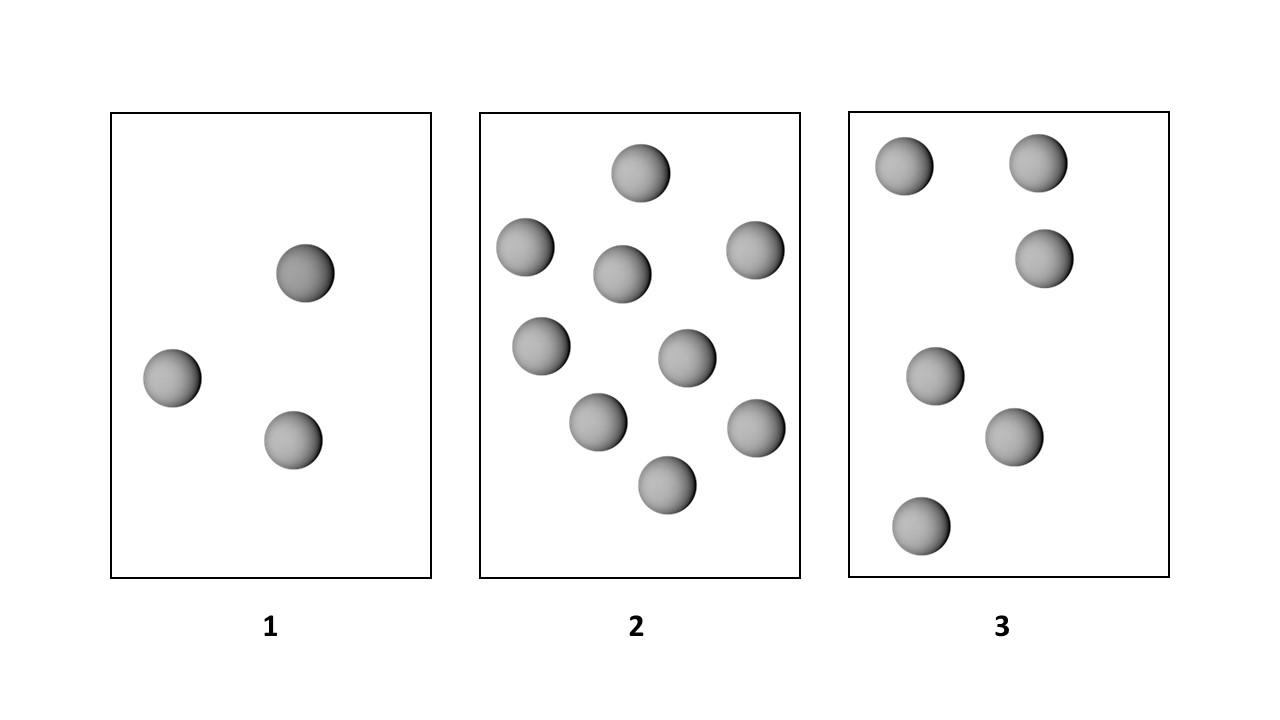
(c) How will the rates and half-lives be affected if the volume of each vessel is decreased by a factor of 2?

Verified step by step guidance

1. Identify that the problem involves the first-order decomposition of A molecules in three vessels of equal volume.
2. Recall that for a first-order reaction, the rate law is given by: rate = k[A], where k is the rate constant and [A] is the concentration of A.
3. Understand that if the volume of each vessel is decreased by a factor of 2, the concentration of A will double because concentration is inversely proportional to volume.
4. Note that the rate of the reaction will increase because the concentration of A has increased. Specifically, the new rate will be twice the original rate since rate = k[A] and [A] has doubled.
5. Remember that the half-life (t_1/2) for a first-order reaction is given by: t_1/2 = 0.693/k. Since the rate constant k does not change with concentration, the half-life will remain the same even if the volume is decreased.

Key Concepts

First-Order Reactions

First-order reactions are chemical reactions where the rate is directly proportional to the concentration of one reactant. This means that if the concentration of the reactant doubles, the rate of reaction also doubles. The rate law for a first-order reaction can be expressed as rate = k[A], where k is the rate constant and [A] is the concentration of the reactant.

Effect of Volume on Concentration

Decreasing the volume of a reaction vessel increases the concentration of the reactants, as concentration is defined as the amount of substance per unit volume. For a fixed number of molecules, halving the volume will double the concentration, which in turn affects the rate of reaction for first-order kinetics, leading to an increase in the reaction rate.

Half-Life of Reactions

The half-life of a reaction is the time required for the concentration of a reactant to decrease to half its initial value. For first-order reactions, the half-life is independent of the initial concentration and is given by the equation t1/2 = 0.693/k. This means that while the rate of reaction may change with concentration, the half-life remains constant regardless of the volume changes.