In 2010, a team of scientists from Russia and the United States reported creation of the first atom of element 117, which is named tennessine, and whose symbol is Ts. The synthesis involved the collision of a target of 24997Bk with accelerated ions of an isotope which we will denote Q. The product atom, which we will call Z, immediately releases neutrons and forms 294117Ts: 24997Bk + Q → Z → 294117Ts + 3 10n (b) Isotope Q is unusual in that it is very long-lived (its half-life is on the order of 1019 yr) in spite of having an unfavorable neutron-to-proton ratio (Figure 21.1). Can you propose a reason for its unusual stability?
Ch.21 - Nuclear Chemistry
Chapter 21, Problem 82
The nuclear masses of 7Be, 9Be, and 10Be are 7.0147, 9.0100, and 10.0113 amu, respectively. Which of these nuclei has the largest binding energy per nucleon?
 Verified step by step guidance
Verified step by step guidance1
Step 1: The binding energy of a nucleus is the energy required to disassemble a nucleus into its constituent protons and neutrons. It is equivalent to the mass defect of the nucleus, which is the difference between the mass of the nucleus and the sum of the masses of its individual protons and neutrons.
Step 2: To find the binding energy, we first need to calculate the mass defect. The mass defect (Δm) can be calculated using the formula Δm = Zm_p + Nm_n - M, where Z is the number of protons, m_p is the mass of a proton, N is the number of neutrons, m_n is the mass of a neutron, and M is the mass of the nucleus.
Step 3: For each isotope of Beryllium (7Be, 9Be, and 10Be), calculate the mass defect using the given nuclear masses and the known masses of a proton (1.0073 amu) and a neutron (1.0087 amu).
Step 4: Convert the mass defect from atomic mass units (amu) to energy using Einstein's equation E=mc^2, where E is energy, m is mass, and c is the speed of light. Note that 1 amu is equivalent to 931.5 MeV/c^2.
Step 5: Finally, to find the binding energy per nucleon, divide the total binding energy by the number of nucleons (protons + neutrons) in the nucleus. The nucleus with the largest binding energy per nucleon is the most stable.

Verified Solution
Video duration:
15mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Binding Energy
Binding energy is the energy required to disassemble a nucleus into its constituent protons and neutrons. It is a measure of the stability of a nucleus; the higher the binding energy, the more stable the nucleus. This energy arises from the strong nuclear force that holds the nucleons together, overcoming the repulsive electromagnetic force between protons.
Recommended video:
Guided course
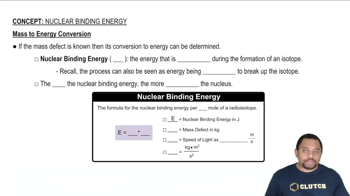
Nuclear Binding Energy
Binding Energy per Nucleon
Binding energy per nucleon is calculated by dividing the total binding energy of a nucleus by the number of nucleons (protons and neutrons) it contains. This value provides a normalized measure of nuclear stability, allowing for comparison between different nuclei. A higher binding energy per nucleon indicates a more stable nucleus, which is crucial for understanding nuclear reactions and stability.
Recommended video:
Guided course

Nuclear Binding Energy
Mass Defect
Mass defect refers to the difference between the mass of a nucleus and the sum of the individual masses of its constituent nucleons. This 'missing' mass is converted into binding energy according to Einstein's equation, E=mc². Understanding mass defect is essential for calculating the binding energy and, consequently, the binding energy per nucleon for different isotopes.
Recommended video:
Guided course

Calculating Mass Defect
Related Practice
Textbook Question
404
views
Open Question
According to current regulations, the maximum permissible dose of strontium-90 in the body of an adult is 1 mCi (1 * 10^-3 Ci). Using the relationship rate = kN, calculate the number of atoms of strontium-90 to which this dose corresponds. To what mass of strontium-90 does this correspond? The half-life for strontium-90 is 28.8 years.
Textbook Question
Each of the following transmutations produces a radionuclide used in positron emission tomography (PET).
(a) In equations (i) and (ii), identify the species signified as 'X.'
(i) 14N(p,α)X
(ii) 18O(p,X)18F
(iii) 14N(d,n)15O
485
views
Open Question
A 26.00-g sample of water containing tritium, ³¹H, emits 1.50 * 10³ beta particles per second. Tritium is a weak beta emitter with a half-life of 12.3 years. What fraction of all the hydrogen in the water sample is tritium?
Open Question
The Sun radiates energy into space at the rate of 3.9 * 1026 J/s. (a) Calculate the rate of mass loss from the Sun in kg/s. (b) How does this mass loss arise? (c) It is estimated that the Sun contains 9 * 1056 free protons. How many protons per second are consumed in nuclear reactions in the Sun?
Open Question
The average energy released in the fission of a single uranium-235 nucleus is about 3 * 10^-11 J. If the conversion of this energy to electricity in a nuclear power plant is 40% efficient, what mass of uranium-235 undergoes fission in a year in a plant that produces 1000 megawatts? Recall that a watt is 1 J/s.
