Classify each of the following reactions as one of the four possible types summarized in Table 19.3: (i) spontaneous at all temperatures; (ii) not spontaneous at any temperature; (iii) spontaneous at low T but not spontaneous at high T; (iv) spontaneous at high T but not spontaneous at low T. (c) N2F4(g) ⟶ 2 NF2(g) ΔH° = 85 kJ; ΔS° = 198 J/K
A certain constant-pressure reaction is barely nonspontaneous at 45 °C. The entropy change for the reaction is 72 J/K. Estimate ΔH.
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Gibbs Free Energy

Entropy Change (ΔS)
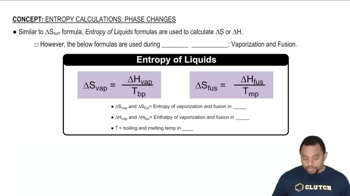
Enthalpy Change (ΔH)

From the values given for ΔH° and ΔS°, calculate ΔG° for each of the following reactions at 298 K. If the reaction is not spontaneous under standard conditions at 298 K, at what temperature (if any) would the reaction become spontaneous? a. 2 PbS(s) + 3 O2(g) → 2 PbO(s) + 2 SO2(g) ΔH° = −844 kJ; ΔS° = −165 J/K
Reactions in which a substance decomposes by losing CO are called decarbonylation reactions. The decarbonylation of acetic acid proceeds according to: CH3COOH(l) → CH3OH(g) + CO(g) By using data from Appendix C, calculate the minimum temperature at which this process will be spontaneous under standard conditions. Assume that ΔH° and ΔS° do not vary with temperature.
Consider the following reaction between oxides of nitrogen: NO2(g) + N2O(g) → 3 NO(g) (a) Use data in Appendix C to predict how ΔG for the reaction varies with increasing temperature.
