Work each problem. Which of the following is the correct complete factorization of x^4-1? A. (x^2-1)(x^2+1) B.(x^2+1)(x+1)(x-1) C. (x^2-1)^2 D. (x-1)^2(x+1)^2

Verified step by step guidance

1. Recognize that \(x^4 - 1\) is a difference of squares, which can be expressed as \((x^2)^2 - 1^2\).
2. Apply the difference of squares formula: \(a^2 - b^2 = (a-b)(a+b)\). Here, \(a = x^2\) and \(b = 1\), so \(x^4 - 1 = (x^2 - 1)(x^2 + 1)\).
3. Notice that \(x^2 - 1\) is also a difference of squares: \((x)^2 - (1)^2\).
4. Factor \(x^2 - 1\) using the difference of squares formula again: \(x^2 - 1 = (x - 1)(x + 1)\).
5. Combine the factors: \(x^4 - 1 = (x - 1)(x + 1)(x^2 + 1)\).

Key Concepts

Difference of Squares

The difference of squares is a fundamental algebraic identity stating that for any two terms a and b, the expression a^2 - b^2 can be factored as (a - b)(a + b). This concept is crucial for factoring polynomials like x^4 - 1, which can be recognized as a difference of squares where a = x^2 and b = 1.

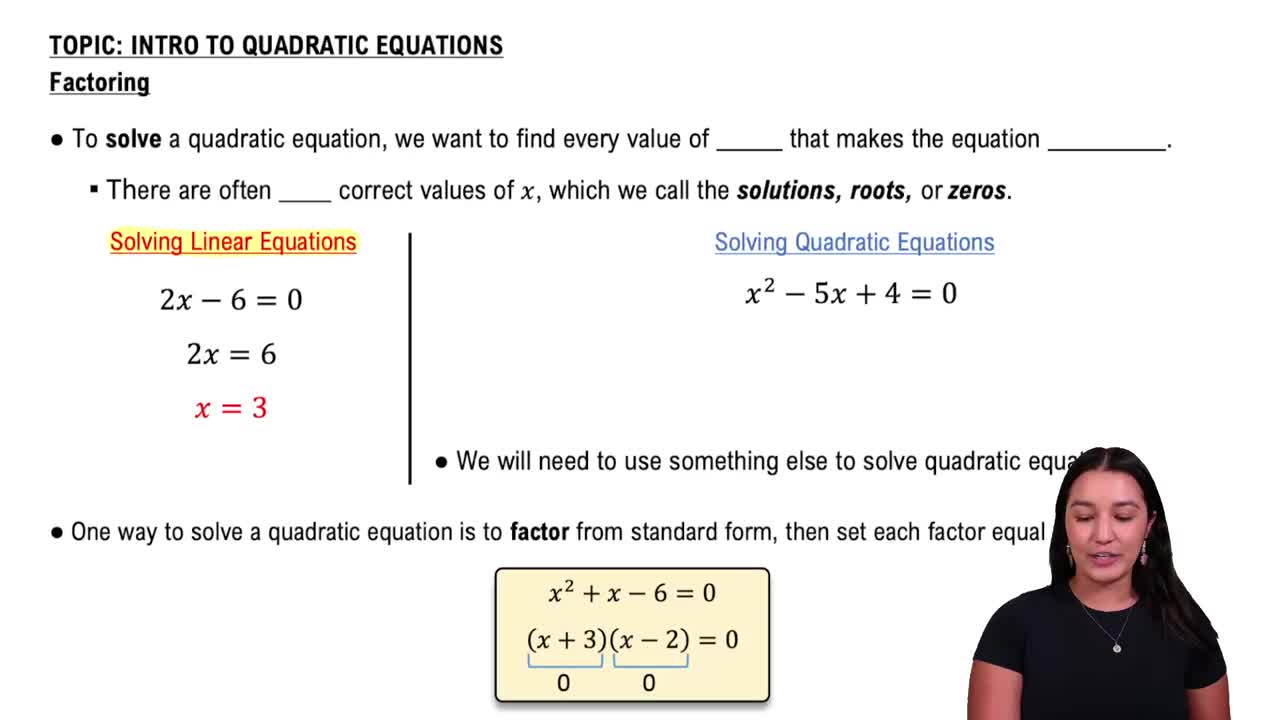
Factoring Polynomials

Factoring polynomials involves rewriting a polynomial as a product of simpler polynomials or factors. Understanding how to factor polynomials, especially those of higher degrees, is essential for simplifying expressions and solving equations. In this case, recognizing that x^4 - 1 can be factored multiple times is key to finding the complete factorization.

Quadratic Factors

Quadratic factors are expressions of the form ax^2 + bx + c, which can often be factored further into linear factors. In the context of the problem, after applying the difference of squares, the resulting quadratic factors like x^2 - 1 can be further factored into (x - 1)(x + 1), illustrating the importance of recognizing and factoring quadratics in polynomial expressions.