Here are the essential concepts you must grasp in order to answer the question correctly.
Vertex of a Quadratic Function
The vertex of a quadratic function in the form f(x) = ax^2 + bx + c represents the highest or lowest point on the graph, depending on the direction of the parabola. The x-coordinate of the vertex can be found using the formula x = -b/(2a), where a and b are the coefficients from the quadratic equation.
Recommended video:
Standard Form of a Quadratic Function
A quadratic function is typically expressed in standard form as f(x) = ax^2 + bx + c, where a, b, and c are constants. The value of 'a' determines the direction of the parabola (upward if a > 0, downward if a < 0), while 'b' and 'c' influence the position of the vertex and the y-intercept.
Recommended video:
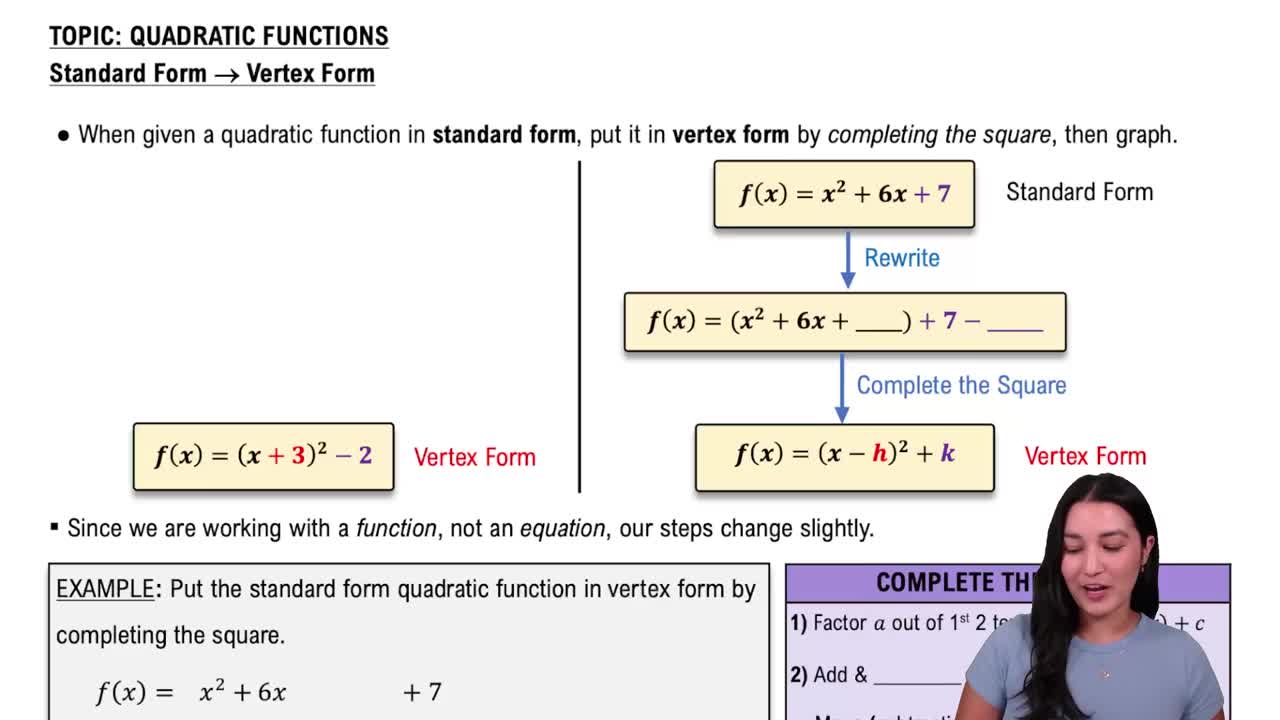
Converting Standard Form to Vertex Form
Completing the Square
Completing the square is a method used to transform a quadratic equation into vertex form, which makes it easier to identify the vertex. This technique involves rewriting the quadratic expression by adding and subtracting the square of half the coefficient of x, allowing for a clearer understanding of the graph's features, including the vertex.
Recommended video:
Solving Quadratic Equations by Completing the Square