Decide whether each statement is true or false. If false, correct the right side of the equation. √-25 = 5i

Key Concepts

Complex Numbers

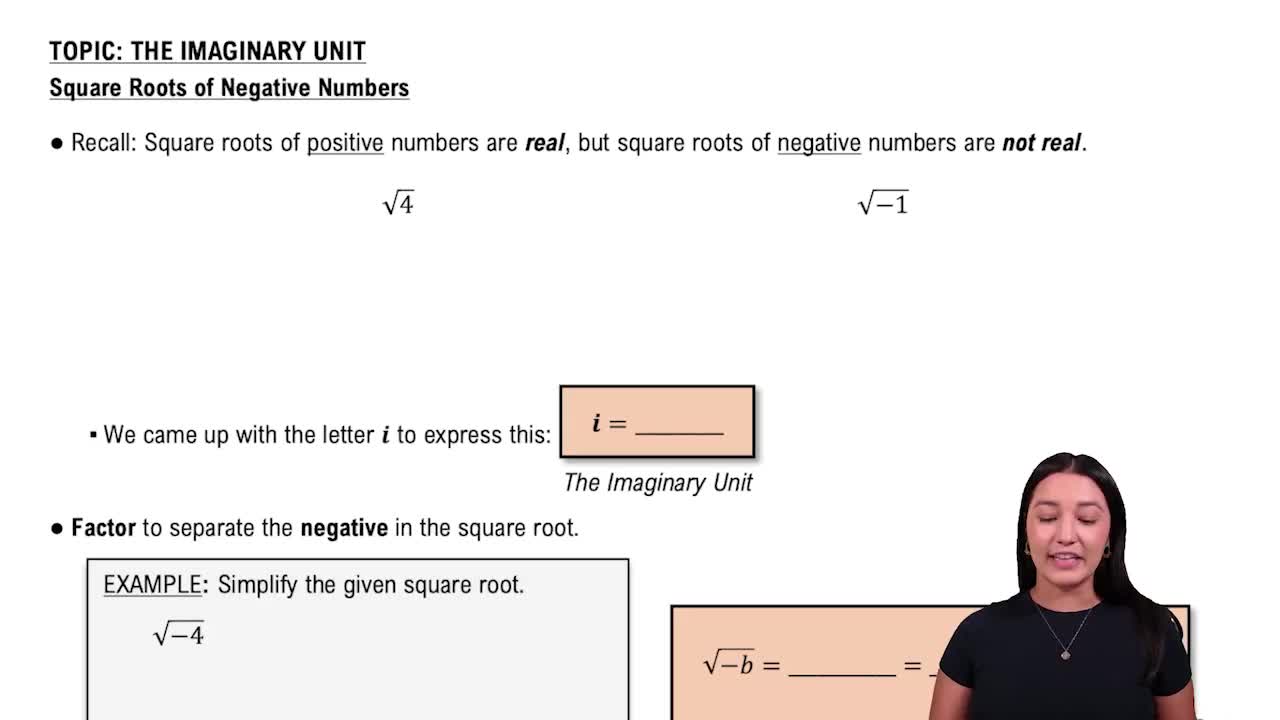
Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where 'a' is the real part and 'b' is the coefficient of the imaginary unit 'i', which is defined as the square root of -1. In this context, the expression √-25 can be rewritten as √(25) * √(-1) = 5i.

Square Roots of Negative Numbers

The square root of a negative number is not defined within the set of real numbers, but it can be expressed using imaginary numbers. For example, √-x can be expressed as i√x, allowing for the extension of the number system to include complex numbers, which is essential for solving equations involving negative square roots.

True or False Statements in Mathematics

In mathematics, determining the truth value of a statement involves verifying whether the statement holds under the defined operations and properties. In this case, the statement √-25 = 5i is true, as both sides represent the same complex number, illustrating the importance of understanding the properties of square roots and complex numbers.