Answer each question. Answer each question. Answer each question. Unknown NumbersUse the following facts.If x represents an integer, then x+1 represents the next consecutive integer.If x represents an even integer, then x+2 represents the next consecutive even integer.If x represents an odd integer, then x+2 represents the next consecutive odd integer. Find two consecutive even integers whose product is 168.

Key Concepts

Consecutive Even Integers

Consecutive even integers are pairs of integers that differ by 2 and are both even. For example, if x is an even integer, the next consecutive even integer can be expressed as x + 2. Understanding this concept is crucial for solving problems that involve finding pairs of even integers that meet specific conditions, such as a given product.

Product of Integers

The product of two integers is the result of multiplying them together. In this context, if we denote two consecutive even integers as x and x + 2, their product can be expressed as x(x + 2). This concept is essential for setting up equations to find the integers that satisfy the given condition of their product being 168.

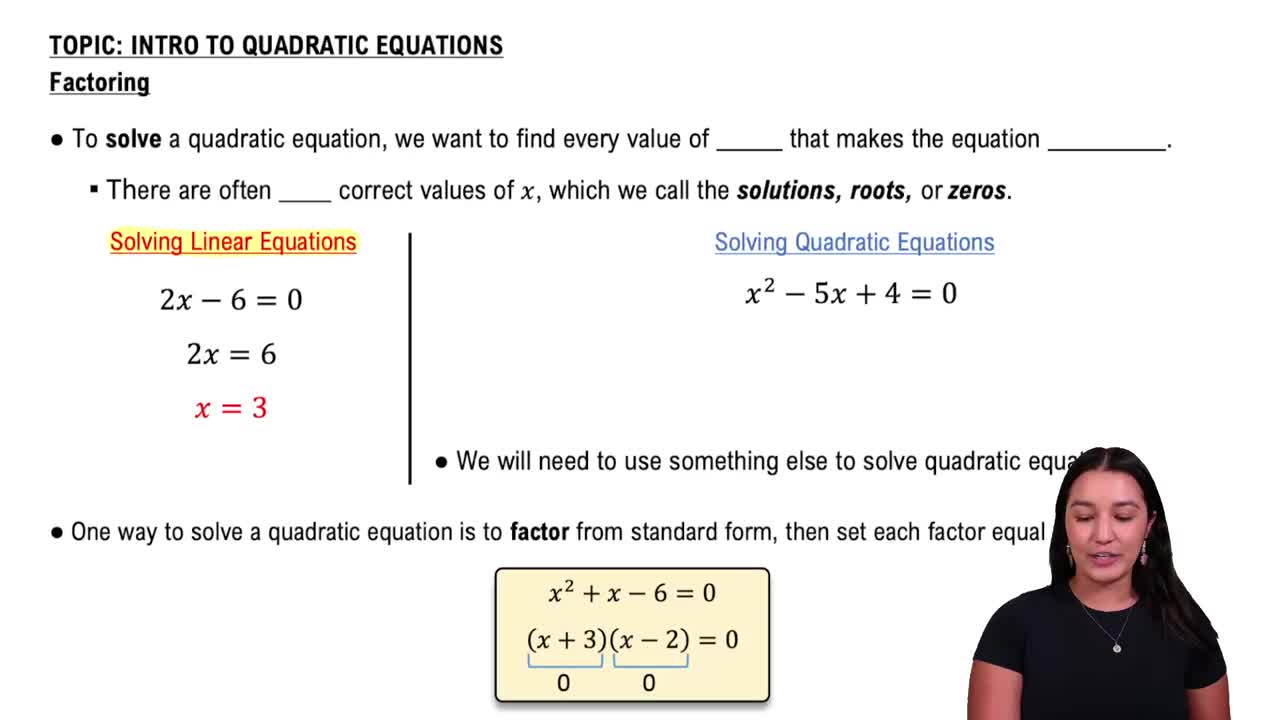
Solving Quadratic Equations

When the product of two integers is set equal to a number, it often leads to a quadratic equation. In this case, the equation x(x + 2) = 168 can be rearranged to form a standard quadratic equation. Solving quadratic equations involves finding the values of x that satisfy the equation, which is a fundamental skill in algebra.