Evaluate each expression in Exercises 1–12, or indicate that the root is not a real number. √−25

Key Concepts

Square Roots

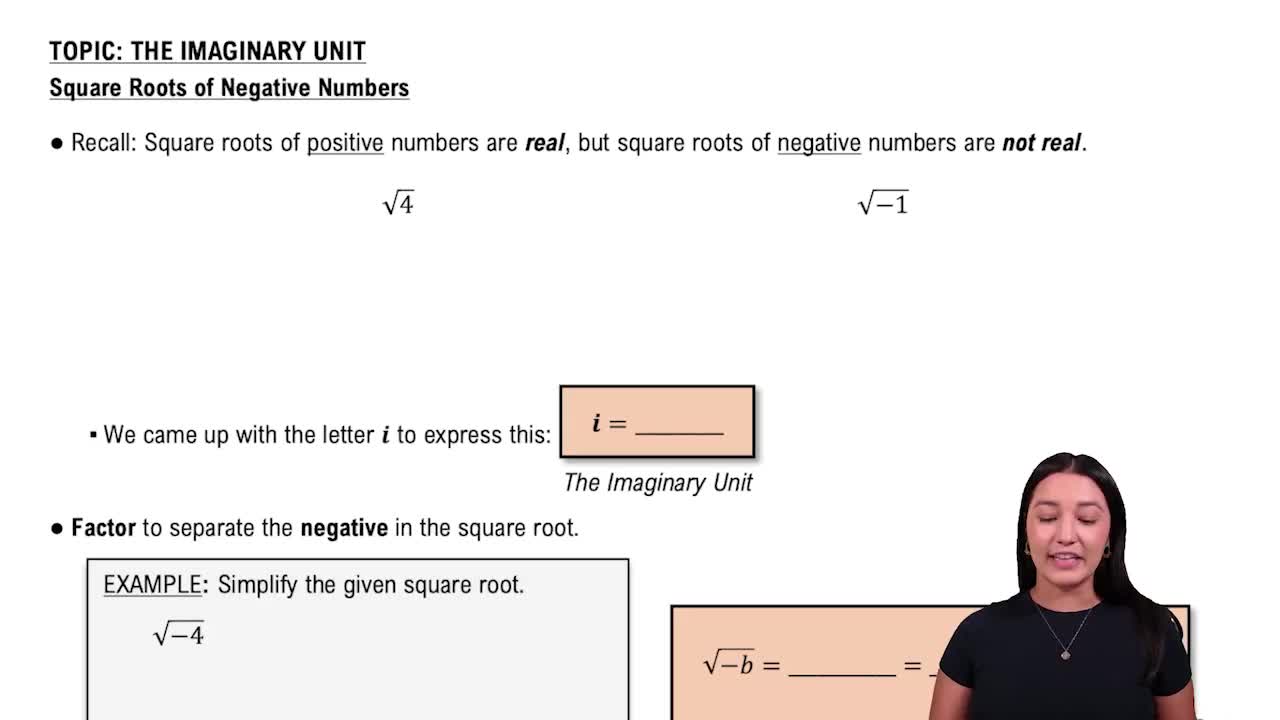
A square root of a number 'x' is a value 'y' such that y² = x. For non-negative numbers, square roots yield real numbers. However, when dealing with negative numbers, the square root is not defined within the real number system, leading to complex numbers.

Complex Numbers

Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where 'a' is the real part, 'b' is the coefficient of the imaginary unit 'i', and 'i' is defined as the square root of -1. This allows for the square roots of negative numbers to be expressed in a meaningful way.

Imaginary Unit

The imaginary unit 'i' is defined as the square root of -1. It is a fundamental concept in complex number theory, enabling the extension of the real number system to include solutions to equations that do not have real solutions, such as the square root of negative numbers.