Here are the essential concepts you must grasp in order to answer the question correctly.
Domain of a Function
The domain of a function refers to the set of all possible input values (x-values) for which the function is defined. For rational functions, the domain is typically restricted by values that would make the denominator zero, as division by zero is undefined.
Recommended video:
Domain Restrictions of Composed Functions
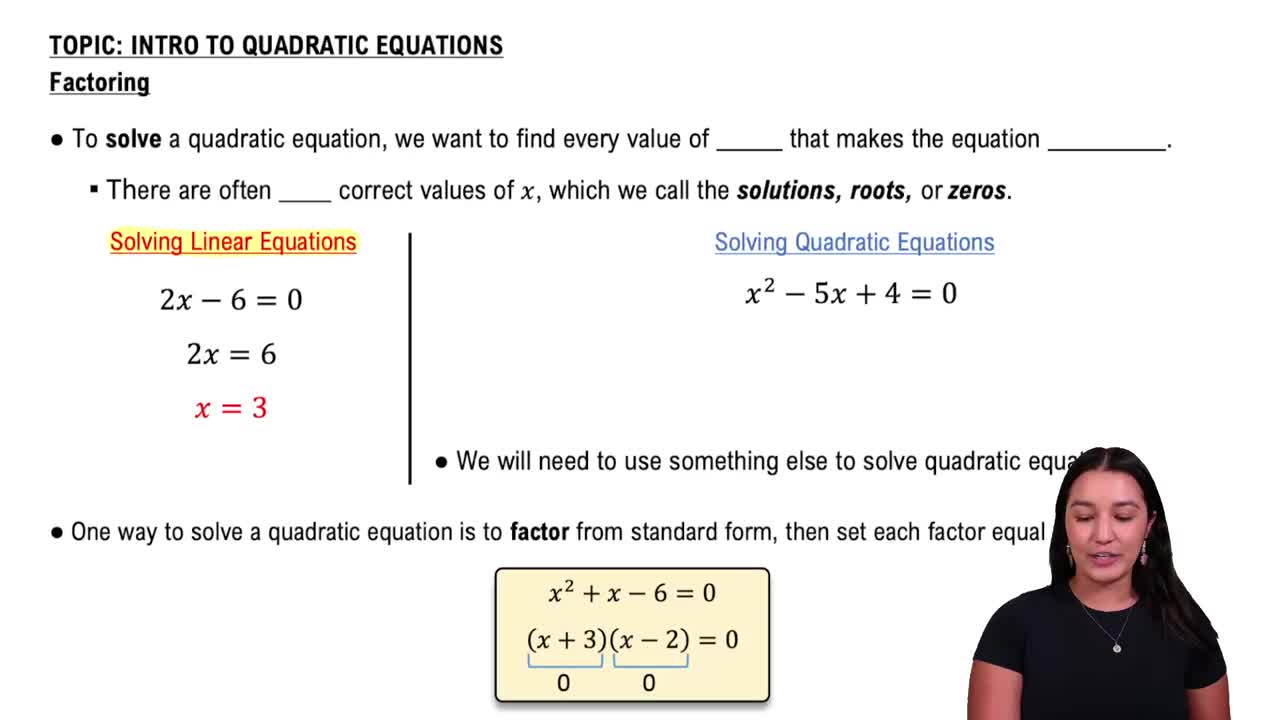
Factoring Quadratic Expressions
Factoring quadratic expressions involves rewriting them as a product of their linear factors. For the function g(x) = 3/(x^2 - 2x - 15), factoring the quadratic in the denominator helps identify the values of x that make the denominator zero, which are critical for determining the domain.
Recommended video:
Solving Quadratic Equations by Factoring
Identifying Asymptotes
Asymptotes are lines that a graph approaches but never touches. In the context of rational functions, vertical asymptotes occur at the x-values that make the denominator zero. Understanding these points is essential for determining the domain, as they indicate where the function is undefined.
Recommended video:
Introduction to Asymptotes