In Exercises 1–8, add or subtract as indicated and write the result in standard form. 6 - (- 5 + 4i) - (- 13 - i)

Verified step by step guidance

1. Identify the expression to simplify: $6 - (-5 + 4i) - (-13 - i)$.
2. Apply the distributive property to remove the parentheses: $6 + 5 - 4i + 13 + i$.
3. Combine the real numbers: $6 + 5 + 13$.
4. Combine the imaginary numbers: $-4i + i$.
5. Write the result in standard form $a + bi$, where $a$ is the sum of the real numbers and $b$ is the sum of the imaginary coefficients.

Key Concepts

Complex Numbers

Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where a is the real part and b is the coefficient of the imaginary unit i (where i = √-1). Understanding how to manipulate complex numbers is essential for performing operations like addition and subtraction.

Standard Form of Complex Numbers

The standard form of a complex number is a + bi, where a and b are real numbers. When performing operations on complex numbers, the goal is to simplify the expression to this form, ensuring that the real and imaginary parts are clearly separated and presented.

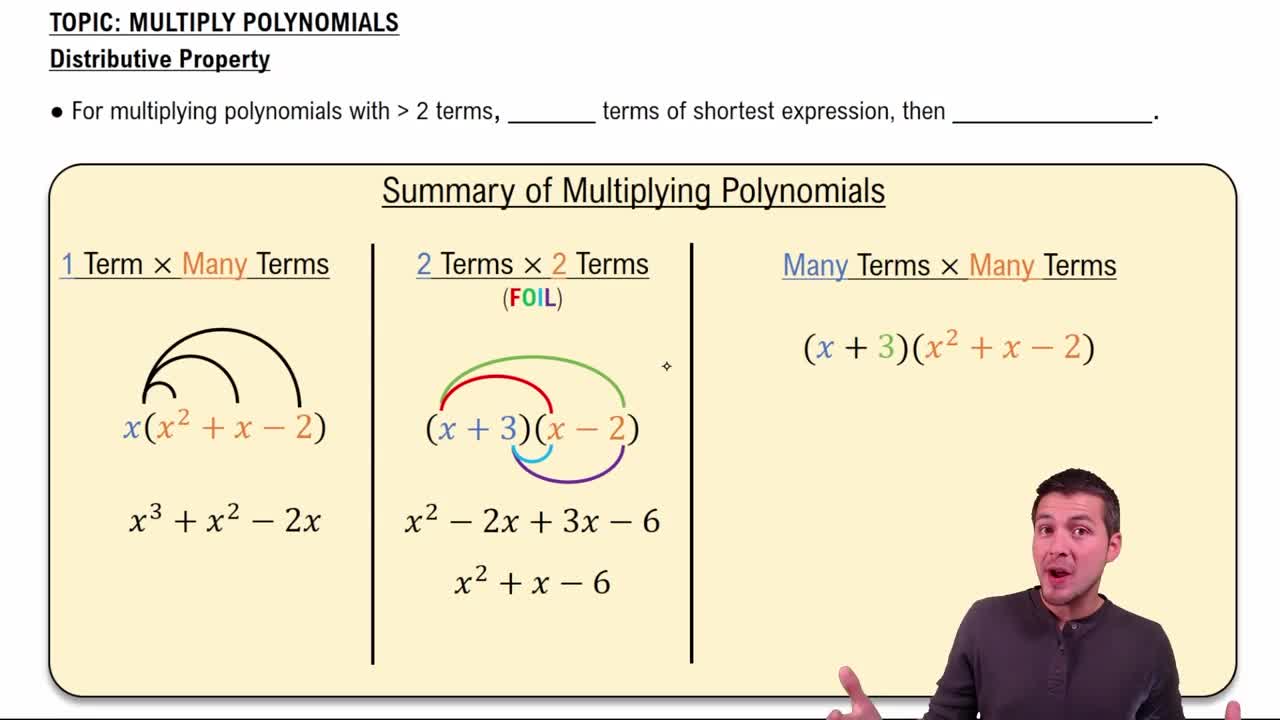
Distributive Property

The distributive property states that a(b + c) = ab + ac. This property is crucial when dealing with expressions that involve parentheses, as it allows you to distribute a term across the terms inside the parentheses, facilitating the addition or subtraction of complex numbers.