In Exercises 1–26, solve and check each linear equation. 25 - [2 + 5y - 3(y + 2)] = - 3(2y - 5) - [5(y - 1) - 3y + 3]

Verified step by step guidance

1. Distribute the terms inside the brackets on both sides of the equation: \(25 - [2 + 5y - 3(y + 2)] = -3(2y - 5) - [5(y - 1) - 3y + 3]\).
2. Simplify the expressions inside the brackets: \(25 - [2 + 5y - 3y - 6] = -3(2y - 5) - [5y - 5 - 3y + 3]\).
3. Combine like terms inside the brackets: \(25 - [2 + 2y - 6] = -3(2y - 5) - [2y - 2]\).
4. Simplify further: \(25 - [2y - 4] = -3(2y - 5) - [2y - 2]\).
5. Distribute and simplify the right side: \(25 - 2y + 4 = -6y + 15 - 2y + 2\).

Key Concepts

Linear Equations

A linear equation is an algebraic expression that represents a straight line when graphed. It typically takes the form ax + b = c, where a, b, and c are constants, and x is the variable. Solving linear equations involves isolating the variable to find its value, which can be done through various algebraic techniques such as addition, subtraction, multiplication, and division.

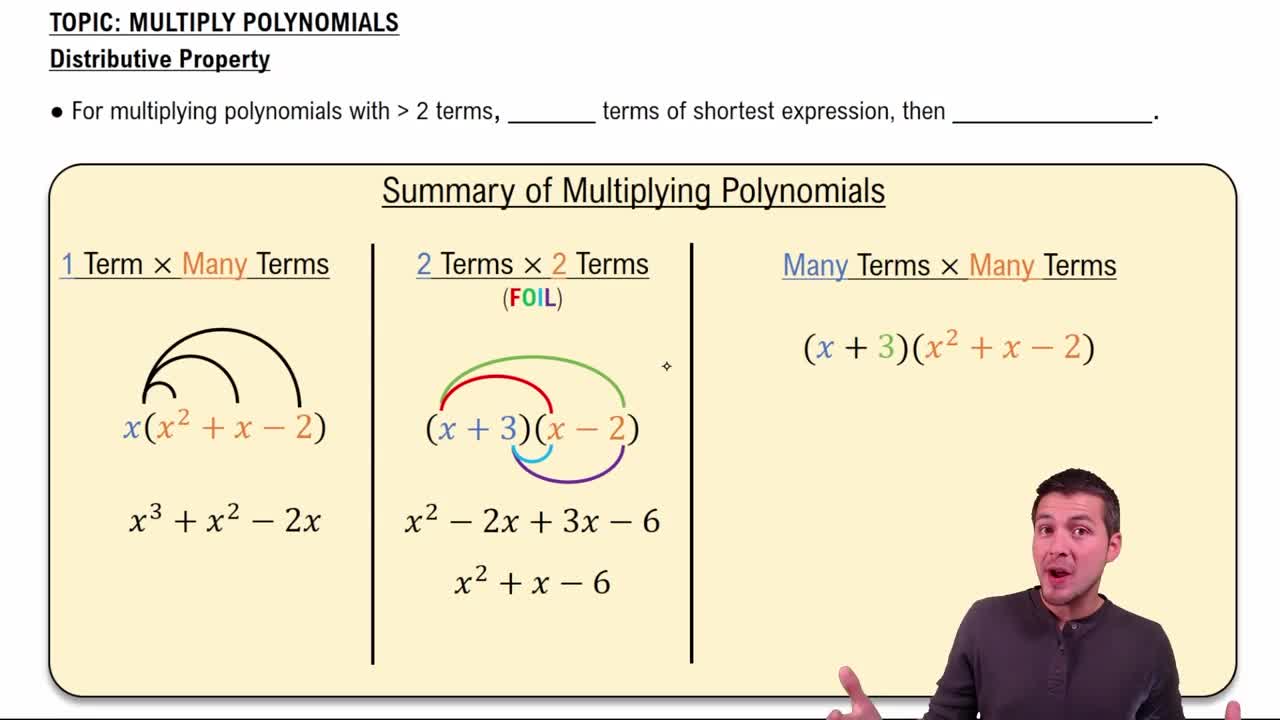
Distributive Property

The distributive property is a fundamental algebraic principle that states a(b + c) = ab + ac. This property allows us to multiply a single term by each term within a parenthesis, simplifying expressions and equations. In the context of solving linear equations, applying the distributive property is essential for expanding and rearranging terms to isolate the variable.

Combining Like Terms

Combining like terms is the process of simplifying an expression by adding or subtracting terms that have the same variable raised to the same power. This step is crucial in solving equations, as it helps to reduce the complexity of the equation, making it easier to isolate the variable. For example, in the expression 3y + 2y, combining like terms results in 5y.