Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
8. Conic Sections
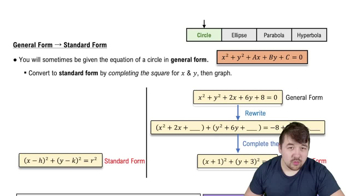
Circles
Problem 23
Textbook Question
Without actually graphing, identify the type of graph that each equation has.
16(x+3)2+16(y−2)2=1
 Verified step by step guidance
Verified step by step guidance1
Recognize the standard form of the equation for a conic section. The given equation is \( \frac{(x+3)^2}{16} + \frac{(y-2)^2}{16} = 1 \).
Compare the given equation to the standard form of an ellipse, which is \( \frac{(x-h)^2}{a^2} + \frac{(y-k)^2}{b^2} = 1 \).
Identify the center of the ellipse from the equation. The center \((h, k)\) is \((-3, 2)\) based on the transformations \(x+3\) and \(y-2\).
Notice that the denominators \(16\) and \(16\) are equal, indicating that the ellipse is actually a circle, since \(a^2 = b^2\).
Conclude that the graph of the given equation is a circle centered at \((-3, 2)\) with a radius of \(\sqrt{16} = 4\).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?

 5:18m
5:18mWatch next
Master Circles in Standard Form with a bite sized video explanation from Nick Kaneko
Start learning