Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
Graphing Systems of Inequalities
Problem 64
Textbook Question
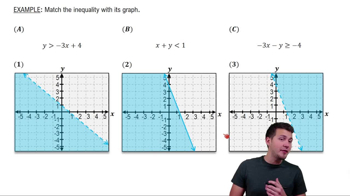
Graph the solution set of each system of inequalities.
y≥3x
y≥2
 Verified step by step guidance
Verified step by step guidance1
Step 1: Identify the inequalities in the system. The system consists of two inequalities: \( y \geq 3^x \) and \( y \geq 2 \).
Step 2: Graph the first inequality \( y \geq 3^x \). Start by graphing the boundary line \( y = 3^x \), which is an exponential function. Plot points for several values of \( x \) to get the shape of the curve, such as \( (0, 1), (1, 3), (2, 9) \), and so on. Since the inequality is \( \geq \), shade the region above the curve.
Step 3: Graph the second inequality \( y \geq 2 \). This is a horizontal line at \( y = 2 \). Since the inequality is \( \geq \), shade the region above this line.
Step 4: Determine the solution set by finding the intersection of the shaded regions from both inequalities. The solution set is where the shaded region from \( y \geq 3^x \) overlaps with the shaded region from \( y \geq 2 \).
Step 5: Clearly mark the solution set on the graph. This is the region that satisfies both inequalities, and it will be the area above both the curve \( y = 3^x \) and the line \( y = 2 \).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?

 7:2m
7:2mWatch next
Master Linear Inequalities with a bite sized video explanation from Patrick Ford
Start learning