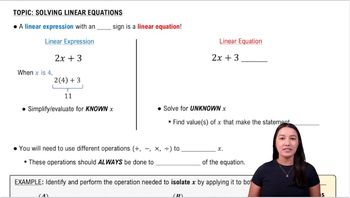
Hi. My name is Rebecca Muller. During this session, we're going to look at solutions to linear equations or to equations that end up being able to be reduced to that format. So let's look at some of the specific subtopics that we're going to consider. We want to look at a definition and basic processes for solving linear equations. We then want to look at equations requiring the multiplication of binomials. We're going to look at equations with rational expressions. And then, we'll delve into what is meant by extraneous roots. We'll look at linear inequalities also and literal equations. Now to start with, let's make sure we understand what a linear equation looks like in its most basic format. A linear equation in one variable, x, is an equation that can be written in the form ax plus b equals 0, where a is not equal to 0. If a were equal to 0, then we wouldn't have a variable in the expression. So now, let's look at our first example. We're going to consider a linear equation that is 1/3x plus 5/6 equals 1/2. Now, the first method that I'm going to use is basically the idea of moving things away from the variable expression in that I notice I can subtract 5/6 from both sides of this equation, leaving me with the 1/3x on its own. So we'll have the 1/3x. We're going to subtract 5/6 from the left hand side, and we're also going to subtract 5/6 from the right hand side. Now, this is a property of equality that tells us that as long as with an equation we're going to be subtracting the same amount from the left as we are from the right, we're still going to have an equality. At this point, what I can choose to do is to combine the two fractions by coming up with a common denominator. Now, 1/2 is the same as 3/6, so that's the way I'm going to go. Let's rewrite 1/2 as 3/6 and then minus 5/6. And now simplifying, 1/3x is going to equal 3 minus gives us negative 2. And then that's been divided by 6, and I'm going to go ahead and simplify that. Let's write it in its simplest version. That's going to be negative 1/3. Now you know, I like to think of algebra as a list of techniques that we can use in order to solve equations when it's not obvious what the solution is. But don't forget that you should really be thinking the whole time you're solving equations-- I mean, right here, if this is bothersome to you, just think, I'm just trying to take 1/3, multiply it times some expression, and end up with negative 1/3. So what is the value that I'd have to multiply positive 1/3 times in order to end up with negative 1/3? That answer is negative 1. You probably don't have to think about it as doing a step to both sides of the equation in order to make this occur. On the other hand, if I do want to write down an algebraic step, what I can think about is right now, I'm multiplying both sides of the equation by 1/3. So therefore-- sorry, not multiplying both sides but multiplying the left hand side by 1/3. So to isolate my variable, I want to divide both sides of the equation by 1/3. That's going to leave me with my x on the left hand side. On the right hand side, this is what it would look like. It would be negative 1/3 divided by positive 1/3. And because these two values are the same except for opposite signs, I can now see that the result would equal negative 1. It's normally a good idea to always check your work, and I'm going to do that in a few moments. But I want to show you an alternative method for solving the same equation before we check. The alternative method-- let's just rewrite the problem-- is that at the outset, what we can do is find the least common denominator of the three denominators that we see. We have a 3, a 6, and a 2. The least common denominator is going to be the value 6. And what I can do because it's an equation is I can multiply both sides of the equation by that value. So here's what that would look like in the second step. It would be 6 multiplied times the left hand side. That's 1/3x plus 5/6 equals 6 multiplied times the right hand side. So that would be 6 times 1/2. Now on the left hand side of the equation, I'm going to have to use distributive property. So that means I'm going to be multiplying the times the first term and then come back and multiply it times a second term, adding the two results. Here's what that looks like. We have 6 times 1/3x plus 6 times 5/6. And of course, that's equal to my 6 times 1/2. Simplifying, 6 times 1/3 is the same as 6/1 times 1/3. Or you can think of it as 1/3 of 6. Either way you look at it, the answer is 2 plus 6 times 5/6. You can think of this as 6/1. Multiply times 5/6. Notice we have a common factor of 6 that will divide out. Alternatively, you could say 6 times 5 is 30, and 30 divided by 6. Either way you look at it, the answer's 5. And on the right hand side, 6 times 1/2, well, that's just half of 6, which is going to equal 3. In the second method, notice that at the outset of the problem I'm able to multiply out the denominator so that I don't have fractions in the simplified version. Now I'm able to use a property of equality that says I can subtract 5 from both sides of the equation. So this will leave me with 2x on the left hand side, and 3 minus 5 gives me negative 2. And now, dividing both sides of the equation by positive gives me x equals negative 1. Of course, either method we work with should give us the same result. Now, I'm going to check my work. We're going to take the value of negative 1, and we're going to substitute into the original equation. When you're checking your answers, make sure you're always substituting into the original format of the equation, because if you make a mistake in some of those subsequent steps, you won't catch it necessarily if you don't substitute at the beginning. So the check looks like this. I'm going to have 1/3 multiplied times negative 1, and then I'm going to add 5/6 to it. And of course, the question is whether or not we end up with 1/2. So I'm going to concentrate on the left hand side of this equation. 1/3 times negative 1 is negative 1/3 plus 5/6. And then, I'm going to get a common denominator, which is going to be 6. And so my second term remains 5/6. My first term I can say to myself, 3 times is going to give me 6. So negative 1 times 2 is going to give me negative 2. Now, I can combine numerators since my denominators are the same, and that gives me negative 2 plus 5, which is 3. And 3 divided by 6 is 1/2. So I'm able to check that 1/2 does equal the 1/2 that we had at the outset. And so I know that my solution is correct. Now sometimes, the equations that you might see in a typical college algebra course will not necessarily start off in a simplified format, and this is one of those examples. So we're going to see that we have to use a variety of different techniques in order to solve this equation. First of all, on the left hand side, we have 5 multiplied times the quantity 3x plus 2 minus 2x multiplied times the quantity 2 plus x equals, on the right hand side, the quantity 2 plus x multiplied times the quantity 5 minus 2x. So we're going to start off by trying to-- we call it getting rid of the parentheses. That's going to require us, first of all, to use the distributive property when we're multiplying 5 times the quantity 3x plus 2. So that's going to give us 5 times 3x, which is going to be 15x, plus 5 times 2, which is equal to 10. In the second term, we have a subtraction of 2x multiplied times 2 plus x. So I'm going to think of this as multiplying negative 2x times to start with, which will give me negative 4x, and then using the distributive property, negative 2x times x, which is going to give me negative 2x squared. And you may be saying, hey, wait a minute. I got an x squared term here. Just hold on. We'll see what happens. On the right hand side, we're going to end up using a method that you might have learned if you were educated in the US. It's called the FOIL method. We have a product of two binomials. We're going to multiply the first terms together. That's going to give us 2 times 5, which is going to give us 10. That's the F in foil. The outer terms, which would be 2 times negative 2x gives us negative 4x, that's the O in FOIL. The inner terms, that would be x multiplied times 5, which is going to give us plus 5x, and that's the I in FOIL. And then the L in FOIL stands for last. So first, outer, inner, last. x multiplied times negative 2x equals negative 2x squared. So remember this negative 2x squared term that kind might have been a shock at this point-- notice that if I add 2x squared to both sides of the equation, I will not have a squared term anymore. Let's go ahead an combine other like terms. We have 15x minus 4x. That's going to give us 11x. The plus 10 will just move down to the next step, so on the left hand side of the equation, we'll end up with 11x plus 10. Because I'm going to add 2x squared to both sides of the equation. On the right hand side of the equation, we notice that we have negative 4x plus 5x that can be combined to give us x. And then, I end up having the constant plus 10. And again, the negative 2x squared was added to both sides of the equation, so it does not appear anymore. So in the second step, I've simplified to the format 11x plus 10 equals x plus 10. What we can do now is we can subtract from both sides of the equation. So I'm going to rewrite the left hand side is 11x equals the value x. And now, if we're trying to combine like terms, I can do that by subtracting x from both sides of the equation. So 11x minus x is going to give us 10x. And if I've subtracted x from the right hand side of the equation, then the result is 0. So 10 times some number equals 0. And you might be able to, by inspection, see that that result has to be 0. You can also think about it as an algebraic step where we're dividing both sides of the equation by the coefficient of our variable, x. So we're dividing both sides by to give us x equals 0, because 0 divided by 10 is 0. Now that I've got a solution, we want to check it in the original format of the equation. So I'm going to check by substituting wherever I see x. So in all of its glory, it looks like this. It's 5, parenthesis, 3 times 0 plus 2, close parenthesis, minus 2 times 0, open parenthesis, 2 plus 0. That's my left hand side of the equation. And what we're trying to figure out is whether or not it would equal the right hand side of the equation, which would be 2 plus 0 multiplied times 5 minus 2 times 0. So let's simplify the left hand side. We know that 3 times 0 is 0 plus 2. And so that's going to give us in the parentheses a value of 2. So that really simplifies to 5 times 2 minus-- notice here I have 2 times 0, which is going to give a 0. And 0 times anything is 0. So on the left hand side, I end up with 5 times 2 minus 0, which is 10. On the right hand side of the equation, we would end up with 2 plus 0, which is 2, times 5 minus 0, which is 5. And that also equals 10. So we can see that our solution does, indeed, check. Now, the cool thing about algebra is it can give us a way to answer questions in a more generalized fashion. For instance, what if you're sitting there thinking about numbers, which sometimes I do, and you say, hey, you know what? I know that 2 divided by 3 does not equal 4 divided by 5. 2/3 does not equal 4/5. But is there ever a case where I could have a scenario like this where they could be equal to each other? Well, if I notice what I'm looking at are having consecutive integers, set it up as fractional format, where if I'd let x equal 2, then x plus 1 equals 3, x plus 2 equals 4, and x plus 3 equals 5. So is it possible to ever have this situation where I could have a number divided by a number that's one more equals a number that's one more than that divided by number that's one more than that, like 2, 3, 4, 5? We can figure out that answer, and the way we can do it is to use an algebraic process. Now, the process I'm going to use here is I'm going to multiply both sides of this equation by the least common denominator. Our denominators now are variable format. But I can say that I definitely need to multiply this by x plus 1. And of course, by a property of equality, if I'm multiplying the left hand side by a value, I have to multiply the right hand side by the same value. That's going to allow me to divide out that denominator. But in order to divide out the denominator to the right, the x plus 3, I need to multiply by x plus 3. And so I need to do the same thing to the left hand side. So what happens is my least common denominator is the product of x plus 1 times x plus 3, and I'm going to multiply both sides of the equation by that product. You could have looked back and said, hey, you know what? This is a-- without the expressions on the right and left, you could have noticed this as a proportion. And there is a process you may have learned earlier in life called cross multiplying. And if that's the case, you can certainly do that, and I'll show you where that step ends up being the same in just a moment. Notice that the x plus 1 is a common factor now. We can think about this whole expression that I'm multiplying by as being over 1. It's a common factor to the numerator and the denominator, so I can divide out the x plus 1, leaving me with my numerator, x, multiplied times x plus on the left hand side of this equation. On the right hand side of the equation, the x plus 3 is a common factor. It's going to divide out, leaving me with my numerator x plus 2 multiplied times x plus 1. And so once more, if you just kind of look at that idea of cross multiplying, you're multiplying the numerator of the first times the denominator of the second, and that result is equal to the numerator of the second multiplied times the denominator of the first. The process works, of course, because of the multiplication by the least common denominator. Now in this equation, I'm going to use distributive property on the left to simplify. That will give us x times x, which is x squared plus x times 3, which is 3x. On the right hand side, I have to multiply x plus 2 times x plus 1. We're going to use the FOIL method here to multiply the two binomials. So x times x is x squared. x times 1 is x. x times 2 is 2x, and then 2 times 1 is 2. Once more, notice that the x squared in this example is going to subtract out, so I'm going to go ahead and-- notice that if I subtract x squared from the left hand side, we end up with 3x. On the right hand side, I'll subtract off the x squared, and at the same time, I notice I can add the x and the 2x to give us 3x. So the right hand side reads 3x plus 2. Well, what can we do now we'd like to get the terms that have x in them on the same side of the equation. That requires me to subtract 3x from both sides of the equation. Now, I'm going to go ahead and write in this middle step. We get 3x minus 3x equals-- on the right hand side, 3x plus 2 minus 3x is just a 2. Well, what's 3x minus 3x? That result is 0. And so at this point in solving the equation, I come up with an answer that doesn't make any sense. It's inconsistent. 0 does not equal 2. What does that mean about the original format of our equation? It means that that cannot happen either. If I come up with a result that is inconsistent, it means the equation is something called inconsistent. And therefore, there would be no solution to that equation. Now, the original question came from the idea, is there any case where I could have this setup, like 2/ equals 4/5, for some maybe very large number where it's 200/201 equals 202/203? The answer is no. So using algebra, we can say that-- this is going to be true for all across the board-- there is no situation where this could occur. Here's our next example. We have 8 divided by x squared minus 1 minus divided by x squared minus x equals 2 divided by x squared plus x. In this problem, I'm going to review factoring and look at finding the LCD after that fact. So the first step that I'm going to look at is going to be to factor each of the denominators in question. So we're going to have 8 divided by-- x squared minus 1 is a difference of two squares, so that factors as x minus 1 times x plus 1. In our second term, we have a numerator of 4, and we're going to have 4 divided by-- and then in the numerator, I notice that a common factor of x that can be factored out. So that's going to be x times x minus 1. And on the right hand side, my numerator is a 2. And I notice that in that denominator, x squared plus x, I also have a common factor of x that can be factored out, so x multiplied times x plus 1. Now, at this point in the problem, there are a couple of things that I want to do. One is I want to find the least common denominator. But another thing that I want to take note of is that I can never allow those denominators to equal 0. So what would make the first denominator equal to 0? Any time either of these factors was equal to 0, the product would be. In other words, if I allow x to equal the value of 1, this first factor would equal 0. And then, that would make the entire denominator 0. And we know that division by 0 is undefined. So I want to make note of the fact that x cannot equal the value 1. The second factor, x plus 1, would be equal to if x was equal to negative 1. So I have to make sure that I note that x cannot equal negative 1. In my second term, the denominator is factors x times x minus 1. So the x cannot equal 0 or else the denominator would be, so x cannot equal 0. I note that x cannot equal 1, but I already have that down. And my last denominator has the x again, which can not equal 0, and x plus 1, which cannot equal 0. And I already have that x cannot equal negative 1. I also want to look at finding in the least common denominator. So my least common denominator is going to end up being a product of all these denominators, but I don't want to do it-- I don't want to do overkill to be able to get the least. So I want to take the smaller-- smallest exponent that I can have of each of those. Here's a way I think about it. In my first fraction, I want to be able to multiply through, and in doing so, I want to be able to eliminate that denominator, which means I definitely need to multiply times x minus 1 and times x plus 1. In my second fraction, I need to multiply by x in order to eliminate this first factor. So I'm going to add that to my least common denominator. I notice I also need to multiply by x minus 1, but I already have that here. In my last fraction, my denominator is x times x plus 1. Well the x I already took care of in my LCD. The x plus 1 I also already have in my LCD. So my least common denominator is the product of x times x minus 1 times x plus 1. Now, what's going to occur in this problem is we're going to come in here, and we're going to multiply both sides of this equation by that least common denominator. I'm gonna go ahead and write it in here. I'm going to have x multiplied times x minus 1 multiplied times x plus 1. That's going to be multiplied times everything on the left hand side of the equation. And then, we're going to do the same thing on the right hand side of the equation, x times x minus 1 times x plus 1. Now, the beauty in doing this step is that in my next step, I should not have any fractions left in the equation, and it should look a lot simpler. Let's look at doing this using distributive property on the left hand side. When I multiply my LCD times my first term, we're looking at what's going to cancel and what's going to be left over. So the x minus 1 will cancel. The x plus 1 will cancel. I'll be left with a value x, and that needs to be multiplied times the numerator 8. So what I end up with is 8x. Then, I have minus, and now let's see what happens when we look at multiplying my LCD times a second term. The x words will divide out. The x minus 1 will divide out, but I'll be left with my numerator, 4, being multiplied times what did not divide out, which is the x plus 1. On the right hand side, the x will divide out. The x plus 1 will divide out. The numerator, 2, will be multiplied times x minus 1. And all of a sudden, a fairly complicated looking example for an equation ends up being something that looks pretty reasonable to work with. I'm now going to be able to simplify this. We can use distributive property. We'll we have 8x minus 4x minus 4 on the left equals 2x minus on the right. Let's go ahead and combine like terms. On the left, that's going to give us 8x minus 4x, which is 4x. And then we have minus 4 equals 2x minus 2. I'm now going to use properties of equality to allow me to put my terms together that have the variable in them and the constant terms together. And I can do that by subtracting 2x from both sides of the equation. So 4x minus 2x gives me 2x. At the same time, I'm gonna add to both sides of the equation. So I'm gonna end up with that 2x equals-- the 2x would have subtracted out. I have negative 2 plus 4, which is going to end up being a positive 2. Dividing both sides by 2 gives us x equals 1. And after all these steps, you're like, shew, I'm done. But remember. At the outset of the problem, we had to note that x could not equal 1, because the value of would have made a denominator equal to 0. So because this is the only solution I come up with and it turns out it can't be a solution, then I have to say there is no solution to this problem. So why did this happen? I did everything right. Well, notice that I start off with a problem that has restrictions on what the variable can be. It has fractions in it. I have to make note of those restrictions. But then, I change the problem to an equation that does not have restrictions on it. Because there are no fractions in this equation, once I've multiplied through by the least common denominator, then I've like lost the restriction. So x equal to 1 does solve the equation where there are no restrictions. The problem is that I've changed it from the format that it had originally. That's why you always want to take a solution and check it in the original version of the problem, not in some subsequent step. So when we end up with a solution that actually cannot work in our original equation, what we say is that we've ended up with something called an extraneous root. Just means kind of like something extra that just doesn't fit-- extraneous. It's time for a quick quiz. An blank route is a solution to an intermediate step of an equation. But not a solution to its original version due to restrictions on the domain. Is the correct answer. A. An absolute route. B. An extraneous route. Or C. An intermittent route. Choose nail from A, B, or C. You're right the correct answer is an extraneous route. Sorry the correct answer is B. an extraneous route. An extraneous route occurs when you've worked the problem correctly but it turns out there were restrictions on the original version of the problem. This is why it's important to go back and check your answer inequations. This occurs for many cases in equations that have fractional values that have a variable in the denominator or a value that might be under a square root where there is restrictions on the domain. An inequality is a mathematical statement that uses one of the following symbols. It could be a greater than symbol, a greater than or equal to symbol, a less than symbol, or a less than or equal to symbol. So let's look at something that we know is true. If we have negative 4-- or, let's say positive 4-- let's go with that first-- is greater than 3. We know that statement's true. What happens if I multiply both sides of this inequality statement by the value negative 1? So we're going to have negative 1 multiplied times 4. And then what we're interested is what's going to happen to that inequality symbol. And we're going to have on the right hand side multiplied times negative 1. So again, negative 1 times 4, and we're questioning what the relationship will be when we look at 3 times negative 1. So negative 1 times 4 we know is negative 4. And again, we're trying to figure out what that has to do with the product 3 times negative 1, which is negative 3. Well, is negative 4 larger than negative or smaller than negative 3? Thinking about it on a number line, we know that negative lies to the left of negative 3 indicating that negative is actually smaller than negative 3. So looking back at the fact that we had-- at the outset of this inequality that 4 was greater than 3, when we multiplied through by a negative value, notice that the inequality symbol switches its direction so that the greater than became a less than. In general, when an inequality is multiplied or divided by a negative number, the direction of the inequality symbol is reversed. So let's solve a couple of linear inequalities next. The first one I want to look at is 3 minus 4x is less than or equal to the value of 1. So when we're solving linear inequalities, we're going to use the same process we used when we were solving equations with the exception of that one time where we multiply or divide by negative values. Here, I'll start the problem by subtracting from both sides of the inequality. So on the left hand side, I'm left with negative 4x. On the right hand side, 1 minus 3 gives us negative 2. Now, my next step is to divide both sides of the inequality by negative 4. Again, remember that it's in this step where the inequality symbol has to be reversed. So dividing on the left hand side by negative is going to give us negative 4x divided by negative 4, which leaves us with x. Because of the division by a negative number, the inequality symbol which used to read less than or equal to now is going to read greater than or equal to. On the right hand side, I'm taking negative and dividing by negative 4. That's going to end up giving me a positive 1/2. So what we're able to see is that as long as I choose a number larger than or equal to 1/2, I will end up having an inequality that's going to be correct in its original format. So for instance, if you choose the number 1, 3 minus 4 times 1, we know that-- we're questioning whether or not that's less than or equal to 1. Well, 3 minus 4 is going to be negative 1. Is that going to be less than or equal to 1? And of course, it is. So we found a solution to that. The next thing to consider is a second example, and this one's a little bit different. It's going to have 1/2 is less than 5 minus 2x, and that's less than or equal to the value of 7. This is really two inequalities put together. One of them is the fact that you have 1/ less than 5 minus 2x, and the other one is that 5 minus 2 x has to be simultaneously less than or equal to 7. I sometimes call this a three-part inequality because of the fact that what I'm going to do to solve this inequality is I'm going to just think about doing the same thing to all three parts of the inequality as I work through it. So one thing I notice is that I have this 1/2 over here. And one thing that's nice, as we learned in linear equations, would be that if I can multiply through by the least common denominator, then I can rid my equation of fractions. And I'm going to choose to use that method. So I'm going to multiply by 2. But recall, that means I'm going to multiply 2 times 1/2. I'm also going to multiply 2 times the middle section, which means I'm multiplying 2 times 5 minus 2x. And then, I'm also multiplying times the right hand side of that inequality, and that's 2 times 7. So simplifying, I end up with 2 times 1/2, which is 1, less than-- in the middle section, I'll use distributive property-- 2 times 5 is 10 and then minus 2 times 2, which is 4x. And that's less than or equal to 14. Now, again, let's just think about how we're going to proceed. And the thing is we're trying to isolate our variable expression. So now, if you just think about what you would do if it didn't have all three parts, you can figure out what your next step would be. To isolate the negative 4x, I'm going to subtract 10 from all three parts of this inequality. So subtracting 10 is going to end up giving me 1 minus 10, which is negative 9, less than-- in the middle when I subtract 10, I'll only have my negative 4x, my variable expression left. And on the right hand side, I'll be subtracting to give us less than or equal to 14 minus 10, which is 4. To isolate x further, we want to end up dividing everything by the value negative 4. And division by a negative number inside of a vote inequality means that we're going to have to switch all of our inequality symbols. So let's do that now. Negative 9 divided by negative 4 is going to be 9/4. The inequality symbol is going to switch from being less than to being greater than. Negative 4x divided by negative 4 is x. And then the inequality symbol will switch from being less than or equal to or greater than or equal to. And 4 divided by negative 4 is going to be negative 1. Now, I can rewrite this in the order from less to greater. That is, I can write negative 1 is less than or equal to x is less than 9/4 simply by thinking about the fact that, for instance, if 9/4 is bigger than x, then x has to be smaller than 9/4. So you can see that as you switch sides, you're going to be changing all of the-- you're basically a rotation of everything. And so this means as long as I choose a value that is between negative 1 and 9/4 and maybe equal to negative 1, then in the original format of the problem, 5 minus 2x will end up being between 1/2 and 7 and maybe including 7. Quick quiz. Blank by a negative value on both sides of an inequality requires a reversal in the direction of the inequality symbol. Should the blank be filled in with Addition. Subtraction. Or multiplication. The correct answer is C. Multiplication. Multiplication by a negative value on both sides of an inequality requires a reversal in the direction of the inequality symbol. Sorry the correct answer is C. Multiplication. Multiplication by a negative value on both sides of an inequality requires a reversal in the direction of the inequality symbol. Now, we're going to look at an extension of what we've been working with with linear equations into something called literal equations. You can kind of think of literal equations like a formula. And here's a formula that we have from resistance. It's 1 divided by r sub 1 plus 1 divided by r sub equals 1 divided by r. So if you're in a physical science or a physics class, you may come across this formula. Now, here, I don't really know what I'm looking for until I'm being told, and the thing that I would like to solve for here is we'd like to solve for one of these variables. And I'm going to choose to solve for r sub 2. One thing about this formula and reason I wanted to use it is it has subscripts. You have to understand that the r sub 1 is different than the r sub 2 is different than the r. Of course, in the formula, it made sense, because it had to do with resistance in each case. If you had resistance of one wire resistance of the next one equals resistance of the total system, then you were going to be able to look at what was going on in the physical sense. Here, mathematically, all I really want to do is see the process that we're going to use in order to solve for that value of r sub 2. I'm going to use some of the same processes that we saw earlier in that since I have fractions here, one thing I can choose to do is to find the least common denominator and multiply both sides of the equation by that value. In order to able to cancel out or divide out the denominator in the first term, I need to be multiplying times r sub 1. In order to divide out the denominator in the second term, I need to multiply times r sub 2. And remember, these are not the same thing. And in the third term, I need to multiply by r. So I'm going to choose to multiply both sides of the equation by this LCD. Let's write it in. We're going to have r multiplied-- r sub 1-- I'll go ahead and put it first-- times r sub 2 times r. It's going to be multiplied times 1 over r sub plus 1 over r sub 2 on the left hand side of the equation. And on the right hand side of the equation, the 1 over r will be multiplied times the same product. The whole idea of multiplying through by these denominators-- by this LCD, that is-- is to end up with no denominators in the next step. On the left hand side of the equation, we need to use distributive property. The LCD multiplied times the first term, the r sub will divide out, but I'll be left with my numerator 1 multiplied times the other two factors. That is, I'll end up with r sub 2 multiplies times r when I multiply the first term. Plus-- in the second term, the r sub will cancel out, leaving me with my numerator 1 multiplied times r sub 1 times r. And on the right hand side of the equation, the r will divide out, leaving me with r sub 1 times r sub 2. Now remember, we were told we would like to solve for r sub 2. Notice there are two terms that have r sub 2 in them. What I'm going to want to do is get those two terms on the same side of the equation. So I can accomplish that by subtracting r sub 2 times r from both sides of the equation. Let's rewrite that now. It's going to be r sub 1 times r left on the left, and that equals r sub 1 times r sub 2, which is the term I had on the right originally, minus r sub 2 times r, which has now been translated over to the right. Now that I have the two terms that have the variable in question by themselves, I notice that it is a common factor in those two terms. So my next step is to rewrite this as r sub 1 times r equals r sub 2 times r sub 1, which is left over when I factor it out, minus r, which is left over when I factor it out from the second term. At this point, because I'm multiplying r sub 2 times the quantity r sub 1 minus r, I'm going to divide both sides of the equation by what basically is its coefficient. So I'm being left with r sub 2 on the right hand side. On the left hand side, I'll have r sub 1 times r divided by r sub 1 minus r. And there are no common factors here. You cannot simplify that any further. So in the formula that we've started with, notice that when we solve for the variable r sub 2, we need to ensure that everything on the other side of the equation has other variables in it, not r sub 2. You cannot solve r sub 2 in terms of itself, which is why, for instance, in this step right here at the bottom, I decided-- when I had r sub 1 times r sub 2 by itself, I did not choose to divide both sides of the equation by r sub 1. Because the other side of the equation also had the term r sub 2-- or the variable r sub 2 in that first term. So once more, when you're solving a literal equation at the end, double check. Have you solved for the variable that was in question? The answer here is yes. And what I've solved for cannot include the same variable in that expression. Now, here's a literal equation for you to try. We want to solve for t in the formula A equals P times the quantity 1 plus r times t. Try this problem on your own, and then we'll come back and work it together. OK, let's see how you did. There are really one of two methods I can think of that you might have chosen to use. So I'm going to do both of those. The first method is maybe you decided to use distributive property to start with. So if that's the method you used-- let's just call this method one-- you would rewrite in the first step A equals P times 1, which is P, plus P times r times t. Now, since we're trying to solve for the variable t, I want to leave that last term by itself, and I can accomplish that by subtracting P from both sides of the equation. So A minus P equals P times r times t. Now, t is being multiplied times P times r. So I'm going to divide both sides of the equation by that result. And I'll go ahead and just write it in here, and what you're doing is dividing out the P times r on both sides of the equation. On the left hand side, we're left with A minus P divided by P times r. On the right hand side, the Pr divides out, and you end up left with the variable that was what we were trying to solve for, which is t. So this is one method you may have chosen to use. Another method-- let's just go ahead and call this method two. And I'm going to go ahead and rewrite the original version of the equation. Perhaps at the outset, you notice that t is inside the parentheses in the term rt, and it's added to 1. But the P that's outside the parentheses could be divided on both sides of the equation. So if you chose that route, then your second step looks like this, A divided by P equals 1 plus r times t. Since the rt is in the last term, I can subtract 1 from both sides of the equation next, so I end up with A divided by P minus 1 equals r times t. Now at this point in the problem, you need to divide by r in order to isolate the value of t. And there's two ways to write this, but I'm going write it in what I think would be probably the most common way, which is just to take the left hand side of the equation and write A divided by P minus all divided by R, and that's going to equal the value t. I wanted to go this method because what you end up with right now is called a complex fraction, and that's typically not considered a good format to leave your answer in. So what is the process we can use by which we can simplify a complex fraction? The reason it's complex is because I have a fraction inside of a fraction. And if I look at the denominator of the fraction that's inside the fraction, it's a denominator of P. I can choose to multiply both the numerator and denominator of this expression by P, because what I'm really doing is multiplying times the value 1, which does not change its value. In the numerator, I have to think about the fact that there are two terms there, so we have to distribute the multiplication by P. P times A/P gives me a-- minus-- P times 1 gives me P. And in the denominator, I have to multiply the P value times r, so that's P times r. And that equals t. And of course, we can see that we come up with the same result regardless of the method that we choose to use. I hope you were able to find this solution in the format that I've been able to give it to you. And I hope that you have fun on your own trying some more work with linear equations, linear inequalities, and literal equations. Good luck.
Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
Linear Equations
Video duration:
6mPlay a video:
Related Videos
Related Practice