Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
5. Rational Functions
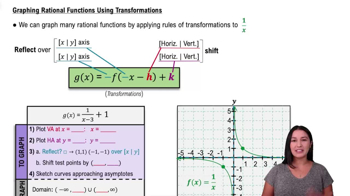
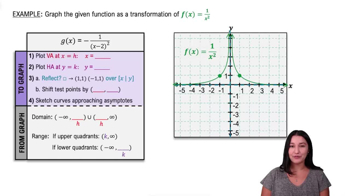
Graphing Rational Functions
Problem 58b
Textbook Question
Identify any vertical, horizontal, or oblique asymptotes in the graph of y=f(x). State the domain of f.
<IMAGE>
 Verified step by step guidance
Verified step by step guidance1
To identify vertical asymptotes, look for values of x that make the denominator of the function zero, as these are points where the function is undefined. Solve the equation set by the denominator equal to zero.
For horizontal asymptotes, compare the degrees of the numerator and the denominator. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is y = 0. If they are equal, the horizontal asymptote is the ratio of the leading coefficients.
If the degree of the numerator is exactly one more than the degree of the denominator, there is an oblique (or slant) asymptote. Perform polynomial long division to find the equation of the oblique asymptote.
To determine the domain of the function, identify all x-values that make the denominator zero, as these are excluded from the domain. The domain is all real numbers except these values.
Summarize the findings: list the vertical asymptotes, horizontal or oblique asymptotes, and state the domain of the function based on the excluded x-values.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?

 5:31m
5:31mWatch next
Master Graphing Rational Functions Using Transformations with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice