Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
Two Variable Systems of Linear Equations
Problem 41b
Textbook Question
Textbook QuestionDetermine the system of equations illustrated in each graph. Write equations in standard form. 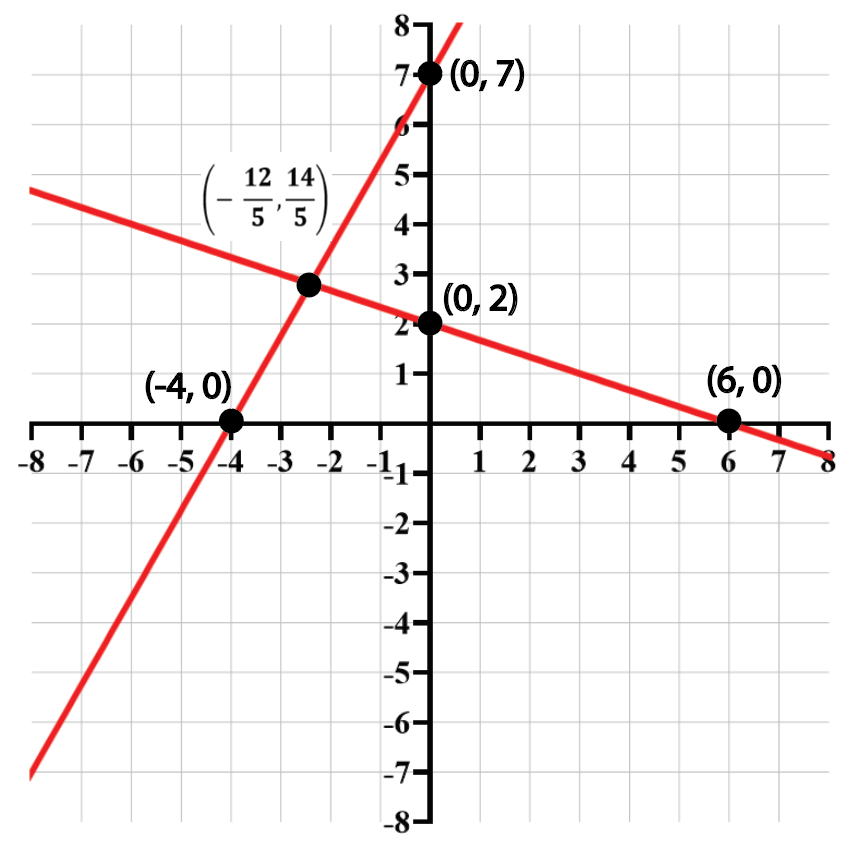
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Linear Equations
Linear equations are mathematical statements that describe a straight line when graphed on a coordinate plane. They can be expressed in various forms, including slope-intercept form (y = mx + b) and standard form (Ax + By = C). Understanding how to derive these equations from a graph is essential for solving problems involving linear relationships.
Recommended video:

Categorizing Linear Equations
Standard Form of a Linear Equation
The standard form of a linear equation is typically written as Ax + By = C, where A, B, and C are integers, and A should be non-negative. This form is useful for quickly identifying intercepts and is often required in problems involving systems of equations. Converting from slope-intercept or point-slope forms to standard form is a common task in algebra.
Recommended video:
Guided course
Standard Form of Line Equations
Graphing Linear Equations
Graphing linear equations involves plotting points that satisfy the equation on a coordinate plane. Key points include the x-intercept (where the line crosses the x-axis) and the y-intercept (where it crosses the y-axis). Understanding how to identify these points from a graph is crucial for writing the corresponding equations and analyzing the relationships between variables.
Recommended video:

Categorizing Linear Equations

 4:27m
4:27mWatch next
Master Introduction to Systems of Linear Equations with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice













