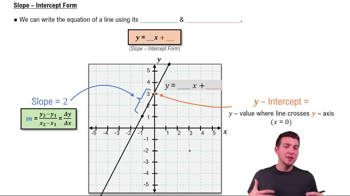
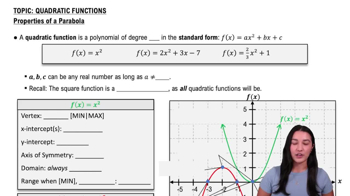
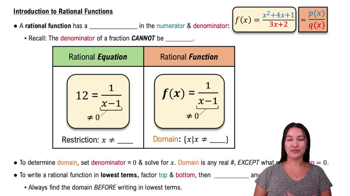
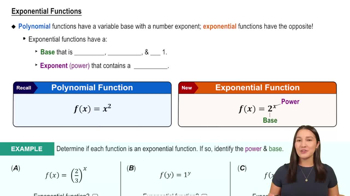
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
Yeast growth Consider a colony of yeast cells that has the shape of a cylinder. As the number of yeast cells increases, the cross-sectional area A (in mm²) of the colony increases but the height of the colony remains constant. If the colony starts from a single cell, the number of yeast cells (in millions) is approximated by the linear function N(A) - CₛA, where the constant Cₛ is known as the cell-surface coefficient. Use the given information to determine the cell-surface coefficient for each of the following colonies of yeast cells, and find the number of yeast cells in the colony when the cross-sectional area A reaches 150 mm². (Source: Letters in Applied Microbiology, 594, 59, 2014)
The scientific name of baker’s or brewer’s yeast (used in making bread, wine, and beer) is Saccharomyces cerevisiae. When the cross-sectional area of a colony of this yeast reaches 100 mm², there are 571 million yeast cells.
 Verified Solution
Verified Solution