Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
0. Functions
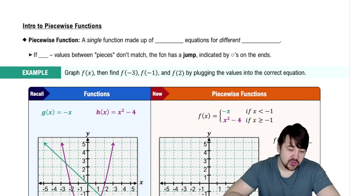
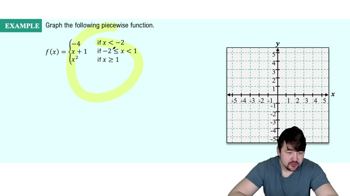
Piecewise Functions
Problem 1.33
Textbook Question
Graph the following functions.
This page contains the following errors:
error on line 1 at column 295: Unescaped '<' not allowed in attributes values
Below is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 295: Unescaped '<' not allowed in attributes values
Below is a rendering of the page up to the first error.
 Verified step by step guidance
Verified step by step guidance1
Identify the function that needs to be graphed. If the function is not explicitly given, ensure that you have the correct expression or equation to work with.
Determine the domain of the function. This involves identifying all the possible input values (x-values) for which the function is defined.
Find the critical points of the function by taking its derivative and setting it equal to zero. Solve for x to find the points where the function's slope is zero, indicating potential maxima, minima, or points of inflection.
Evaluate the function at the critical points and endpoints of the domain to determine the corresponding y-values. This will help in understanding the behavior of the function at these key points.
Sketch the graph by plotting the critical points and any intercepts, and then draw the curve based on the behavior of the function as determined by its derivative and second derivative (if necessary). Consider asymptotic behavior and continuity to ensure an accurate representation.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Related Videos
Related Practice