Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
Introduction to Trigonometric Functions
Problem 68
Textbook Question
In Exercises 65–68, ABC is a right triangle with the right angle at C. The sides opposite angles A, B, and C are a, b, and c, respectively.
a. Express sin A in terms of a and c.
b. Express sin A in terms of b and c.
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the problem setup. We have a right triangle ABC with the right angle at C. The sides opposite angles A, B, and C are labeled as a, b, and c, respectively. In a right triangle, the side opposite the right angle is the hypotenuse, which is c in this case.
Step 2: Recall the definition of the sine function in a right triangle. The sine of an angle is defined as the ratio of the length of the side opposite the angle to the length of the hypotenuse.
Step 3: To express sin A in terms of a and c, use the definition of sine. Since angle A is opposite side a and the hypotenuse is c, we have: <math xmlns='http://www.w3.org/1998/Math/MathML'><mrow><mi>sin</mi><mo> </mo><mi>A</mi><mo>=</mo><mfrac><mi>a</mi><mi>c</mi></mfrac></mrow></math>
Step 4: To express sin A in terms of b and c, we need to use the Pythagorean identity. In a right triangle, the square of the hypotenuse (c) is equal to the sum of the squares of the other two sides (a and b). Therefore, <math xmlns='http://www.w3.org/1998/Math/MathML'><mrow><msup><mi>c</mi><mn>2</mn></msup><mo>=</mo><msup><mi>a</mi><mn>2</mn></msup><mo>+</mo><msup><mi>b</mi><mn>2</mn></msup></mrow></math>. Solve for a: <math xmlns='http://www.w3.org/1998/Math/MathML'><mrow><mi>a</mi><mo>=</mo><msqrt><mrow><msup><mi>c</mi><mn>2</mn></msup><mo>-</mo><msup><mi>b</mi><mn>2</mn></msup></mrow></msqrt></mrow></math>
Step 5: Substitute the expression for a from Step 4 into the sine formula from Step 3 to express sin A in terms of b and c: <math xmlns='http://www.w3.org/1998/Math/MathML'><mrow><mi>sin</mi><mo> </mo><mi>A</mi><mo>=</mo><mfrac><msqrt><mrow><msup><mi>c</mi><mn>2</mn></msup><mo>-</mo><msup><mi>b</mi><mn>2</mn></msup></mrow></msqrt><mi>c</mi></mfrac></mrow></math>
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Sine Function
The sine function is a fundamental trigonometric function defined for a right triangle as the ratio of the length of the side opposite an angle to the length of the hypotenuse. For angle A in triangle ABC, sin A = opposite/hypotenuse = a/c, where 'a' is the side opposite angle A and 'c' is the hypotenuse.
Recommended video:

Graph of Sine and Cosine Function
Right Triangle Properties
In a right triangle, the relationship between the angles and sides is governed by the Pythagorean theorem and trigonometric ratios. The right angle (90 degrees) allows for the use of sine, cosine, and tangent to relate the angles to the lengths of the sides, which is essential for solving problems involving right triangles.
Recommended video:

Properties of Functions
Trigonometric Ratios
Trigonometric ratios are the ratios of the lengths of sides of a right triangle relative to its angles. These ratios (sine, cosine, tangent) are used to express relationships between the angles and sides, allowing for the calculation of unknown lengths or angles in triangle ABC, particularly in expressing sin A in terms of different sides.
Recommended video:
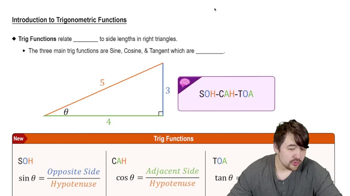
Introduction to Trigonometric Functions

 5:4m
5:4mWatch next
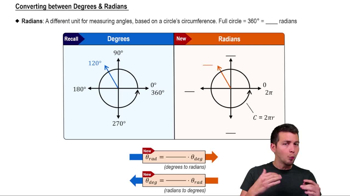
Master Converting between Degrees & Radians with a bite sized video explanation from Patrick Ford
Start learning