Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
4. Applications of Derivatives
Linearization
Problem 39
Textbook Question
Use linear approximations to estimate the following quantities. Choose a value of a to produce a small error.
√146
 Verified step by step guidance
Verified step by step guidance1
Identify the function you want to approximate. In this case, it's the square root function, f(x) = √x.
Choose a value of 'a' that is close to 146 and easy to compute. A good choice is a perfect square near 146, such as 144, because √144 = 12.
Find the derivative of the function f(x) = √x. The derivative is f'(x) = 1/(2√x).
Use the linear approximation formula: L(x) = f(a) + f'(a)(x - a). Substitute a = 144, f(144) = 12, and f'(144) = 1/(2√144) = 1/24.
Calculate L(146) using the formula: L(146) = 12 + (1/24)(146 - 144). This will give you an estimate for √146.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Linear Approximation
Linear approximation is a method used to estimate the value of a function near a given point using the tangent line at that point. It is based on the idea that a function can be closely approximated by a linear function when the input is near a specific value. The formula for linear approximation is f(a) + f'(a)(x - a), where f(a) is the function value at point a, and f'(a) is the derivative at that point.
Recommended video:
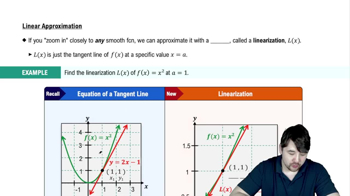
Linearization
Derivative
The derivative of a function at a point measures the rate at which the function's value changes as its input changes. It is a fundamental concept in calculus that provides information about the slope of the tangent line to the function at that point. For the function f(x) = √x, the derivative can be calculated using the power rule, which helps in determining the linear approximation.
Recommended video:

Derivatives
Choosing a Value for a
Selecting an appropriate value for 'a' is crucial in linear approximation to minimize error. Ideally, 'a' should be a value close to the point of interest (in this case, 146) where the function is easy to compute. For estimating √146, a nearby perfect square, such as 144 (where √144 = 12), can be chosen to ensure that the linear approximation yields a small error.
Recommended video:

Average Value of a Function
Related Videos
Related Practice



