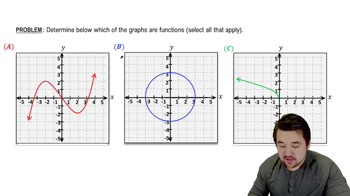
Decide whether $f$, $g$, or both represent one-to-one functions.​​ <IMAGE>

Verified step by step guidance

1. Understand the definition of a one-to-one function: A function is one-to-one if and only if every element of the function's range is mapped to by exactly one element of its domain. This means that no two different inputs should produce the same output.
2. To determine if a function is one-to-one, you can use the Horizontal Line Test. If any horizontal line intersects the graph of the function more than once, the function is not one-to-one.
3. Examine the graph of function f. Check if there are any horizontal lines that intersect the graph at more than one point. If such lines exist, f is not one-to-one.
4. Examine the graph of function g. Similarly, check for horizontal lines that intersect the graph at more than one point. If such lines exist, g is not one-to-one.
5. Conclude based on your observations: If neither graph is intersected more than once by any horizontal line, both functions are one-to-one. If only one graph passes the test, only that function is one-to-one. If both fail, neither is one-to-one.