Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
0. Functions
Graphs of Trigonometric Functions
Problem 66
Textbook Question
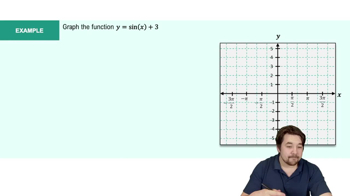
Find a trigonometric function f represented by the graph in the figure. <IMAGE>
 Verified step by step guidance
Verified step by step guidance1
Identify the key features of the trigonometric graph, such as amplitude, period, phase shift, and vertical shift. These features will help determine the specific trigonometric function.
Determine the amplitude by measuring the vertical distance from the midline of the graph to a peak or trough. The amplitude is the absolute value of this distance.
Calculate the period of the function by identifying the horizontal length of one complete cycle of the graph. This can be done by measuring the distance between two consecutive peaks or troughs.
Check for any phase shift by observing if the graph is shifted horizontally from the standard position of a basic trigonometric function like sine or cosine.
Look for any vertical shift by determining if the midline of the graph is above or below the x-axis. This will indicate if the function has been shifted up or down.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
7mPlay a video:
Was this helpful?

 5:53m
5:53mWatch next
Master Graph of Sine and Cosine Function with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice