Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
Applied Optimization
Problem 49e
Textbook Question
Travel costs A simple model for travel costs involves the cost of gasoline and the cost of a driver. Specifically, assume gasoline costs $p/gallon and the vehicle gets g miles per gallon. Also assume the driver earns $w/hour.
e. Should the optimal speed be increased or decreased (compared with part (d)) if L is increased from 400 mi to 500 mi? Explain.
 Verified step by step guidance
Verified step by step guidance1
First, understand that the total travel cost is composed of two main components: the cost of gasoline and the cost of the driver. The cost of gasoline depends on the price per gallon \( p \) and the vehicle's fuel efficiency \( g \) in miles per gallon. The cost of the driver depends on the wage \( w \) per hour and the time taken to travel the distance \( L \).
The time taken to travel a distance \( L \) at speed \( v \) is given by \( \frac{L}{v} \) hours. Therefore, the cost of the driver is \( w \times \frac{L}{v} \).
The amount of gasoline needed to travel \( L \) miles is \( \frac{L}{g} \) gallons. Thus, the cost of gasoline is \( p \times \frac{L}{g} \).
The total cost \( C \) as a function of speed \( v \) is given by the sum of the gasoline cost and the driver cost: \( C(v) = p \times \frac{L}{g} + w \times \frac{L}{v} \).
To determine whether the optimal speed should be increased or decreased when \( L \) is increased from 400 mi to 500 mi, consider how each component of the cost function \( C(v) \) changes with \( L \). Analyze the derivative of \( C(v) \) with respect to \( v \) to find the optimal speed and observe how it is affected by changes in \( L \).
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Cost Function
A cost function in this context represents the total expenses incurred during travel, which includes both gasoline costs and driver wages. It can be expressed as a function of distance traveled, speed, and fuel efficiency, allowing for the analysis of how changes in these variables affect overall travel costs.
Recommended video:

Properties of Functions
Marginal Cost
Marginal cost refers to the additional cost incurred by increasing the distance traveled or changing the speed. Understanding marginal costs is crucial for determining the optimal speed, as it helps identify the point where the cost of additional travel equals the benefits gained from reaching the destination faster.
Recommended video:

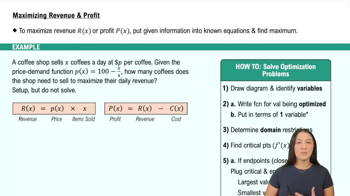
Example 3: Maximizing Profit
Optimal Speed
Optimal speed is the speed at which travel costs are minimized for a given distance. It is influenced by factors such as fuel efficiency, time costs associated with the driver's wage, and the distance to be traveled. Analyzing how changes in distance (like from 400 mi to 500 mi) affect optimal speed is essential for making cost-effective travel decisions.
Recommended video:

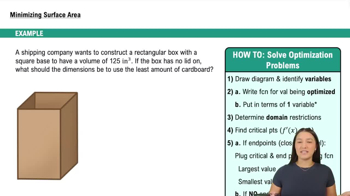
Intro to Applied Optimization: Maximizing Area

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice